42.136+272.15+28.68.2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: \(\left(x-2\right)\left(3x-1\right)\left(x^2-4x+1\right)\)
\(=\left(3x^2-x-6x+2\right)\left(x^2-4x+1\right)\)
\(=\left(3x^2-7x+2\right)\left(x^2-4x+1\right)\)
\(=3x^4-12x^3+3x^2-7x^3+28x^2-7x+2x^2-8x+2\)
\(=3x^4-19x^3+33x^2-15x+2\)
b: \(x\left(3-4x\right)\left(2x^2-3x\right)\)
\(=\left(-4x^2+3x\right)\left(2x^2-3x\right)\)
\(=-8x^4+12x^3+6x^3-9x^2\)
\(=-8x^4+18x^3-9x^2\)
a)
\(\left(x-2\right)\left(3x-1\right)\left(x^2-4x+1\right)\\ =\left(3x^2-6x-x+2\right)\left(x^2-4x+1\right)\\ =\left(3x^2-7x+2\right)\left(x^2-4x+1\right)\\ =3x^4-12x^3+3x^2-7x^3+28x^2-7x-8x+2\\ =3x^4-19x^3+31x^2-15x+2\)
b)
\(x\left(3-4x\right)\left(2x^2-3x\right)\\ =\left(3x-4x^2\right)\left(2x^2-3x\right)\\ =6x^3-9x^2-8x^4+12x^3\\ =-8x^4+18x^3-9x^2\)

\(x^7+x^2+1\)
\(=x^7+x^6+x^5-x^6-x^5-x^4+x^4+x^3+x^2-x^3-x^2-x+x^2+x+1\)
\(=x^5\left(x^2+x+1\right)-x^4\left(x^2+x+1\right)+x^2\left(x^2+x+1\right)-x\left(x^2+x+1\right)+\left(x^2+x+1\right)\)
\(=\left(x^2+x+1\right)\left(x^5-x^4+x^2-x+1\right)\)

Bài 1: Chiều dài mảnh đất là 132:4=33(m)
Chu vi miếng đất là \(\left(33+4\right)\times2=74\left(m\right)\)
Bài 1:
Chiều dài mảnh đất là:
\(132:4=33\left(m\right)\)
Chu vi mảnh đất là:
\(\left(33+4\right)\times2=74\left(m\right)\)
Đáp số: 72 m
Bài 2:
Đổi \(14m5dm=145dm;2m=20dm\)
Chu vi mảnh vườn là:
\(145\times4=580\left(dm\right)\)
Độ dài hàng rào là:
\(580-2=578\left(dm\right)\)
Đáp số: 578 dm

a)
\(32< 2^x< 128\\ =>2^5< 2^x< 2^7\\ =>5< x< 7\\ =>x=6\)
b)
\(2\cdot16\ge2^x>4\\ =>2\cdot2^4\ge2^x>2^2\\ =>2^5\ge2^x>2^2\\ =>5\ge x>2\\ =>x\in\left\{3;4;5\right\}\)
c)
\(9\cdot27\le3^x\le243\\ =>3^2\cdot3^3\le3^x\le3^5\\ =>3^5\le3^x\le3^5\\ =>5\le x\le5\\ =>x=5\)
d)
\(x^{2019}=x\\ =>x^{2019}-x=0\\ =>x\left(x^{2018}-1\right)=0\)
TH1: x = 0
TH2: `x^2018-1=0`
`=>x^2018=1`
`=>x^2018=1^2018`
`=>x=1` hoặc `x=-1`
a: \(32< 2^x< 128\)
=>\(2^5< 2^x< 2^7\)
=>5<x<7
mà x là số tự nhiên
nên x=6
b: \(2\cdot16>=2^x>4\)
=>\(2^5>=2^x>2^2\)
=>2<x<=5
mà x là số tự nhiên
nên \(x\in\left\{3;4;5\right\}\)
c: \(9\cdot27< =3^x< =243\)
=>\(243< =3^x< =243\)
=>\(3^x=243=3^5\)
=>x=5
d: \(x^{2019}=x\)
=>\(x\left(x^{2018}-1\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x^{2018}-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x^{2018}=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
e: \(2^{x+1}+4\cdot2^x=3\cdot2^7\)
=>\(2^x\cdot2+4\cdot2^x=6\cdot2^6\)
=>\(6\cdot2^x=6\cdot2^6\)
=>x=6
f: \(2^{2x}+2^{2x+3}=3^2\cdot8^4\)
=>\(2^{2x}+2^{2x}\cdot8=9\cdot8^4\)
=>\(9\cdot2^{2x}=9\cdot2^{12}\)
=>2x=12
=>x=6
g: \(27^{x+1}=9^{x+5}\)
=>\(3^{3\left(x+1\right)}=3^{2\left(x+5\right)}\)
=>3(x+1)=2(x+5)
=>3x+3=2x+10
=>3x-2x=10-3
=>x=7
h: \(3^{x+2}+5\cdot3^{x+1}=648\)
=>\(3^x\cdot9+5\cdot3^x\cdot3=648\)
=>\(3^x\cdot24=648\)
=>\(3^x=\dfrac{648}{24}=27=3^3\)
=>x=3

a) Thay x=2 vào ta có:
\(2^2-4m\cdot2+1=0\\ \Leftrightarrow4-8m+1=0\\ \Leftrightarrow5-8m=0\\ \Leftrightarrow8m=5\\ \Leftrightarrow m=\dfrac{5}{8}\)
b) Thay x=2 vào ta có:
\(3\cdot2^2-5m\cdot2+7\\ \Leftrightarrow12-10m+7=0\\ \Leftrightarrow19-10m=0\\ \Leftrightarrow10m=19\\\Leftrightarrow m=\dfrac{19}{10}\)
a:
Đặt \(x^2-4mx+1=0\left(1\right)\)
Thay x=2 vào (1), ta được:
\(2^2-4m\cdot2+1=0\)
=>\(4-8m+1=0\)
=>5-8m=0
=>8m=5
=>\(m=\dfrac{5}{8}\)
b: Đặt \(3x^2-5mx+7=0\left(2\right)\)
Thay x=2 vào (2), ta được:
\(3\cdot2^2-5m\cdot2+7=0\)
=>12-10m+7=0
=>19-10m=0
=>10m=19
=>\(m=\dfrac{19}{10}\)

ĐKXĐ: \(x\notin\left\{0;-20\right\}\)
\(\dfrac{100}{x}-\dfrac{100}{x+20}=\dfrac{5}{12}\)
=>\(\dfrac{20}{x}-\dfrac{20}{x+20}=\dfrac{1}{12}\)
=>\(\dfrac{20\left(x+20\right)-20x}{x\left(x+20\right)}=\dfrac{1}{12}\)
=>\(\dfrac{400}{x\left(x+20\right)}=\dfrac{1}{12}\)
=>\(x\left(x+20\right)=400\cdot12=4800\)
=>\(x^2+20x-4800=0\)
=>(x+80)(x-60)=0
=>\(\left[{}\begin{matrix}x+80=0\\x-60=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-80\left(nhận\right)\\x=60\left(nhận\right)\end{matrix}\right.\)

Tỉ số giữa Số học sinh khá và cả lớp là:
\(\dfrac{1}{2}\left(1-\dfrac{1}{4}\right)=\dfrac{1}{2}\cdot\dfrac{3}{4}=\dfrac{3}{8}\)
Số học sinh trung bình chiếm: \(1-\dfrac{3}{8}-\dfrac{1}{4}=\dfrac{5}{8}-\dfrac{2}{8}=\dfrac{3}{8}\)(số học sinh cả lớp)
Số học sinh cả lớp là \(24:\dfrac{3}{8}=24\cdot\dfrac{8}{3}=64\left(bạn\right)\)
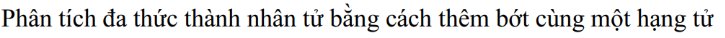
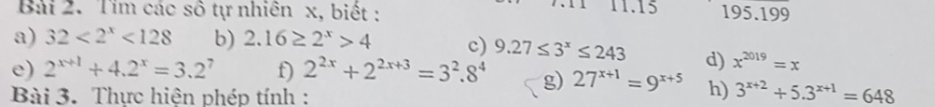
\(42\cdot136+272\cdot15+28\cdot68\cdot2\)
\(=42\cdot136+30\cdot136+28\cdot136\)
\(=136\left(42+30+28\right)\)
\(=136\cdot100=13600\)
42 x 136 + 272 x 15 + 28 x 68 x 2
= 21 x (2 x 136) + 272 x 15 + (68 x 4) x 7 x 2
= 21 x 272 + 272 x 15 + 272 x 14
= 272 x (21 + 15 + 14)
= 272 x 50
= 13600