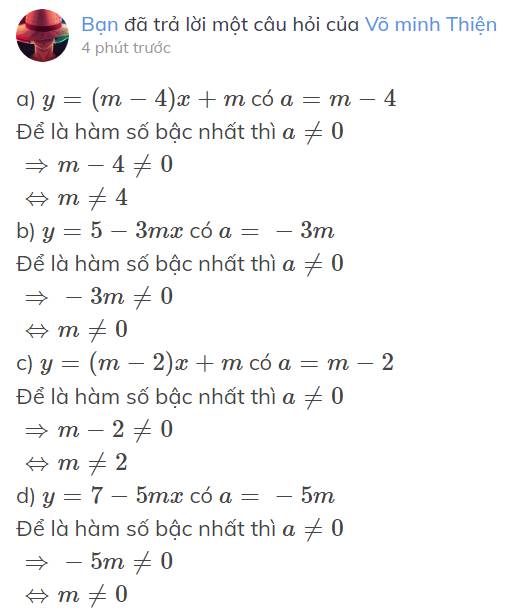
Bài 2:

a) ĐKXĐ: \(\left\{{}\begin{matrix}x+3\ne0\\x-3\ne0\\9-x^2\ne0\end{matrix}\right.\Leftrightarrow x\ne\pm3\)

b) \(A=\dfrac{3}{x+3}+\dfrac{1}{x-3}-\dfrac{18}{9-x^2}\)

\(A=\dfrac{3}{x+3}+\dfrac{1}{x-3}+\dfrac{18}{x^2-9}\)

\(A=\dfrac{3\left(x-3\right)}{\left(x+3\right)\left(x-3\right)}+\dfrac{x+3}{\left(x+3\right)\left(x-3\right)}+\dfrac{18}{\left(x+3\right)\left(x-3\right)}\)

\(A=\dfrac{3x-9+x+3+18}{\left(x+3\right)\left(x-3\right)}\)

\(A=\dfrac{4x+12}{\left(x+3\right)\left(x-3\right)}\)

\(A=\dfrac{4\left(x+3\right)}{\left(x+3\right)\left(x-3\right)}\)

\(A=\dfrac{4}{x-3}\) 

c) Thay `x=-1` vào A ta có:

\(A=\dfrac{4}{-1-3}=\dfrac{4}{-4}=-1\)

d) `A=-4` khi: \(\dfrac{4}{x-3}=-4\)

\(\Leftrightarrow x-3=-1\)

\(\Leftrightarrow x=2\left(tm\right)\)

Bài 1:

a: ĐKXĐ: x<>3

\(\dfrac{9}{x-3}+\dfrac{3x}{3-x}\)

\(=\dfrac{9}{x-3}-\dfrac{3x}{x-3}=\dfrac{9-3x}{x-3}\)

\(=\dfrac{-3\left(x-3\right)}{x-3}=-3\)

b: \(\dfrac{5}{x+5}+\dfrac{-4}{x+4}\)

\(=\dfrac{5\left(x+4\right)-4\left(x+5\right)}{\left(x+5\right)\left(x+4\right)}\)

\(=\dfrac{5x+20-4x-20}{\left(x+5\right)\left(x+4\right)}=\dfrac{x}{\left(x+5\right)\left(x+4\right)}\)

c: \(\dfrac{x+5}{2x-3}-\dfrac{2x-7}{3-2x}-\dfrac{x+4}{3-2x}\)

\(=\dfrac{x+5}{2x-3}+\dfrac{2x-7}{2x-3}+\dfrac{x+4}{2x-3}\)

\(=\dfrac{x+5+2x-7+x+4}{2x-3}\)

\(=\dfrac{4x+2}{2x-3}\)

d: \(\dfrac{x^2-y^2}{10x^3y}:\dfrac{x-y}{5xy}\)

\(=\dfrac{\left(x-y\right)\left(x+y\right)}{10x^3y}\cdot\dfrac{5xy}{x-y}\)

\(=\dfrac{x+y}{1}\cdot\dfrac{5xy}{10x^3y}\)

\(=\dfrac{x+y}{2x^2}\)

e: \(\dfrac{2x^2-20x+50}{3x+3}\cdot\dfrac{x^2-1}{4\left(x-5\right)^3}\)

\(=\dfrac{2\left(x^2-10x+25\right)}{3\left(x+1\right)}\cdot\dfrac{\left(x+1\right)\left(x-1\right)}{4\left(x-5\right)^3}\)

\(=\dfrac{2\left(x-5\right)^2}{4\left(x-5\right)^3}\cdot\dfrac{x-1}{3}\)

\(=\dfrac{x-1}{3\cdot2\left(x-5\right)}=\dfrac{x-1}{6x-30}\)

f: \(\dfrac{x-2}{x+1}:\dfrac{x^2-5x+6}{x^2-2x-3}\)

\(=\dfrac{x-2}{x+1}:\dfrac{\left(x-2\right)\left(x-3\right)}{\left(x-3\right)\left(x+1\right)}\)

\(=\dfrac{x-2}{x+1}\cdot\dfrac{\left(x+1\right)}{x-2}=1\)

g: \(\dfrac{x}{x-2y}+\dfrac{x}{x+2y}+\dfrac{4xy}{4y^2-x^2}\)

\(=\dfrac{x}{x-2y}+\dfrac{x}{x+2y}-\dfrac{4xy}{\left(x-2y\right)\left(x+2y\right)}\)

\(=\dfrac{x\left(x+2y\right)+x\left(x-2y\right)-4xy}{\left(x-2y\right)\left(x+2y\right)}\)

\(=\dfrac{2x^2-4xy}{\left(x-2y\right)\left(x+2y\right)}=\dfrac{2x\left(x-2y\right)}{\left(x-2y\right)\left(x+2y\right)}=\dfrac{2x}{x+2y}\)

h: \(\dfrac{1}{x-y}+\dfrac{3xy}{y^3-x^3}+\dfrac{x-y}{x^2+xy+y^2}\)

\(=\dfrac{1}{x-y}-\dfrac{3xy}{\left(x-y\right)\cdot\left(x^2+xy+y^2\right)}+\dfrac{x-y}{x^2+xy+y^2}\)

\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)

i: \(\left(\dfrac{2}{x+2}+\dfrac{2}{x-1}\right)\cdot\dfrac{x^2-4}{4x^2-1}\)

\(=\dfrac{2\left(x-1\right)+2\left(x+2\right)}{\left(x+2\right)\left(x-1\right)}\cdot\dfrac{\left(x-2\right)\left(x+1\right)}{\left(2x-1\right)\left(2x+1\right)}\)

\(=\dfrac{2\left(2x+1\right)}{x-1}\cdot\dfrac{x+1}{\left(2x-1\right)\left(2x+1\right)}=\dfrac{2\left(x+1\right)}{\left(2x-1\right)\left(x-1\right)}\)

j: \(1+\dfrac{x^3-x}{x^2+1}\cdot\left(\dfrac{1}{1-x}-\dfrac{1}{1-x^2}\right)\)

\(=1+\dfrac{x\left(x-1\right)\left(x+1\right)}{x^2+1}\cdot\left(\dfrac{-1}{x-1}+\dfrac{1}{\left(x-1\right)\left(x+1\right)}\right)\)

\(=1+\dfrac{x\left(x-1\right)\left(x+1\right)}{x^2+1}\cdot\dfrac{-x-1+1}{\left(x-1\right)\left(x+1\right)}\)

\(=1+\dfrac{x\left(x-1\right)\left(x+1\right)}{x^2+1}\cdot\dfrac{-x}{\left(x-1\right)\left(x+1\right)}\)

\(=1-\dfrac{x^2}{x^2+1}=\dfrac{1}{x^2+1}\)

Pt: \(\dfrac{3}{x^2+x+1}+\dfrac{4}{x^2+x+2}-\dfrac{6}{x^2+x+4}=1\) (*)

ĐK: \(\left\{{}\begin{matrix}x^2+x+1\ne0\\x^2+x+2\ne0\\x^2+x+4\ne0\end{matrix}\right.\)(luôn đúng)

Đặt: \(x^2+x+2=t\ge\dfrac{7}{4}\)

(*) trở thành:

\(\dfrac{3}{t-1}+\dfrac{4}{t}-\dfrac{6}{t+2}=1\)  

\(\Leftrightarrow\dfrac{3t\left(t+2\right)}{t\left(t-1\right)\left(t+2\right)}+\dfrac{4\left(t-1\right)\left(t+2\right)}{t\left(t-1\right)\left(t+2\right)}-\dfrac{6t\left(t-1\right)}{t\left(t-1\right)\left(t+2\right)}=1\)

\(\Leftrightarrow3t\left(t+2\right)+4\left(t-1\right)\left(t+2\right)-6t\left(t-1\right)=t\left(t-1\right)\left(t+2\right)\)

\(\Leftrightarrow3t^2+6t+4\left(t^2+t-2\right)-6t^2+6t=t\left(t^2+t-2\right)\)

\(\Leftrightarrow-3t^2+12t+4t^2+4t-8=t^3+t^2-2t\)

\(\Leftrightarrow t^2+16t-8=t^3+t^2-2t\)

\(\Leftrightarrow t^3-18t+8=0\)

\(\Leftrightarrow\left(t-4\right)\left(t^2+4t-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}t=4\left(tm\right)\\t=\sqrt{6}-2\left(ktm\right)\\t=-\sqrt{6}-2\left(ktm\right)\end{matrix}\right.\)

\(\Rightarrow x^2+x+2=4\)

\(\Leftrightarrow x^2+x-2=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)

Vậy: ... 

gọi chiều dài,chiều rộng là:a,b

Theo bài

(a+4)(b+2)-ab=8

ab+4b+2a+8-ab=8

2a+4b+8=8

Nửa chu vi là:36:2=18

=>36+2b+8=8

44+2b=8

2b=8-44

2b=-36

b=-18

=>a là : 36

em nghĩ bài này chx chắc nên anh tham khảo

Nửa chu vi mảnh vườn là:

36:2=18(m)

Gọi chiều dài mảnh vườn là x(m)

(ĐK: 0<x<18)

Chiều rộng mảnh vườn là 18-x(m)

Chiều dài khi tăng thêm 4m là x+4(m)

Chiều rộng khi giảm đi 2m là 18-x-2=16-x(m)

Diện tích tăng thêm 8m² nên ta có:
(x+4)(16-x)-x(18-x)=8
=>\(16x-x^2+64-4x-18x+x^2=8\)

=>-6x=8-64=-56

=>\(x=\dfrac{56}{6}=\dfrac{28}{3}\left(nhận\right)\)

Vậy: Chiều dài mảnh vườn là 28/3(m)

Chiều rộng mảnh vườn là \(18-\dfrac{28}{3}=\dfrac{26}{3}\left(m\right)\)

a: Xét ΔOAB và ΔOCD có

\(\widehat{OAB}=\widehat{OCD}\)(hai góc so le trong, AB//CD)

\(\widehat{AOB}=\widehat{COD}\)(hai góc đối đỉnh)

Do đó: ΔOAB~ΔOCD

b: Sửa đề: cắt BC tại N

Xét ΔADC có OM//DC
nên \(\dfrac{OM}{DC}=\dfrac{AO}{AC}\)

=>\(OM\cdot AC=DC\cdot AO\)

c: Xét ΔADC có OM//DC

nên \(\dfrac{OM}{DC}=\dfrac{AO}{AC}\)(1)

Xét ΔBDC có ON//DC

nên \(\dfrac{ON}{DC}=\dfrac{BO}{BD}\left(2\right)\)

Ta có: ΔOAB~ΔOCD

=>\(\dfrac{OA}{OC}=\dfrac{OB}{OD}\)

=>\(\dfrac{OC}{OA}=\dfrac{OD}{OB}\)

=>\(\dfrac{OC+OA}{OA}=\dfrac{OD+OB}{OB}\)

=>\(\dfrac{AC}{OA}=\dfrac{BD}{OB}\)

=>\(\dfrac{OA}{AC}=\dfrac{OB}{BD}\left(3\right)\)

Từ (1),(2),(3) suy ra OM=ON

=>O là trung điểm của MN