Bài 3: Tính giá trị của

a, M = 3\(x^2\)y² - 2\(xy\) - 1 tại \(x\) = 1; \(y\) = -2 

Thay \(x\) = 1; \(y\) = -2 vào biểu thức M = 3\(x^2\)y² - 2\(xy\) - 1 ta có:

M = 3.(1)².(-2)² - 2.(1)².(-2) = 12 + 4 = 16

b, N = -\(x\)³ + 2\(x\)² - 1 tại \(x\) = 2 

Thay \(x\) = 2 vào biểu thức N = - \(x^3\) + 2\(x^2\) - 1 ta có:

N = -(2)³ +2.(2)² - 1 = -1

c, Không rõ tại \(x\) = ?; \(y\) = ?

d, Q = 2\(x^9\) - 5\(y\)⁸ + 4 và \(x;y\) thỏa mãn (\(x-1\))²⁰²⁰ +(\(y\) +1)²⁰²⁰

Ta có: \(\left\{{}\begin{matrix}\left(x-1\right)^{2020}\ge0\forall x\\\left(y+1\right)^{2020}\ge0\forall x\end{matrix}\right.\)

     ⇒ (\(x-1\))²⁰²⁰+(y+1)²⁰²⁰ = 0 

      ⇔ \(\left\{{}\begin{matrix}x-1=0\\y+1=0\end{matrix}\right.\) ⇔ \(\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)

Thay \(\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\) vào biểu thức:

Q = 2\(x^9\) - 5\(y^8\) + 4 ta có:

Q = 2.(1)⁹ - 5.(-1)⁸ + 4 = 2  - 5 + 4 = 1

giúp mình với ạ

Với x ≥ 0 thì \(\sqrt{x}\ge0\) nên \(\sqrt{x}+1\ge1\)

Khi đó \(B=\left(\sqrt{x}+1\right)^{99}+2022\ge1^{99}+2022\)

Hay \(B=\left(\sqrt{x}+1\right)^{99}+2022\ge2023\)

Dấu "=" xảy ra khi \(\sqrt{x}=0\) hay x = 0

Vậy GTNN của \(B=\left(\sqrt{x}+1\right)^{99}+2022\) là 2023 khi x = 0

\(B=\left(\sqrt{x}+1\right)^{99}+2022\left(x\ge0\right)\)

Vì: \(x\ge0\)

Nên => \(\left(\sqrt{x}+1\right)^{99}\ge0\)

=> \(\left(\sqrt{x}+1\right)^{99}+2022\ge2022\)

=> \(B\ge2022\)

Dấu " = " xảy ra khi: \(\Leftrightarrow\sqrt{x}+1=0\Leftrightarrow\sqrt{x}=-1\left(voli\right)\)

Vậy: B không có giá trị nhỏ nhất

Lời giải:

$27\equiv 1\pmod {13}$

$\Rightarrow 27^{12}\equiv 1^{12}\equiv 1\pmod {13}(1)$

$43\equiv 4\pmod {13}\Rightarrow 43^7\equiv 4^7\pmod {13}(2)$

$9\equiv -4\pmod {13}\Rightarrow 9^{17}\equiv (-4)^{17}\pmod {13}(3)$

Từ $(1); (2); (3)\Rightarrow 27^{12}+43^7+9^{17}\equiv 1+4^7+(-4)^{17}$

$\equiv 1+4^7(1-4^{10})\pmod {13}$

Mà: 
$4^3\equiv -1\pmod {13}$

$\Rightarrow 4^7=(4^3)^2.4\equiv (-1)^2.4\equiv 4\pmod {13}$

$4^{10}=(4^3)^3.4\equiv (-1)^3.4\equiv -4\pmod {13}$

$\Rightarrow 27^{12}+43^7+9^{17}\equiv 1+4^7(1-4^{10})\equiv 1+4(1--4)\equiv 21\equiv 8\pmod {13}$

Tức là tổng trên không chia hết cho 13 bạn nhé.

Lời giải:

Gọi mẫu của ps đó là $x$ với $x$ là số nguyên. Theo bài ra ta có:

$\frac{-11}{3}< \frac{-9}{x}< \frac{-11}{5}$

$\Rightarrow \frac{11}{3}> \frac{9}{x}> \frac{11}{5}$

$\Rightarrow \frac{99}{27}> \frac{99}{11x}> \frac{99}{45}$

$\Rightarrow 27< 11x< 45$

$\Rightarrow 2< x< 5$

$\Rightarrow $x=3$ hoặc $x=4$. Vậy hai phân số cần tìm là $\frac{-9}{3}$ và $\frac{-9}{4}$

\(-32:\left(-2\right)^n=4\\ =>\left(-2\right)^n=\left(-32\right):4=-8=\left(-2\right)^3\\ =>n=3\)

\(\left|x\right|+x=\dfrac{1}{3}\)

\(\Rightarrow\left|x\right|=\dfrac{1}{3}-x\)

\(\left|x\right|=\left\{{}\begin{matrix}xkhix\ge0\\-xkhix< 0\end{matrix}\right.\)

Với \(x\ge0\Rightarrow x=\dfrac{1}{3}-x\Rightarrow2x=\dfrac{1}{3}\Rightarrow x=\dfrac{1}{6}\left(tm\right)\)

Với \(x< 0\Rightarrow-x=\dfrac{1}{3}-x\Rightarrow-x+x=\dfrac{1}{3}\Rightarrow0=\dfrac{1}{3}\left(VL\right)\)

Vậy \(x=\dfrac{1}{6}\)

\(\left|x\right|+x=\dfrac{1}{3}\left(1\right)\)

TH1 : \(x\ge0\)

\(\left(1\right)=>x+x=\dfrac{1}{3}\\ =>2x=\dfrac{1}{3}\\ =>x=\dfrac{1}{3}:2=\dfrac{1}{6}\left(TMDK\right)\)

\(TH2:x< 0\)

\(\left(1\right)=>-x+x=\dfrac{1}{3}\\ =>0=\dfrac{1}{3}\)( Vô lí )

Vậy `x=1/6`

\(2.16\ge2^n>4\)

\(2.2^4\ge2^n>2^2\)

\(2^5\ge2^n>2^2\)

=> \(n\in\left\{3,4,5\right\}\)

Vậy: \(n\in\left\{3,4,5\right\}\)

a) Để A là phân số thì : \(n-2\ne0=>n\ne2\)

b) Để A nhận giá trị nguyên âm lớn nhất 

\(=>A=-1\\ =>\dfrac{n-6}{n-2}=-1\\ =>n-6=-\left(n-2\right)\\ =>n-6=-n+2\\ =>n+n=6+2\\ =>2n=8\\ =>n=4\left(TMDK\right)\)

c) \(A=\dfrac{n-6}{n-2}=\dfrac{n-2-4}{n-2}=1-\dfrac{4}{n-2}\)

Để A nhận gt số nguyên thì : \(\dfrac{4}{n-2}\in Z=>4⋮\left(n-2\right)\\ =>n-2\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\\ =>n\in\left\{3;1;4;0;6;-2\right\}\)

Đến đây bạn lập bảng giá trị rồi thay từng gt n vào bt A, giá trị nào cho A là STN thì bạn nhận gt đó ạ.

d) Mình nghĩ bạn thiếu đề ạ 

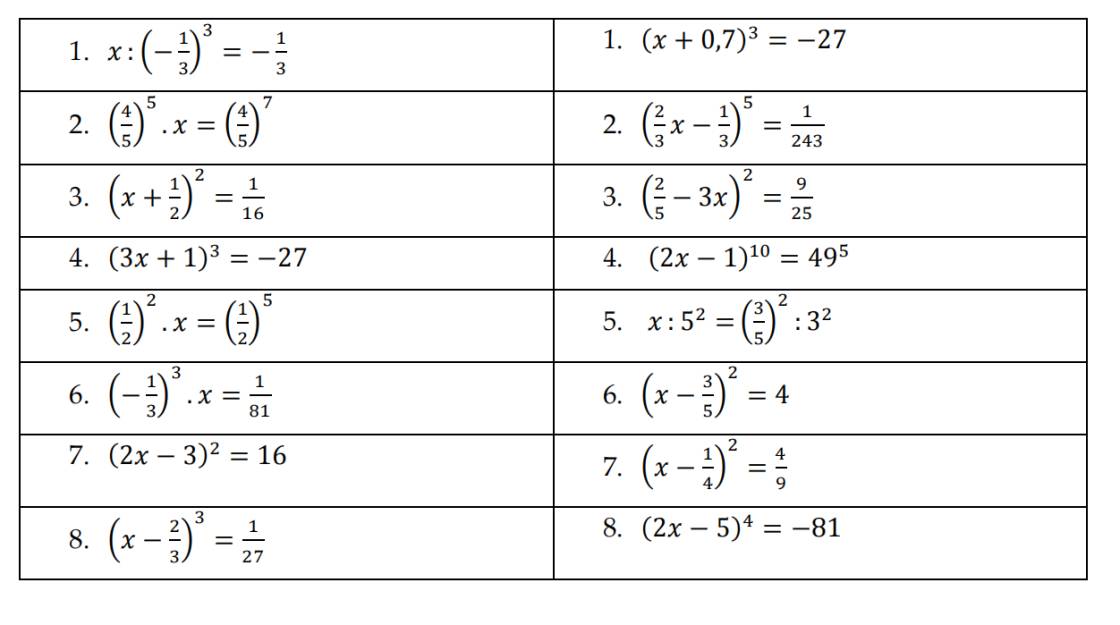
\(1,x:\left(-\dfrac{1}{3}\right)^3=\left(-\dfrac{1}{3}\right)\\ \Leftrightarrow x=\left(-\dfrac{1}{3}\right)\times\left(-\dfrac{1}{3}\right)^3\\ \Leftrightarrow x=\left(-\dfrac{1}{3}\right)^4=\dfrac{1}{81}\\ 2,\left(\dfrac{4}{5}\right)^5.x=\left(\dfrac{4}{5}\right)^7\\ \Leftrightarrow x=\left(\dfrac{4}{5}\right)^7:\left(\dfrac{4}{5}\right)^5=\left(\dfrac{4}{5}\right)^{7-5}=\left(\dfrac{4}{5}\right)^2=\dfrac{16}{25}\)

\(3,\left(x+\dfrac{1}{2}\right)^2=\dfrac{1}{16}\\ \Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{2}=\dfrac{1}{4}\\x+\dfrac{1}{2}=-\dfrac{1}{4}\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{4}\\x=-\dfrac{3}{4}\end{matrix}\right.\)

\(4,\left(3x+1\right)^3=-27\\ \Leftrightarrow\left(3x+1\right)^3=\left(-3\right)^3\\ \Leftrightarrow3x+1=-3\\ \Leftrightarrow3x=-4\\ \Leftrightarrow x=-\dfrac{4}{3}\)

\(5,\left(\dfrac{1}{2}\right)^2.x=\left(\dfrac{1}{2}\right)^5\\ \Leftrightarrow x=\left(\dfrac{1}{2}\right)^5:\left(\dfrac{1}{2}\right)^2\\ \Leftrightarrow x=\left(\dfrac{1}{2}\right)^{5-2}=\left(\dfrac{1}{2}\right)^3=\dfrac{1}{8}\)

\(6,\left(-\dfrac{1}{3}\right)^3.x=\dfrac{1}{81}\\ \Leftrightarrow\left(-\dfrac{1}{3}\right)^3.x=\left(-\dfrac{1}{3}\right)^4\\ \Leftrightarrow x=\left(-\dfrac{1}{3}\right)^4:\left(-\dfrac{1}{3}\right)^3=-\dfrac{1}{3}\)

\(7,\left(2x-3\right)^2=16\\ \Leftrightarrow\left[{}\begin{matrix}2x-3=4\\2x-3=-4\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=-\dfrac{1}{2}\end{matrix}\right.\)

\(8,\left(x-\dfrac{2}{3}\right)^3=\dfrac{1}{27}\\ \Leftrightarrow\left(x-\dfrac{2}{3}\right)^3=\left(\dfrac{1}{3}\right)^3\\ \Leftrightarrow x-\dfrac{2}{3}=\dfrac{1}{3}\\ \Leftrightarrow x=\dfrac{1}{3}+\dfrac{2}{3}=\dfrac{3}{3}=1\)

`@` `\text {Ans}`

`\downarrow`

(Vế 1)

`1.`

`x \div(-1/3)^3 =-1/3`

`=> x= (-1/3) \times (-1/3)^3`

`=> x= (-1/3)^4`

`2.`

`(4/5)^5 *x = (4/5)^7`

`=> x = (4/5)^7 \div (4/5)^5`

`=> x=(4/5)^2`

`3.`

`(x+1/2)^2 =1/16`

`=> (x+1/2)^2 = (+-1/4)^2`

`=>`\(\left[{}\begin{matrix}x+\dfrac{1}{2}=\dfrac{1}{4}\\x+\dfrac{1}{2}=-\dfrac{1}{4}\end{matrix}\right.\)

`=>`\(\left[{}\begin{matrix}x=\dfrac{1}{4}-\dfrac{1}{2}\\x=-\dfrac{1}{4}-\dfrac{1}{2}\end{matrix}\right.\)

`=>`\(\left[{}\begin{matrix}x=-\dfrac{1}{4}\\x=-\dfrac{3}{4}\end{matrix}\right.\)

`4.`

`(3x+1)^3 = -27`

`=> (3x+1)^3 = (-3)^3`

`=> 3x+1=-3`

`=> 3x=-3-1`

`=> 3x =-4`

`=> x=-4/3`

`5.`

`(1/2)^2*x=(1/2)^5`

`=> x=(1/2)^5 \div (1/2)^2`

`=> x=(1/2)^3`

`6.`

`(-1/3)^3*x=1/81`

`=> (-1/3)^3*x = (1/3)^4`

`=> x= (1/3)^4 \div (-1/3)^3`

`=> x=(-1/3)`

`7.`

`(2x-3)^2 = 16`

`=> (2x-3)^2 = (+-4)^2`

`=>`\(\left[{}\begin{matrix}2x-3=4\\2x-3=-4\end{matrix}\right.\)

`=>`\(\left[{}\begin{matrix}2x=7\\2x=-1\end{matrix}\right.\)

`=>`\(\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=-\dfrac{1}{2}\end{matrix}\right.\)

`8.`

`(x-2/3)^3 = 1/27`

`=> (x-2/3)^3 = (1/3)^3`

`=> x-2/3=1/3`

`=> x=1/3 + 2/3`

`=> x=1`

\(9.27\le3n\le243\\ =>9.27:3\le3n:3\le243:3\\=>81\le n\le81\\ =>n=81\)

\(9.27\le3^n\le243\)

\(3.3^3\le3^n\le3^5\)

\(3^4\le3^n\le3^5\)

\(n\in\left\{4,5\right\}\)

Vậy: \(n\in\left\{4,5\right\}\)