\(A=\dfrac{3x-1}{x+2}\inℕ\left(x\inℕ;x\ne-2\right)\)

\(\Rightarrow3x-1⋮x+2\)

\(\Rightarrow3x-1-3\left(x+2\right)⋮x+2\)

\(\Rightarrow3x-1-3x-6⋮x+2\)

\(\Rightarrow-7⋮x+2\)

\(\Rightarrow x+2\in U\left(7\right)=\left\{1;7\right\}\)

\(\Rightarrow x\in\left\{-1;5\right\}\)

\(\Rightarrow x\in\left\{5\right\}\left(x\inℕ\right)\)

          \(\dfrac{x-6}{1998}\) + \(\dfrac{x-4}{2000}\) = \(\dfrac{x-2000}{4}\) + \(\dfrac{x-1998}{6}\)

\(\dfrac{x-6}{1998}\) - 1 + \(\dfrac{x-4}{2000}\) - 1 = \(\dfrac{x-2000}{4}\) - 1 + \(\dfrac{x-1998}{6}\) - 1

\(\dfrac{x-6-1998}{1998}\) + \(\dfrac{x-4-2000}{2000}\)  = \(\dfrac{x-2000-4}{4}\) + \(\dfrac{x-1998-6}{6}\)

\(\dfrac{x-2004}{1998}\)  + \(\dfrac{x-2004}{2000}\) = \(\dfrac{x-2004}{4}\) + \(\dfrac{x-2004}{6}\)

(\(x-2004\)).[\(\dfrac{1}{1998}\) + \(\dfrac{1}{2000}\) - \(\dfrac{1}{4}\) - \(\dfrac{1}{6}\)] = 0

\(x\) - 2004 = 0

\(x\)             = 2004

\(\dfrac{x+1}{65}+\dfrac{x+3}{63}+\dfrac{x+5}{61}+\dfrac{x+7}{59}\)

\(\Leftrightarrow\dfrac{x+1}{65}+\dfrac{x+3}{63}-\dfrac{x+5}{61}-\dfrac{x+7}{59}=0\)

\(\left(\dfrac{x+1}{65}+1\right)+\left(\dfrac{x+3}{63}+1\right)-\left(\dfrac{x+5}{61}+1\right)-\left(\dfrac{x+7}{59}+1\right)\)

\(\Leftrightarrow\dfrac{x+66}{65}+\dfrac{x+66}{63}+\dfrac{x+66}{61}+\dfrac{x+66}{59}=0\)

\(\Leftrightarrow\left(x+66\right).\left[\left(\dfrac{1}{65}+\dfrac{1}{63}\right)-\left(\dfrac{1}{61}+\dfrac{1}{59}\right)\right]\)\(=0\)

Do \(\dfrac{1}{65}< \dfrac{1}{63}< \dfrac{1}{61}< \dfrac{1}{59}\) 

\(\Rightarrow\left(\dfrac{1}{65}+\dfrac{1}{63}\right)-\left(\dfrac{1}{61}+\dfrac{1}{59}\right)< 0\)

Vậy để \(\left(x+66\right).\left[\left(\dfrac{1}{65}+\dfrac{1}{63}\right)-\left(\dfrac{1}{61}+\dfrac{1}{59}\right)\right]=0\)

\(\Leftrightarrow x+66=0\)

\(\Leftrightarrow x=-66\)

Vậy \(x\in\left\{-66\right\}\)

\(\left(\dfrac{1}{10}\right)^{15}=\left[\left(\dfrac{1}{10}\right)^3\right]^5=\left(\dfrac{1}{1000}\right)^5=\left(\dfrac{10}{10000}\right)^5\)

\(\left(\dfrac{3}{10}\right)^{20}=\left[\left(\dfrac{3}{10}\right)^4\right]^5=\left(\dfrac{81}{10000}\right)^5\)

 \(\dfrac{10}{10000}< \dfrac{81}{10000}\)

\(\Rightarrow\left(\dfrac{10}{10000}\right)^5< \left(\dfrac{81}{10000}\right)^5\)

\(\Rightarrow\left(\dfrac{1}{10}\right)^{15}< \left(\dfrac{3}{10}\right)^{20}\)

Ta có:

\(\left(\dfrac{1}{10}\right)^{15}=\left[\left(\dfrac{1}{10}\right)^3\right]^5=\left(\dfrac{1}{1000}\right)^5\)

\(\left(\dfrac{3}{10}\right)^{20}=\left[\left(\dfrac{3}{10}\right)^4\right]^5=\left(\dfrac{81}{10000}\right)^5\)

Ta thấy: \(\dfrac{1}{1000}< \dfrac{81}{10000}\)

\(\Rightarrow\left(\dfrac{1}{1000}\right)^5< \left(\dfrac{81}{10000}\right)^5\)

\(\Rightarrow\left(\dfrac{1}{10}\right)^{15}< \left(\dfrac{3}{10}\right)^{20}\)

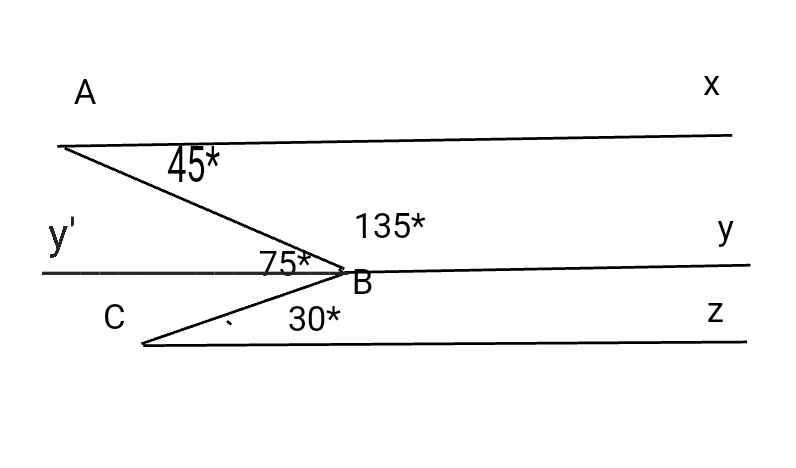
a) Vẽ tia By' là tia đối của tia By

Ta có:

∠ABy' + ∠ABy = 180⁰ (kề bù)

⇒ ∠ABy' = 180⁰ - ∠ABy

= 180⁰ - 135⁰

= 45⁰

⇒ ∠ABy' = ∠BAx = 45⁰

Mà ∠ABy' và ∠BAx là hai góc so le trong

⇒ By // Ax

b) Ta có:

∠CBy' = ∠ABC - ∠ABy'

= 75⁰ - 45⁰

= 30⁰

⇒ ∠CBy' = ∠BCz = 30⁰

Mà ∠CBy' và ∠BCz là hai góc so le trong

⇒ By // Cz

\(a^m=a^n\)

\(\Rightarrow m=n\)

Với \(a^m=a^n\) mọi \(m=n\) 

Vậy: \(m=n\in\left\{1;2;3;4;...\right\}\)

m = n vô số nha

Tìm \(x\) biết: |\(x\) + 1| + |\(x\) + 4| = 3\(x\) ( đk \(x\) ≥ 0)

                        |\(x\) + 1| + | \(x\) + 4| = 3\(x\)

Với \(x\) ≥ 0 ta có: \(x\) + 1 + \(x\) + 4 = 3\(x\)

                         2\(x\) + 5   = 3\(x\)

                         3\(x\) - 2\(x\) = 5

                            \(x\) = 5 (thỏa mãn)

Vậy \(x\) = 5 

\(\left|x+1\right|+\left|x+4\right|=3x\left(1\right)\)

Ta có :

\(\left|x+1\right|+\left|x+4\right|\ge\left|x+1+x+4\right|=\left|2x+5\right|\)

\(pt\left(1\right)\Leftrightarrow\left|2x+5\right|=3x\)

\(\Leftrightarrow\left[{}\begin{matrix}2x+5=3x\\2x+5=-3x\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=5\\5x=-5\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-1\end{matrix}\right.\)

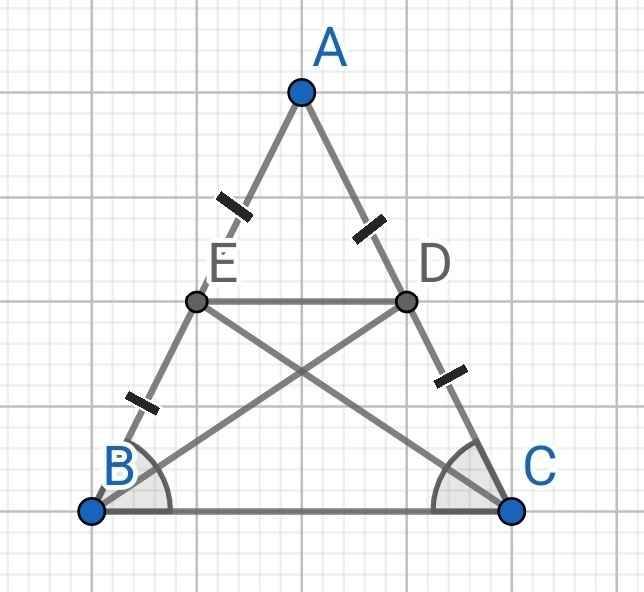 a) Do ∆ABC cân tại A

⇒ AB = AC (1)

Do BD là đường trung tuyến

⇒ D là trung điểm của AC

⇒ AD = CD (2)

Do CE là đường trung tuyến

⇒ E là trung điểm của AB

⇒ AE = BE (3)

Từ (1), (2) và (3)

⇒ AE = AD

∆AED có:

⇒ AE = AD (cmt)

⇒ ∆AED cân tại A

b) ∆AED cân tại A (cmt)

⇒ ∠AED = ∠ADE = (180⁰ - ∠A) : 2 (4)

∆ABC cân tại A

⇒ ∠ABC = ∠ACB = (180⁰ - ∠A) : 2 (5)

Từ (4) và (5)

⇒ ∠AED = ∠ABC

Mà ∠AED và ∠ABC là hai góc đồng vị

⇒ ED // BC

Tứ giác BCDE có:

ED // BC (cmt)

⇒ BCDE là hình thang

Mà ∠CBE = ∠BCD (∆ABC cân tại A)

⇒ BCDE là hình thang cân

Ai giúp tui đi mà :((

\(a=2^{100}=\left(2^4\right)^{25}=16^{25}\)

\(b=3^{75}=\left(3^3\right)^{25}=27^{25}\)

\(c=5^{50}=\left(5^2\right)^{25}=25^{25}\)

Vì \(16^{25}< 25^{25}< 27^{25}\)

\(\Rightarrow a< c< b\)

\(a=2^{100},b=3^{75},c=5^{50}\\ \Rightarrow a=30^{85},b=30^{65},c=30^{44}\\ \Rightarrow a>b>c\)

Ta gọi \(x;y;z\) là số tờ tiền loại \(2000;5000;10000\)

Tổng giá trị 3 cọc tiền là:

\(2000.x+5000.y+10000.z\)

Giá trị 3 cọc tiền bằng nhau là:

\(2000.x=5000.y=10000.z\)

\(\Leftrightarrow\dfrac{x}{5000.10000}=\dfrac{y}{2000.10000}=\dfrac{z}{2000.5000}\)

\(=\dfrac{x+y+z}{50000000+20000000+10000000}\)

\(=\dfrac{72}{80000000}=\dfrac{9}{10000000}\)

Áp dụng tính chất dãy tỉ số bằng nhau ta có:

\(\Rightarrow x=50000000.\dfrac{9}{10000000}=45\)

\(\Rightarrow y=20000000.\dfrac{9}{10000000}=18\)

\(\Rightarrow z=10000000.\dfrac{9}{10000000}=9\)

Vậy loại \(2000\) đồng có \(45\) tờ

Loại \(5000\) đồng có \(18\) tờ

Loại \(10000\) đồng có \(9\) tờ