Giá trị rút gọn của biểu thức là .......
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\dfrac{8-2x}{x^2+x-20}=-\dfrac{2\left(4-x\right)}{\left(4-x\right)\left(x+5\right)}=\dfrac{-2}{x+5}\)
Để biểu thức trên nhận giá trị dương khi
\(x+5< 0\)do -2 < 0
\(\Leftrightarrow x< -5\)

\(\left(\dfrac{\dfrac{x}{x+1}}{\dfrac{x^2}{x^2+x+1}}-\dfrac{2x+1}{x^2+x}\right)\dfrac{x^2-1}{x-1}\)ĐK : \(x\ne\pm1\)
\(=\left(\dfrac{x}{x+1}.\dfrac{x^2+x+1}{x^2}-\dfrac{2x+1}{x\left(x+1\right)}\right)\left(x+1\right)=\left(\dfrac{x^2+x-1}{x^2+x}-\dfrac{2x+1}{x\left(x+1\right)}\right)\left(x+1\right)\)
\(=\left(\dfrac{x^2+x-1-2x-1}{x\left(x+1\right)}\right)\left(x+1\right)=\dfrac{x^2-3x-2}{x}\)
à xin lỗi mình nhầm dòng cuối
\(=\dfrac{x^2-x-2}{x}=\dfrac{\left(x+1\right)\left(x-2\right)}{x}\)
Để biểu thức trên nhận giá trị dương khi
\(\dfrac{\left(x+1\right)\left(x-2\right)}{x}>0\)bạn tự xét TH cả tử và mẫu nhé, mình đánh trên này bị lỗi

a) Ta có: \(P=\dfrac{x^2-\sqrt{x}}{x+\sqrt{x}+1}-\dfrac{2x+\sqrt{x}}{\sqrt{x}}+\dfrac{2\left(x-1\right)}{\sqrt{x}-1}\)
\(=\sqrt{x}\left(\sqrt{x}-1\right)-2\sqrt{x}-1+2\left(\sqrt{x}+1\right)\)
\(=x-\sqrt{x}-2\sqrt{x}-1+2\sqrt{x}+2\)
\(=x-\sqrt{x}+1\)

a) a ≠ 0 , a ≠ − 5
b) Ta có A = a 3 + 4 a 2 − 5 a 2 a ( a + 5 ) = a ( a − 1 ) ( a + 5 ) 2 a ( a + 5 ) = a − 1 2
c) Thay a = -1 (TMĐK) vào a ta được A = -1
d) Ta có A = 0 Û a = 1 (TMĐK)

a: Khi x=16/9 thì \(A=\left(\dfrac{4}{3}-2\right):\left(\dfrac{4}{3}-3\right)=\dfrac{-2}{3}:\dfrac{-5}{3}=\dfrac{2}{5}\)
b: \(=\dfrac{x+2\sqrt{x}+3\sqrt{x}-6-9\sqrt{x}-10}{x-4}\)
\(=\dfrac{x-4\sqrt{x}-16}{x-4}\)

a) ĐK:\(\begin{cases} x + 2≠0\\ x - 2≠0 \end{cases}\)⇔\(\begin{cases} x ≠ -2\\ x≠ 2 \end{cases}\)
Vậy biểu thức P xác định khi x≠ -2 và x≠ 2
b) P= \(\dfrac{3}{x+2}\)-\(\dfrac{2}{2-x}\)-\(\dfrac{8}{x^2-4}\)
P=\(\dfrac{3}{x+2}\)+\(\dfrac{2}{x-2}\)-\(\dfrac{8}{(x-2)(x+2)}\)
P= \(\dfrac{3(x-2)}{(x-2)(x+2)}\)+\(\dfrac{2(x+2)}{(x-2)(x+2)}\)-\(\dfrac{8}{(x-2)(x+2)}\)
P= \(\dfrac{3x-6+2x+4-8}{(x-2)(x+2)}\)
P=\(\dfrac{5x-10}{(x-2)(x+2)}\)
P=\(\dfrac{5(x-2)}{(x-2)(x+2)}\)
P=\(\dfrac{5}{x+2}\)
Vậy P=\(\dfrac{5}{x+2}\)

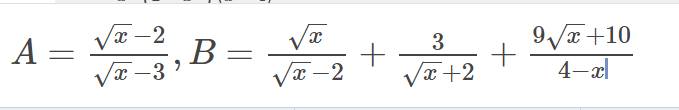
 Với
Với
\(\sqrt{13+4\sqrt{3}}\cdot\sqrt{28+6\sqrt{3}}-5\sqrt{3}\)
\(=\sqrt{\left(2\sqrt{3}+1\right)^2}\cdot\sqrt{\left(3\sqrt{3}+1\right)^2}-5\sqrt{3}\)
\(=\left(2\sqrt{3}+1\right)\left(3\sqrt{3}+1\right)-5\sqrt{3}\)
\(=6\cdot3+2\sqrt{3}+3\sqrt{3}+1-5\sqrt{3}\)
\(=18+1=19\)