Để A là số nguyên thì \(2x-1\in\left\{1;-1;5;-5\right\}\)

hay \(x\in\left\{1;0;3;-2\right\}\)

bn ghi bắng công thức đi

Bài 2:

a: Ta có: \(2\left(5x-8\right)-3\left(4x-5\right)=4\left(3x-4\right)+11\)

\(\Leftrightarrow10x-16-12x+15=12x-16+11\)

\(\Leftrightarrow-14x=-4\)

hay \(x=\dfrac{2}{7}\)

b: Ta có: \(2x\left(6x-2x^2\right)+3x^2\left(x-4\right)=8\)

\(\Leftrightarrow12x^2-4x^3+3x^3-12x^2=8\)

\(\Leftrightarrow x^3=-8\)

hay x=-2

Bài 1: 

a: Ta có: \(I=x\left(y^2-xy^2\right)+y\left(x^2y-xy+x\right)\)

\(=xy^2-x^2y^2+x^2y^2-xy^2+xy\)

\(=xy\)

=1

b: Ta có: \(K=x^2\left(y^2+xy^2+1\right)-\left(x^3+x^2+1\right)\cdot y^2\)

\(=x^2y^2+x^3y^2+x^2-x^3y^2-x^2y^2-y^2\)

\(=x^2-y^2\)

\(=\dfrac{1}{4}-\dfrac{1}{4}=0\)

Để hệ có nghiệm duy nhất thì \(\dfrac{2m}{8}\ne\dfrac{1}{m}\)

=>\(m^2\ne4\)

=>\(m\notin\left\{2;-2\right\}\)

\(\left\{{}\begin{matrix}2mx+y=2\\8x+my=m+2\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}2m^2\cdot x+my=2m\\8x+my=m+2\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}x\left(2m^2-8\right)=m-2\\y=2-2mx\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}x=\dfrac{m-2}{2m^2-8}=\dfrac{1}{2\left(m+2\right)}\\y=2-\dfrac{2m}{2\left(m+2\right)}=2-\dfrac{m}{m+2}=\dfrac{2m+4-m}{m+2}=\dfrac{m+4}{m+2}\end{matrix}\right.\)

4x+3y=7

=>\(\dfrac{4}{2\left(m+2\right)}+\dfrac{3\left(m+4\right)}{m+2}=7\)

=>\(\dfrac{2+3\left(m+4\right)}{m+2}=7\)

=>7(m+2)=2+3m+12

=>7m+14=3m+14

=>4m=0

=>m=0(nhận)

{ x² - [ 6² - ( 8² - 9.7)³ - 7.5]³ - 5.3 }³ = 1

{ x² + [ 36 - (64 - 63)³ - 35]³ - 15}³ = 1

[ x² - ( 36 - 1³ - 35 ) - 15 ]³ = 1

[ x² - ( 36 - 1 - 35 ) - 15]³ = 1

[ x² - ( 35 - 35 ) - 15]³ = 1

[ x² - 0 - 15]³ = 1

( x² - 15 )³ = 1

<=> ( x² - 15)³ = 1³

=> x² - 15 = 1

<=> x² = 16

=> x = 4

`6/x+1/2=2`

`6/x=2-1/2`

`6/x=3/2`

`x=6:3/2`

`x=4`

Vậy `x=4`

\(\left(3x+1\right)^2=9\left(x-2\right)^2\)

\(\Leftrightarrow9x^2+6x+1=9\left(x^2-4x+4\right)\)

\(\Leftrightarrow9x^2+6x+1=9x^2-36x+36\)

\(\Leftrightarrow9x^2+6x+1-9x^2+36x-36=0\)

\(\Leftrightarrow42x-35=0\)

\(\Leftrightarrow42x=35\)

\(\Leftrightarrow x=\dfrac{35}{42}=\dfrac{5}{6}\)

Vậy: \(S=\left\{\dfrac{5}{6}\right\}\)

\(\Delta=\left[-2\left(m-1\right)\right]^2-4\left(m-2\right)\left(m+1\right)\)

    \(=4m^2-8m+4-4\left(m^2+m-2m-2\right)\)

   \(=4m^2-8m+4-4m^2+4m+8\)

   \(=-4m+12\)

Để pt có 2 nghiệm thì \(\Delta>0\)

                                    \(\Leftrightarrow-4m+12>0\)

                                     \(\Leftrightarrow m< 3\)

Theo hệ thức Vi-ét, ta có:\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2m-2}{m+1}\\x_1x_2=\dfrac{m-2}{m+1}\end{matrix}\right.\)

\(\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{7}{4}\)

\(\Leftrightarrow\dfrac{x_1+x_2}{x_1x_2}=\dfrac{7}{4}\)

\(\Leftrightarrow\dfrac{2m-2}{m+1}:\dfrac{m-2}{m+1}=\dfrac{7}{4}\)

\(\Leftrightarrow\dfrac{2m-2}{m-2}=\dfrac{7}{4}\)

\(\Leftrightarrow8m-8=7m-14\)

\(\Leftrightarrow m=-6\left(tm\right)\)

Vậy \(m=-6\)

Khiếp, nhanh thế, nhường iem đi cj :v

\(\Leftrightarrow a\cdot\dfrac{13}{15}=\dfrac{28}{13}:2=\dfrac{14}{13}\)

=>\(a=\dfrac{14}{13}:\dfrac{13}{15}=\dfrac{210}{169}\)

Lời giải:
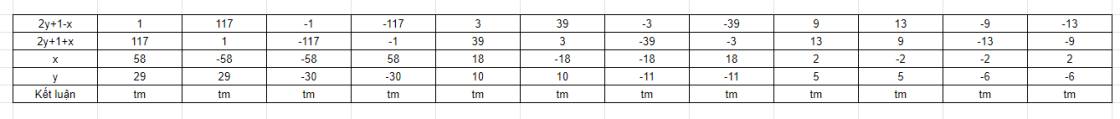
$117=(2y+1)^2-x^2=(2y+1-x)(2y+1+x)$

Vì $x,y$ nguyên nên $2y+1-x, 2y+1+x$ nguyên. Do đó ta có bảng sau: