Tính :
a ) S= 2+4+6+...+2018 ( giải bằng hai cách )
b ) 10 + 102 +103 +...+10100 ( giaỉ bằng hai cách )
c ) \(S=\dfrac{1}{5}+\dfrac{1}{5^2}+\dfrac{1}{5^3}+...+\dfrac{1}{5^{100}}\)( giải bằng hai cách )
d ) \(S=\dfrac{1!}{3!}+\dfrac{2!}{4!}+\dfrac{3!}{5!}+....+\dfrac{2018!}{2020!}\)
biết rằng : n! = \(1\times2\times3\times...\times n\)
VD : 1! = 1
2! = \(1\times2\)
3! = \(1\times2\times3\)
4! \(1\times2\times3\times4\)


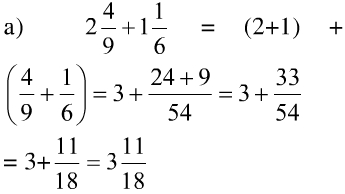
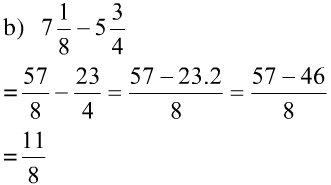
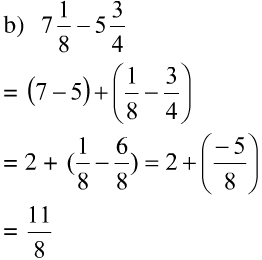
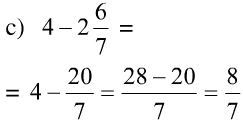
a: Số số hạng là \(\dfrac{2018-2}{2}+1=1009\left(số\right)\)
Tổng là: \(\dfrac{2018+2}{2}\cdot1009=1009\cdot1010=1019090\)
b: \(10S=10^2+10^3+...+10^{101}\)
\(\Rightarrow9S=10^{101}-10\)
hay \(S=\dfrac{10^{101}-10}{9}\)
c: \(5S=1+\dfrac{1}{5}+...+\dfrac{1}{5^{99}}\)
\(\Leftrightarrow4S=1-\dfrac{1}{5^{100}}\)
hay \(S=\dfrac{1}{4}\left(1-\dfrac{1}{5^{100}}\right)\)