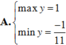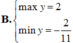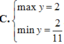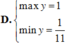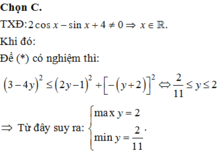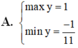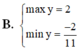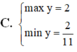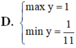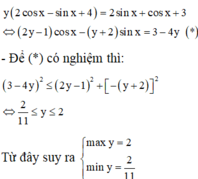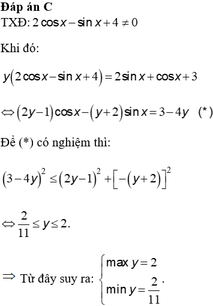a.\(-1\le cosx\le1\Rightarrow-4\le y=3cosx-1\le2\)

b.-1 \(\le sinx\le1\)\(\Rightarrow3\le y=5+2sinx\le7\)  

c.\(\sqrt{3-1}\le\sqrt{3+cos2x}\le\sqrt{3+1}\Rightarrow\sqrt{2}\le y\le2\)

d.\(y=\sqrt{5sinx-1}+2\le\sqrt{5.1-1}+2=4\)

\(y=\sqrt{5sinx-1}+2\ge2\) . " = " \(\Leftrightarrow sinx=\dfrac{1}{5}\Leftrightarrow\left[{}\begin{matrix}x=arcsin\left(\dfrac{1}{5}\right)+2k\pi\\x=\pi-arcsin\left(\dfrac{1}{5}\right)+2k\pi\end{matrix}\right.\)  ( k thuộc Z ) 

Có: `-1 <= sin x <= 1`

`<=>-2 <= sin x-1 <= 0=>sin x-1 <= 0`

Để hàm số đã cho xác định `<=>sin x-1 >= 0`    Mà `sin x - 1 <= 0`

         `=>sin x -1=0<=>x=\pi/2+k2\pi`   `(k in ZZ)`

  `=>TXĐ: D=\pi/2 +k2\pi`   `(k in ZZ)`.

Ta có:

`@-1 <= sin x <= 1`

  `<=>0 <= 1+sin x <= 2=>1+sin x >= 0`

`@-1 <= cos x <= 1`

`<=>1 >= -cos x >= -1`

`<=>2 >= 1-cos x >= 0=>1-cos x >= 0`

Hàm số xác định `<=>[1+sin x]/[1-cos x] >= 0`

     `<=>{(1+sin x >= 0(L Đ)),(1-cos x > 0):}<=>1-cos x ne 0<=>x ne k2\pi (k in ZZ)`

   `=>TXĐ: D=R\\{k2\pi| k in ZZ}`.

\(y=\left|2sin^2x-sinx-1\right|-2sinx\)

Đặt \(sinx=t\in\left[-1;1\right]\)

\(\Rightarrow y=f\left(t\right)=\left|2t^2-t-1\right|-2t\)

BBT cho \(f\left(t\right)\) trên \(\left[-1;1\right]\):

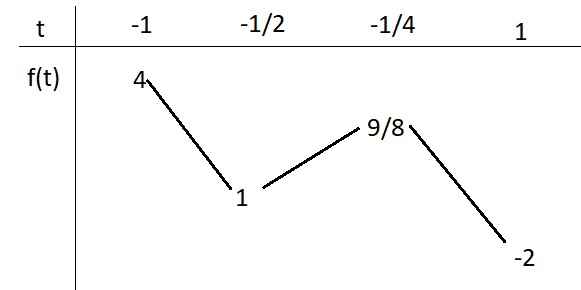
Từ BBT ta thấy \(y_{max}=4\) khi \(sinx=-1\); \(y_{min}=-2\) khi \(sinx=1\)

Đáp án C

TXĐ:

- Khi đó: