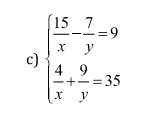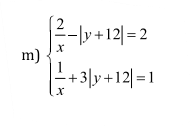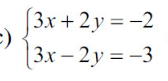b: \(\left\{{}\begin{matrix}3x-2y=4\\2x+y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x-2y=4\\4x+2y=10\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}-x=-6\\2x+y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=6\\y=5-2x=5-12=-7\end{matrix}\right.\)

\(\left\{{}\begin{matrix}x+3y=-4\\5x-8y=3\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-4-3y\\5\left(-4-3y\right)-8y=3\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-4-3y\\-20-15y-8y=3\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-4-3y\\-20-23y=3\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-4-3\left(-1\right)\\y=-1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=-1\end{matrix}\right.\)

\(\begin{cases} 4x+5y=3\\x+3y=-1 \end{cases} <=>\begin{cases} 4(-1-3y)+5y=3\\x=-1-3y \end{cases} \\<=>\begin{cases} -7y=7\\x=-1-3y \end{cases} <=>\begin{cases} y=-1\\x=-1-3.(-1) \end{cases} \\<=>\begin{cases} y=-1\\x=2 \end{cases}\)

\(\left\{{}\begin{matrix}x+y=3\\x+2y=5\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=3-y\\3-y+2y=5\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=3-y\\3+y=5\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=3-2\\y=2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)

\(\left\{{}\begin{matrix}x+y=3\\x+2y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3-y\\3-y+2y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)

Vậy hpt có nghiệm (x;y) = (1;2) 

\(\left(2\right)\Leftrightarrow\left|x-1\right|=3-3y\)

Thay vào \(\left(1\right)\Leftrightarrow3-3y+\left|y-2\right|=1\Leftrightarrow\left|y-2\right|=3y-2\)

\(\Leftrightarrow\left[{}\begin{matrix}y-2=3y-2\left(y\ge2\right)\\2-y=3y-2\left(y< 2\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}y=0\left(tkm\right)\\y=1\left(tm\right)\end{matrix}\right.\)

Với \(y=1\Leftrightarrow\left|x-1\right|=3-3=0\Leftrightarrow x=1\)

Vậy \(\left(x;y\right)=\left(1;1\right)\)

\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{135}{x}-\dfrac{63}{y}=81\\\dfrac{28}{x}+\dfrac{63}{y}=245\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{163}{x}=326\\\dfrac{4}{x}+\dfrac{9}{y}=35\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{2}\\\dfrac{9}{y}=35-\dfrac{4}{x}=35-8=27\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{2}\\y=\dfrac{1}{3}\end{matrix}\right.\)

\(ĐK:x\le12\\ PT\Leftrightarrow\left(\sqrt[3]{x+24}-3\right)+\left(\sqrt{12-x}-3\right)=0\\ \Leftrightarrow\dfrac{x-3}{\sqrt[3]{\left(x+24\right)^2}+3\sqrt[3]{x+24}+9}-\dfrac{x-3}{\sqrt{12-x}+3}=0\\ \Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\\dfrac{1}{\sqrt[3]{\left(x+24\right)^2}+3\sqrt[3]{x+24}+9}=\dfrac{1}{\sqrt{12-x}+3}\left(1\right)\end{matrix}\right.\)

\(\left(1\right)\Leftrightarrow\sqrt[3]{\left(x+24\right)^2}+3\sqrt[3]{x+24}+9=\sqrt{12-x}+3\\ \Leftrightarrow\sqrt[3]{x+24}\left(\sqrt[3]{x+24}+3\right)+6-\sqrt{12-x}=0\\ \Leftrightarrow\dfrac{\left(x+24\right)\left(\sqrt[3]{x+24}+3\right)}{\sqrt[3]{\left(x+24\right)^2}}+\dfrac{x+24}{6+\sqrt{12-x}}=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-24\left(tm\right)\\\dfrac{\sqrt[3]{x+24}+3}{\sqrt[3]{\left(x+24\right)^2}}=\dfrac{-1}{6+\sqrt{12-x}}\left(2\right)\end{matrix}\right.\)

\(\left(2\right)\Leftrightarrow\dfrac{\sqrt[3]{x+24}+3}{\sqrt[3]{x+24}}+\dfrac{1}{\sqrt[3]{x+24}}+\dfrac{1}{6+\sqrt{12-x}}-\dfrac{1}{\sqrt[3]{x+24}}=0\\ \Leftrightarrow\dfrac{\sqrt[3]{x+24}+4}{\sqrt[3]{x+24}}+\dfrac{\sqrt[3]{x+24}+4-10-\sqrt{12-x}}{\sqrt[3]{x+24}\left(6+\sqrt{12-x}\right)}=0\\ \Leftrightarrow\dfrac{x+88}{\sqrt[3]{x+24}\left(\sqrt[3]{\left(x+24\right)^2}-4\sqrt[3]{x+24}+16\right)}+\dfrac{\sqrt[3]{x+24}+4-10-\sqrt{12-x}}{\sqrt[3]{x+24}\left(6+\sqrt{12-x}\right)}=0\)

Xét \(\sqrt[3]{x+24}+4-10-\sqrt{12-x}=\dfrac{x+88}{\sqrt[3]{\left(x+24\right)^2}-4\sqrt[3]{x+24}+16}-\dfrac{x+88}{10+\sqrt{12-x}}=0\)

\(=\left(x+88\right)\left(\dfrac{1}{\sqrt[3]{\left(x+24\right)^2}-4\sqrt[3]{x+24}+16}-\dfrac{1}{10+\sqrt{12-x}}\right)\)

Thay vào PT (2) ta đặt đc nhân tử chung là \(x+88\)

Và ngoặc lớn còn lại vô nghiệm

\(\Leftrightarrow x+88=0\Leftrightarrow x=-88\left(tm\right)\)

Vậy PT có nghiệm \(x\in\left\{-88;-24;3\right\}\)

P/s mình thấy giải theo PP đặt ẩn phụ dễ hơn á ;-;

\(\left\{{}\begin{matrix}3x+2y=-2\\3x-2y=-3\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}3x+2y=-2\\3x+2y-3x+2y=-2+3\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}3x+2y=-2\\4y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}3x+2.\dfrac{1}{4}=-2\\y=\dfrac{1}{4}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{5}{6}\\x=\dfrac{1}{4}\end{matrix}\right.\)