Cho y=f(x) nghịch biến trên R và thỏa [f(x)-x].f(x) = x^6 + 3x^4 + 2x^2 . tìm GTLN và GTNN của f(x) trên [1,2]
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Những câu hỏi liên quan

CM
16 tháng 11 2019
Đáp án D
Ta có Đáp án D
Ta có y’ = –f’(1 – x) + 2018 = –[1–(1–x)][(1–x)+2]g(1–x) – 2018 + 2018
= –x(3–x)g(1–x)
Suy ra 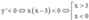 (vì g(1–x) < 0,
∀
x
∈
R
)
(vì g(1–x) < 0,
∀
x
∈
R
)
Vậy hàm số đã cho nghịch biến trên khoảng 3 ; + ∞


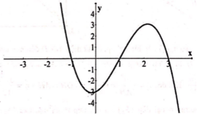
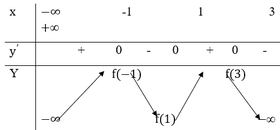

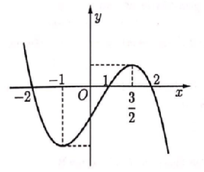
Do \(f\left(x\right)\) nghịch biến \(\Rightarrow\min\limits_{\left[1;2\right]}f\left(x\right)=f\left(2\right)\); \(\max\limits_{\left[1;2\right]}=f\left(1\right)\)
Thay \(x=1\) vào ta được:
\(f^2\left(1\right)-f\left(1\right)=6\Rightarrow f^2\left(1\right)-f\left(1\right)-6=0\Rightarrow\left[{}\begin{matrix}f\left(1\right)=3\\f\left(1\right)=-2\end{matrix}\right.\)
Thay \(x=2\) vào ta được:
\(f^2\left(2\right)-2f\left(2\right)-120=0\Rightarrow\left[{}\begin{matrix}f\left(2\right)=12>f\left(1\right)\left(l\right)\\f\left(2\right)=-10\end{matrix}\right.\)
\(\Rightarrow\min\limits_{\left[1;2\right]}f\left(x\right)=-10\)
Đạo hàm 2 vế giả thiết:
\(\left[f'\left(x\right)-1\right]f\left(x\right)+f'\left(x\right)\left[f\left(x\right)-x\right]=6x^5+12x^3+4x\)
- Nếu \(f\left(1\right)=3\) thay \(x=1\) vào biểu thức trên ta được:
\(\left[f'\left(1\right)-1\right].3+f'\left(1\right).\left[3-1\right]=22\) \(\Rightarrow f'\left(1\right)=5>0\) (vô lý do \(f\left(x\right)\) nghịch biến trên R nên \(f'\left(x\right)< 0\) \(\forall x\))
\(\Rightarrow f\left(1\right)=-2\Rightarrow\max\limits_{\left[1;2\right]}f\left(x\right)=-2\)