Chỉ ý kiến của mk thôi

chưa chắc đúng

Tham khảo nhé

Cau a va b dat cot tim so du .Vi la phep chia het nen du bang 0.Cau c thi da thuc se chia het cho tich (x+3)(x-3) lam tuong tu hai cau a va b

trình bày ra bố ạ!

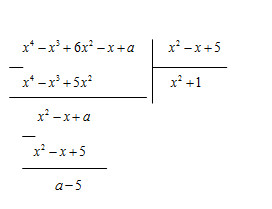
Vậy a = 5 thì đa thức x^4 - x^3 + 6x^2 - x +a chia hết cho đa thức x^2 - x + 5

Vì \(f\left(x\right)⋮x-2;f\left(x\right):x^2-1\) dư 1\(\Rightarrow\left\{{}\begin{matrix}f\left(x\right)=g\left(x\right)\cdot\left(x-2\right)\\f\left(x\right)=q\left(x\right)\left(x^2-1\right)+x=q\left(x\right)\left(x-1\right)\left(x+1\right)+x\end{matrix}\right.\) 

\(\Rightarrow\left\{{}\begin{matrix}f\left(2\right)=0\\f\left(1\right)=1\\f\left(-1\right)=-1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}32+4a+2b+c=0\\2+a+b+c=1\\2+a-b+c=-1\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}4a+2b+c=-32\left(1\right)\\a+b+c=-1\left(2\right)\\a-b+c=-3\left(3\right)\end{matrix}\right.\)

 Trừ từng vế của (2) cho (3) ta được:

\(\Rightarrow2b=2\Rightarrow b=1\)

Thay b=1 vào lần lượt (1) ,(2),(3) ta được:

\(\Rightarrow\left\{{}\begin{matrix}4a+2+c=-32\\a+1+c=-1\\a-1+c=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4a+c=-34\\a+c=-2\\a+c=-2\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}4a+c=-34\left(4\right)\\a+c=-2\left(5\right)\end{matrix}\right.\)

Trừ từng vế của (4) cho (5) ta được:

\(\Rightarrow3a=-32\Rightarrow a=-\dfrac{32}{3}\Rightarrow c=-2+\dfrac{32}{3}=\dfrac{26}{3}\) Vậy...

Đa thức x² - 3x + 2 có nghiệm \(\Leftrightarrow\)x² - 3x + 2 = 0

\(\Leftrightarrow x^2-2x-x+2=0\)

\(\Leftrightarrow x\left(x-2\right)-\left(x-2\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-2\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}x-1=0\\x-2=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=1\\x=2\end{cases}}\)

1 và 2 là hai nghiệm của đa thức x² - 3x + 2

Để f(x) = x⁴ + ax³ + bx - 1  chia hết cho x² - 3x + 2 thì 1 và 2 cũng là hai nghiệm của đa thức f(x) = x⁴ + ax³ + bx - 1

Nếu x = 1 thì \(1+a+b-1=0\Leftrightarrow a+b=0\)(1

Nếu x = 2 thì \(16+8a+2b-1=0\Leftrightarrow4a+b=\frac{-15}{2}\)(2)

Lấy (2) - (1), ta được: \(3a=\frac{-15}{2}\Leftrightarrow a=\frac{-5}{2}\)

\(\Rightarrow b=0+\frac{5}{2}=\frac{5}{2}\)

Vậy \(a=\frac{-5}{2};b=\frac{5}{2}\)