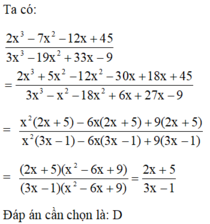
a, Để phân thức trên có nghĩa thì:

      \(3x^3-19x^2+33x-9\ne0\)

 \(\Rightarrow3x^3-9x^2-10x^2+30x+3x-9\ne0\)

\(\Rightarrow3x^2\left(x-3\right)-10x\left(x-3\right)+3\left(x-3\right)\ne0\)

\(\Rightarrow\left(x-3\right)\left(3x^2-10x+3\right)\ne0\)

\(\Rightarrow\left(x-3\right).\left[3x^2-9x-x+3\right]\ne0\)

\(\Rightarrow\left(x-3\right)\left[3x\left(x-3\right)-\left(x-3\right)\right]\ne0\)

\(\Rightarrow\left(x-3\right)^2.\left(3x-1\right)\ne0\)

\(\Rightarrow\hept{\begin{cases}x-3\ne0\\3x-1\ne0\end{cases}\Rightarrow\hept{\begin{cases}x\ne3\\x\ne\frac{1}{3}\end{cases}}}\)

Xét tử thức ta có

2x³-7x²-12x+45

= 2x³+5x²-12x²-30x+18x+45

= x²(2x+5)-6x(2x+5)+9(2x+5)

= (2x+5)(x²-6x+9)

= (2x+5)(x-3)² (1)

Xét mẫu thức ta có

3x³-19x²+33x-9

= 3x³-x²-18x²+6x+27x-9

= x²(3x-1)-6x(3x-1)+9(3x-1)

= (3x-1)(x²-6x+9)

= (3x-1)(x-3)² (2)

Thay (1) và (2) vào A ta được\(A=\frac{\left(2x+5\right)\left(x-3\right)^2}{\left(3x-1\right)\left(x-3\right)^2}=\frac{2x+5}{3x-1}\)

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{\left(2x+5\right)\left(x-3\right)^2}{\left(3x-1\right)\left(x-3\right)^2}=\frac{2x+5}{3x-1}\)

Ta có tử bằng:2x³-7x²-12x+45

                    =(2x³-6x²)-(x²-3x)-(15x-45)

                    =2x²(x-3)-x(x-3)-15(x-3)

                    =(x-3)(2x²-x-15)

                    =(x-3)(2x²-6x+5x-15)

                   =(x-3)²(2x+5)                   (1)

Ta có mẫu bằng:3x³-19x²+33x-9

                        =(3x³-x²)-(19x²-6x)+(27x-9)

                        =x²(3x-1)-6x(3x-1)+9(3x-1)

                        =(3x-1)(x²-6x+9)

                        =(3x-1)(x-3)²                (2)

Thay (1) và (2) vào phân thức ,ta có:

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{\left(x-3\right)^2\left(2x+5\right)}{\left(x-3\right)^2\left(3x-1\right)}=\frac{2x+5}{3x-1}\)

\(a)\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{(x-3)^2(2x+5)}{(3x-1)(x-3)^2}(ĐK:x\ne3,x\ne\frac{1}{3})\)

                                                \(=\frac{2x+5}{3x-1}\)

Còn bài b bạn tự làm nhé

Điều kiện: \(x\ne\left\{-1;-2;-5\right\}\)

\(\frac{x^3+x^2-4x-4}{x^3+8x^2+17x+10}=\frac{x^2\left(x+1\right)-4\left(x+1\right)}{x^2\left(x+1\right)+7x\left(x+1\right)+10\left(x+1\right)}\)

\(=\frac{\left(x+1\right)\left(x^2-4\right)}{\left(x+1\right)\left(x^2+7x+10\right)}\)

\(=\frac{\left(x+1\right)\left(x-2\right)\left(x+2\right)}{\left(x+1\right)\left[x\left(x+2\right)+5\left(x+2\right)\right]}\)

\(=\frac{\left(x+1\right)\left(x-2\right)\left(x+2\right)}{\left(x+1\right)\left(x+2\right)\left(x+5\right)}=\frac{x-2}{x+5}\)

Điều kiện: \(x\ne\left\{3;\frac{1}{3}\right\}\)

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{2x^3-6x^2-x^2+3x-15x+45}{3x^3-9x^2-10x^2+30x+3x-9}\)

\(=\frac{2x^2\left(x-3\right)-x\left(x-3\right)-15\left(x-3\right)}{3x^2\left(x-3\right)-10x\left(x-3\right)+3\left(x-3\right)}\)

\(=\frac{\left(x-3\right)\left(2x^2-x-15\right)}{\left(x-3\right)\left(3x^2-10x+3\right)}\)

\(=\frac{2x^2-x-15}{3x^2-10x+3}=\frac{2x\left(x-3\right)+5\left(x-3\right)}{3x\left(x-3\right)-\left(x-3\right)}\)

\(=\frac{\left(2x+5\right)\left(x-3\right)}{\left(3x-1\right)\left(x-3\right)}=\frac{2x+5}{3x-1}\)

\(B=\dfrac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}\)

\(=\dfrac{2x^3+5x^2-12x^2-30x+18x+45}{3x^3-x^2-18x^2+6x+27x-9}\)

\(=\dfrac{\left(2x^3+5x^2\right)-\left(12x^2+30x\right)+\left(18x+45\right)}{\left(3x^3-x^2\right)-\left(18x^2-6x\right)+\left(27x-9\right)}\)

\(=\dfrac{x^2\left(2x+5\right)-6x\left(2x+5\right)+9\left(2x+5\right)}{x^2\left(3x-1\right)-6x\left(3x-1\right)+9\left(3x-1\right)}\)

\(=\dfrac{\left(2x+5\right)\left(x^2-6x+9\right)}{\left(3x-1\right)\left(x^2-6x+9\right)}\)

\(=\dfrac{\left(2x+5\right)\left(x-3\right)^2}{\left(3x-1\right)\left(x-3\right)^2}\)

ĐKXĐ : \(\left\{{}\begin{matrix}3x-1\ne0\\x-3\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{1}{3}\\x\ne3\end{matrix}\right.\)

\(a,B=\dfrac{\left(2x+5\right)\left(x-3\right)^2}{\left(3x-1\right)\left(x-3\right)^2}=\dfrac{2x+5}{3x-1}\)

b,Để \(B>0\)

\(\Leftrightarrow\dfrac{2x+5}{3x-1}>0\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}2x+5>0\\3x-1>0\end{matrix}\right.\\\left\{{}\begin{matrix}2x+5< 0\\3x-1< 0\end{matrix}\right.\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x>-\dfrac{5}{2}\\x>\dfrac{1}{3}\end{matrix}\right.\\\left\{{}\begin{matrix}x< -\dfrac{5}{2}\\x< \dfrac{1}{3}\end{matrix}\right.\end{matrix}\right.\)

Vậy \(\left[{}\begin{matrix}x>\dfrac{1}{3}\\x< -\dfrac{5}{2}\end{matrix}\right.\) thì B > 0

a) ĐKXĐ:\(x\ne\dfrac{1}{3};x\ne3\)

\(B=\dfrac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}\)

\(B=\dfrac{\left(2x^3-12x^2+18x\right)+\left(5x^2-30x+45\right)}{\left(3x^3-18x^2+27x\right)-\left(x^2-6x+9\right)}\)

\(B=\dfrac{2x\left(x^2-6x+9\right)+5\left(x^2-6x+9\right)}{3x\left(x^2-6x+9\right)-\left(x^2-6x+9\right)}\)

\(B=\dfrac{\left(2x+5\right)\left(x^2-6x+9\right)}{\left(3x-1\right)\left(x^2-6x+9\right)}\)

\(B=\dfrac{2x+5}{3x-1}\)

b) Để \(B>0\Leftrightarrow\dfrac{2x+5}{3x-1}>0\Leftrightarrow2x+5\)và \(3x-1\) cùng dấu

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}2x+5>0\\3x-1>0\end{matrix}\right.\\\left\{{}\begin{matrix}2x+5< 0\\3x-1< 0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x>\dfrac{-5}{2}\\x>\dfrac{1}{3}\end{matrix}\right.\\\left\{{}\begin{matrix}x< \dfrac{-5}{2}\\x< \dfrac{1}{3}\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x>\dfrac{1}{3}\\x< -\dfrac{5}{2}\end{matrix}\right.\)

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}\)

\(=\frac{\left(x-\frac{2}{5}\right)\left(x+3\right)}{\left(x+\frac{1}{3}\right)\left(x+3\right)}\)

\(=\frac{x-\frac{2}{5}}{x+\frac{1}{3}}\)

=\(\frac{2x^3-6x^2-x^2+3x-15x+45}{3x^3-9x^2-10x^2+30x+3x-9}\)

=\(\frac{2x^2\left(x-3\right)-x\left(x-3\right)-15\left(x-3\right)}{3x^2\left(x-3\right)-10x\left(x-3\right)+3\left(x-3\right)}\)

=\(\frac{\left(x-3\right)\left(2x^2-x-15\right)}{\left(x-3\right)\left(3x^2-10x+3\right)}\)

=\(\frac{2x^2-6x+5x-15}{3x^2-9x-x+3}\)

=\(\frac{2x\left(x-3\right)+5\left(x-3\right)}{3x\left(x-3\right)-\left(x-3\right)}\)

=\(\frac{2x+5}{3x-1}\)

2x³ - 7x² - 12x + 45 = 2x³ - 6x² - x² + 3x - 15x + 45

                          =  2x²(x - 3) - x(x - 3) - 15(x - 3)

                          = (x - 3)(2x² - x - 15)

                          = (x - 3)(2x² - 6x + 5x - 15)

                          = (x -  3)((2x(x - 3) + 5(x - 3))

                         =  (x - 3)²(2x + 5)

3x³ - 19x² +33x - 9 = 3x³ -9x² -10x² + 30x +3x - 9

                              = 3x²(x - 3) - 10x(x - 3) + 3(x - 3)

                             = (x - 3)(3x² - 10x + 3)

                            = (x - 3)(3x² -9x - x +3)

                            = (x - 3)((3x(x-3) - (x - 3))

                           =(x - 3)²(3x - 1)

Lời giải:

Ta có:

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{\text{TS}}{\text{MS}}\)

Xét \(\text{TS}=2x^2(x-3)-x(x-3)-15(x-3)\)

\(=(x-3)(2x^2-x-15)=(x-3)[2x(x-3)+5(x-3)]\)

\(=(x-3)(x-3)(2x+5)=(x-3)^2(2x+5)\)

Xét \(\text{MS}=3x^2(x-3)-10x(x-3)+3(x-3)\)

\(=(x-3)(3x^2-10x+3)=(x-3)[3x(x-3)-(x-3)]\)

\(=(x-3)(x-3)(3x-1)=(x-3)^2(3x-1)\)

Do đó:

\(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{(x-3)^2(2x+5)}{(x-3)^2(3x-1)}=\frac{2x+5}{3x-1}\)

a/ \(\dfrac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\dfrac{2x^3-12x^2+18x+5x^2-30x+45}{3x^3-18x^2+27x-x^2+6x-9}\)

\(=\dfrac{2x\left(x^2-6x+9\right)+5\left(x^2-6x+9\right)}{3x\left(x^2-6x+9\right)-\left(x^2-6x+9\right)}=\dfrac{\left(2x+5\right)\left(x^2-6x+9\right)}{\left(3x-1\right)\left(x^2-6x+9\right)}\)

\(=\dfrac{2x+5}{3x-1}\)

b/ \(\dfrac{x^3+x^2-4x-4}{x^3+8x^2+17x+10}=\dfrac{x^3+3x^2+2x-2x^2-6x-4}{x^3+3x^2+2x+5x^2+15x+10}\)

\(=\dfrac{x\left(x^2+3x+2\right)-2\left(x^2+3x+2\right)}{x\left(x^2+3x+2\right)+5\left(x^2+3x+2\right)}=\dfrac{\left(x-2\right)\left(x^2+3x+2\right)}{\left(x+5\right)\left(x^2+3x+2\right)}\)

\(=\dfrac{x-2}{x+5}\)

Lời giải:

ĐKXĐ:.........

a) \(\frac{2x^3-7x^2-12x+45}{3x^3-19x^2+33x-9}=\frac{2x^3-6x^2-(x^2-3x)-(15x-45)}{3x^3-9x^2-(10x^2-30x)+(3x-9)}\)

\(=\frac{2x^2(x-3)-x(x-3)-15(x-3)}{3x^2(x-3)-10x(x-3)+3(x-3)}=\frac{(x-3)(2x^2-x-15)}{(x-3)(3x^2-10x+3)}\)

\(=\frac{(x-3)[2x(x-3)+5(x-3)]}{(x-3)[3x(x-3)-(x-3)]}=\frac{(x-3)(x-3)(2x+5)}{(x-3)(x-3)(3x-1)}=\frac{2x+5}{3x-1}\)

b)

\(\frac{x^3+x^2-4x-4}{x^3+8x^2+17x+10}=\frac{x^2(x+1)-4(x+1)}{x^3+x^2+7x^2+7x+10x+10}\)

\(=\frac{(x+1)(x^2-4)}{x^2(x+1)+7x(x+1)+10(x+1)}=\frac{(x+1)(x-2)(x+2)}{(x+1)(x^2+7x+10)}\)

\(=\frac{(x-2)(x+2)}{x^2+7x+10}=\frac{(x-2)(x+2)}{x(x+2)+5(x+2)}=\frac{(x-2)(x+2)}{(x+2)(x+5)}=\frac{x-2}{x+5}\)