a,M=2^0-2^1+2^2-2^3+2^4-2^5+.....+2^2012

2M=2^1-2^2+2^3-2^4+2^5-2^5+......-2^2012+2^2013

3M=2^0+2^2013

M=(2^0+2^2013)÷3

Vậy.......

b,N=3-3^2+3^3-3^4+3^5-3^6+.....+3^2011-3^2012

3N=3^2-3^3+3^4-3^5+3^6-3^7+......+3^2012-3^2013

4N=3-3^2013

N=(3-3^2013)÷4

Vậy........

K tao nhé ko lên lớp tao đánh m😈😈😈

Bt dễ thế mà ko làm dc😂😂😂😂😂

`Answer:`

`a)`

`A=5(x+1)^2-3(x-3)^2-4(x^2-4)`

`=>A=5(x^2+2x+1)-3(x^2-6x+9)-4x^2+16`

`=>A=5x^2+10x+5-3x^2+18x-27-4x^2+16`

`=>A=(5x^2-3x^2-4x^2)+(10x+18x)+(5-27+16)`

`=>A=-2x^2+28x-6`

`b)`

`B=5(x+1)^2-3(x-3)^2-4(x+2)(x-2)`

`=2x(3x+5)-3(3x+5)-2x(x^2-4x+4)-[(2x)^2-3^2]`

`=6x^2+10x-9x-15-2x^3+8x^2-8x-4x^2+9`

`=(6x^2-4x^2+8x^2)-2x^3+(10x-9x-8x)+(-15+9)`

Thay `x=-7` vào ta được:

`B=10(-7)^2-2(-7)^3-7(-7)-6`

`=>B=10.49-2(-343)+49-6`

`=>B=490+686+49-6`

`=>B=1219`

Bài 1: 

a: \(A=\dfrac{x^2-3+x+3}{\left(x-3\right)\left(x+3\right)}\cdot\dfrac{x+3}{x}=\dfrac{x\left(x+1\right)}{x\left(x-3\right)}=\dfrac{x+1}{x-3}\)

b: Để A=3 thì 3x-9=x+1

=>2x=10

hay x=5

Bài 2: 

a: \(A=\dfrac{x+x-2-2x-4}{\left(x-2\right)\left(x+2\right)}:\dfrac{x+2-x}{x+2}\)

\(=\dfrac{-6}{x-2}\cdot\dfrac{1}{2}=\dfrac{-3}{x-2}\)

b: Để A nguyên thì \(x-2\in\left\{1;-1;3;-3\right\}\)

hay \(x\in\left\{3;1;5;-1\right\}\)

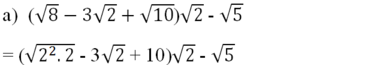
= (2√2 - 3√2 + 10)√2 - √5

= 2.(√2)2 - 3.(√2)2 + √10.√2 - √5

= 4 - 6 + √20 - √5 = -2 + 2√5 - √5

= -2 + √5

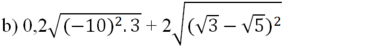
= 0,2.10.√3 + 2|√3 - √5|

s

= 2√3 + 2(√5 - √3)

= 2√3 + 2√5 - 2√3 = 2√5

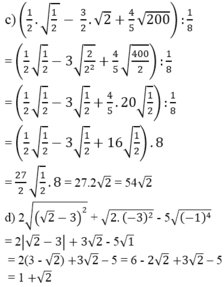
A = (2x - 1)(x + 2) - 3x² + (x - 1)²

= 2x² + 4x - x - 2 - 3x² + x² - 2x + 1

= (2x² - 3x² + x²) + (4x - x - 2x) + (-2 + 1)

= x - 1

B = (x - 2)(x² + 2x + 4) - (x³ + x²) - (3 - x)(3 + x)

= x³ - 8 - x³ - x² - 9 + x²

= (x³ - x³) + (-x² + x²) + (-8 - 9)

= -17

A = (2x - 1)(x + 2) - 3x² + (x - 1)²

= 2x² + 4x - x - 2 - 3x² + x² - 2x + 1

= (2x² - 3x² + x²) + (4x - x - 2x) + (-2 + 1)

= x - 1

B = (x - 2)(x² + 2x + 4) - (x³ + x²) - (3 - x)(3 + x)

= x³ - 8 - x³ - x² - 9 + x²

= (x³ - x³) + (-x² + x²) + (-8 - 9)

= -17

kết quả đây

chúc bạn học tốt

a: \(=\dfrac{6+4\sqrt{2}}{\sqrt{2}+2+\sqrt{2}}+\dfrac{6-4\sqrt{2}}{\sqrt{2}-2+\sqrt{2}}\)

\(=\dfrac{6+4\sqrt{2}}{2+2\sqrt{2}}+\dfrac{6-4\sqrt{2}}{2\sqrt{2}-2}\)

\(=\dfrac{3+2\sqrt{2}}{\sqrt{2}+1}+\dfrac{3-2\sqrt{2}}{\sqrt{2}-1}\)

=căn 2+1+căn 2-1=2căn 2

b: \(=\dfrac{\sqrt{3}+\sqrt{3+\sqrt{3}}+\sqrt{3}-\sqrt{3+\sqrt{3}}}{1-\sqrt{3}-1}=\dfrac{-2\sqrt{3}}{\sqrt{3}}=-2\)

bạn ơi cho mình hỏi câu b chi tiết hơn đước ko ạ

mình chưa hiểu lắm

       A =          1 +   \(\dfrac{1}{3^2}\) + \(\dfrac{1}{3^3}\) +.......+\(\dfrac{1}{3^{n-1}}\) + \(\dfrac{1}{3^n}\)  

3\(\times\) A  =  3  +  \(\dfrac{1}{3}\) +  \(\dfrac{1}{3^2}\) + \(\dfrac{1}{3^3}\)+........+ \(\dfrac{1}{3^{n-1}}\)

3A - A =  3 + \(\dfrac{1}{3}\) - 1 - \(\dfrac{1}{3^n}\) 

    2A  = \(\dfrac{7}{3}\) - \(\dfrac{1}{3^n}\)

      A  = ( \(\dfrac{7}{3}\) - \(\dfrac{1}{3^n}\)): 2

     A =   \(\dfrac{7.3^{n-1}-1}{3^n}\) : 2

     A = \(\dfrac{7.3^{n-1}-1}{2.3^n}\)

   B   =      \(\dfrac{1}{2}\) - \(\dfrac{1}{2^2}\) + \(\dfrac{1}{2^3}\) - \(\dfrac{1}{2^4}\)+......+\(\dfrac{1}{2^{99}}\) - \(\dfrac{1}{2^{100}}\)

2B    =  2 - \(\dfrac{1}{2}\) + \(\dfrac{1}{2^2}\) -  \(\dfrac{1}{2^3}\)+ \(\dfrac{1}{2^4}\)-.......-\(\dfrac{1}{2^{99}}\)

2B + B = 2 - \(\dfrac{1}{2^{100}}\)

  3B     =  2 - \(\dfrac{1}{2^{100}}\)

    B     =   ( 2 - \(\dfrac{1}{2^{100}}\)): 3

    B     =     \(\dfrac{2.2^{100}-1}{2^{100}}\) : 3

    B     = \(\dfrac{2^{101}-1}{3.2^{100}}\)

a) \(\left(3x-2\right)^2-\left(2x+3\right)\left(2x-3\right)\)

\(=9x^2-12x+4-4x^2+9\)

\(=5x^2-12x+13\)

b) \(3x\left(5x-2\right)-\left(2x^2-1\right)\left(2-x\right)\)

\(=15x^2-6x-\left(4x^2-2x^3-2+x\right)\)

\(=15x^2-6x-4x^2+2x^3+2-x\)

\(=11x^2-7x+2x^3+2\)

b,     B        =                       \(\dfrac{1}{2}\) - \(\dfrac{1}{2^2}\)  + \(\dfrac{1}{2^3}\) -   \(\dfrac{1}{2^4}\)+.....+ \(\dfrac{1}{2^{99}}\) - \(\dfrac{1}{2^{100}}\)

2 \(\times\)  B       =                 1 + \(\dfrac{1}{2}\) + \(\dfrac{1}{2^2}\) -  \(\dfrac{1}{2^3}\) + \(\dfrac{1}{2^4}\)-.......-\(\dfrac{1}{2^{99}}\)

2 \(\times\) B + B  =                1  -  \(\dfrac{1}{2^{100}}\)

3B             =              ( 1 - \(\dfrac{1}{2^{100}}\)) 

             B =               ( 1 - \(\dfrac{1}{2^{100}}\)) : 3

       A              =          1 + \(\dfrac{1}{3}\) + \(\dfrac{1}{3^2}\)+ \(\dfrac{1}{3^3}\)+......+ \(\dfrac{1}{3^{n-1}}\) + \(\dfrac{1}{3^n}\) 

A\(\times\)  3             =   3 +  1 + \(\dfrac{1}{3}\) +  \(\dfrac{1}{3^2}\) + \(\dfrac{1}{3^2}\)+....+  \(\dfrac{1}{3^{n-1}}\) 

A \(\times\) 3 - A        = 3 - \(\dfrac{1}{3^n}\)

       2A           = 3  - \(\dfrac{1}{3^n}\)

         A           = ( 3 - \(\dfrac{1}{3^n}\)) : 2

1/2  x 2 (6x – 3) – x(  x 2 + 1/2) + 1/2.(x + 4)

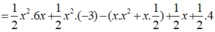
= (3 x 3  – 3/2. x 2 ) – ( x 3  + 1/2.x) + (1/2.x + 2)

= 3 x 3  - 3/2  x 2  –  x 3  - 1/2 x + 1/2 x + 2

= ( 3 x 3  –  x 3  ) - 3/2.  x 2  – (1/2 x - 1/2 x) + 2

= 2 x 3  - 3/2  x 2  + 2