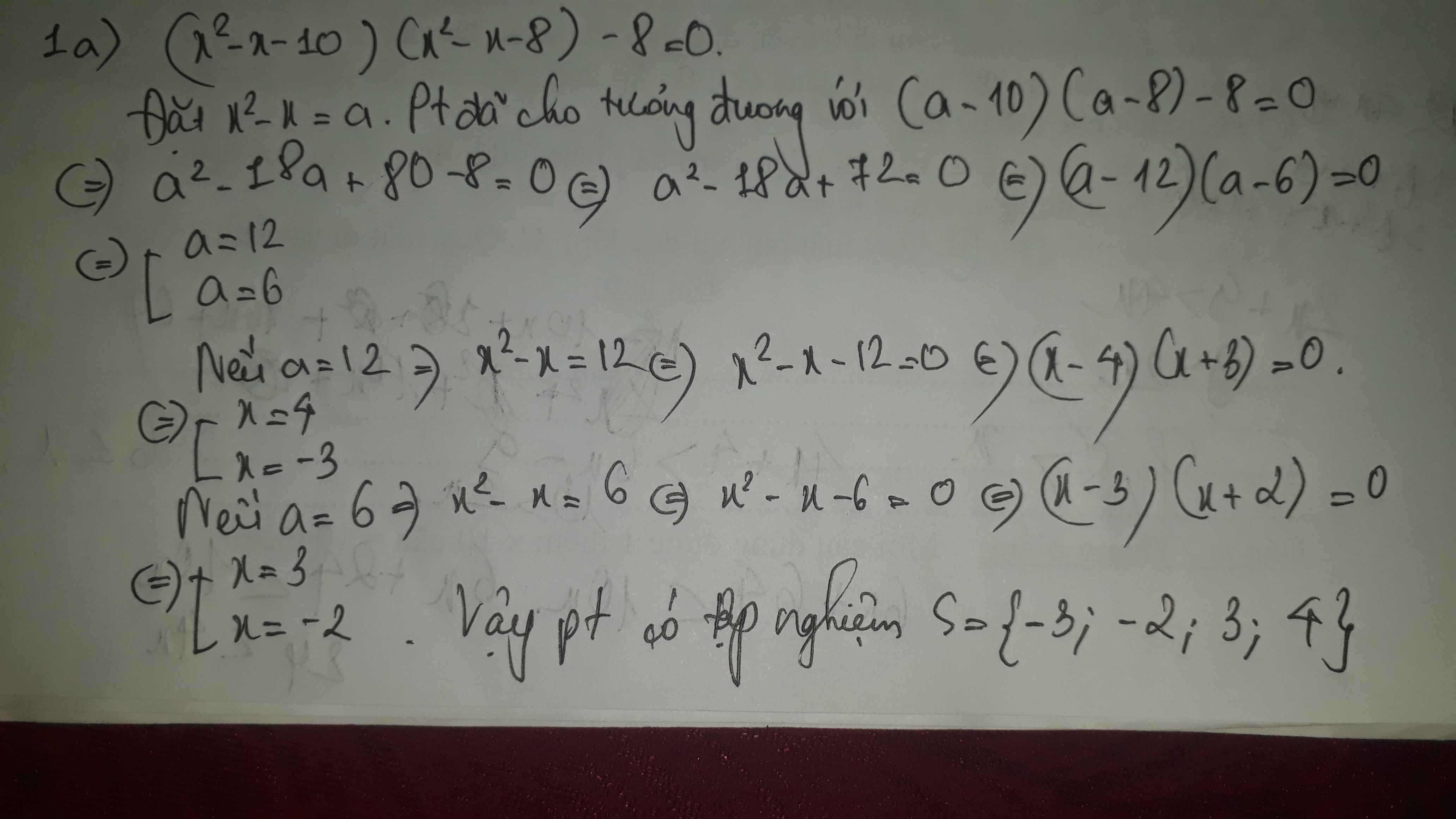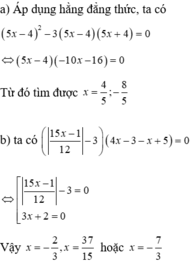a,

<=>(x+3)(x⁴-3x³-6x²+18x-9)=0

sau đó vô (Trích: Dự án phần mềm giải phương trình bậc 4 của Bùi Thế Việt ...

b,GPT: $x^5+10x^3+20x-18=0 - Diễn đàn Toán học

a/ \(\Leftrightarrow\left(x+3\right)\left(x^4-3x^3-6x^2+18x-9\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x^4-3x^3-6x^2+18x-9=0\left(1\right)\end{matrix}\right.\)

Xét (1):

\(\Leftrightarrow x^4-3x^3+3x^2-9x^2+18x-9=0\)

\(\Leftrightarrow x^4-3x^2\left(x-1\right)-9\left(x-1\right)^2=0\)

Nhận thấy \(x=1\) không phải nghiệm, chia 2 vế cho \(\left(x-1\right)^2\)

\(\left(\frac{x^2}{x-1}\right)^2-\frac{3x^2}{x-1}-9=0\)

Đặt \(\frac{x^2}{x-1}=a\Rightarrow a^2-3a-9=0\Rightarrow...\)

b/ ĐKXĐ: ...

\(\Leftrightarrow11-\frac{25x^2}{\left(x+5\right)^2}=x^2\)

\(\Leftrightarrow x^2+\frac{25x^2}{\left(x+5\right)^2}-2.x.\frac{5x}{x+5}+\frac{10x^2}{x+5}-11=0\)

\(\Leftrightarrow\left(x-\frac{5x}{x+5}\right)^2+\frac{10x^2}{x+5}-11=0\)

\(\Leftrightarrow\left(\frac{x^2}{x+5}\right)^2+\frac{10x^2}{x+5}-11=0\)

Đặt \(\frac{x^2}{x+5}=a\Rightarrow a^2+10a-11=0\)

c/ Nhận thấy \(x=y=0\) là nghiệm

Với \(x;y\ne0\), đặt \(y=kx\) ta được:

\(\left\{{}\begin{matrix}x^3-k^2x^3+2000kx=0\\k^3x^3-kx^3-500x=0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x^2-k^2x^2+2000k=0\\k^3x^2-kx^2-500=0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x^2\left(k^2-1\right)=2000k\\x^2\left(k^3-k\right)=500\end{matrix}\right.\)

Nhận thấy \(k=\left\{-1;0;1\right\}\) không thỏa mãn

\(\Rightarrow\left\{{}\begin{matrix}x^2=\frac{2000k}{k^2-1}\\x^2=\frac{500}{k^3-k}\end{matrix}\right.\) \(\Rightarrow\frac{2000k}{k^2-1}=\frac{500}{k\left(k^2-1\right)}\)

\(\Rightarrow4k^2=1\Rightarrow\left[{}\begin{matrix}k=\frac{1}{2}\\k=-\frac{1}{2}\end{matrix}\right.\)

- Với \(k=\frac{1}{2}\Rightarrow x=2y\) thay vào pt dưới: \(y^3-4y^3-1000y=0\)

- Với \(k=-\frac{1}{2}\Rightarrow x=-2y\Rightarrow y^3-4y^3+1000y=0\)

\(x^6-6x^5+15x^4-20x^3+15x^2-6x+1=0\)

\(\Leftrightarrow x^6-x^5-5x^5+5x^4+10x^4-10x^3-10x^3+10x^2+5x^2-5x-x+1=0\)

\(\Leftrightarrow x^5\left(x-1\right)-5x^4\left(x-1\right)+10x^3\left(x-1\right)-10x^2\left(x-1\right)+5x\left(x-1\right)-\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x^5-5x^4+10x^3-10x^2+5x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left[x^5-x^4-4x^4+4x^3+6x^3-6x^2-4x^2+4x+x-1\right]=0\)

\(\Leftrightarrow\left(x-1\right)\left[x^4\left(x-1\right)-4x^3\left(x-1\right)+6x^2\left(x-1\right)-4x\left(x-1\right)+x-1\right]=0\)

\(\Leftrightarrow\left(x-1\right)^2\left[x^4-4x^3+6x^2-4x+1\right]=0\)

\(\Leftrightarrow\left(x-1\right)^2\left[x^4-x^3-3x^3+3x^2+3x^2-3x-x+1\right]=0\)

\(\Leftrightarrow\left(x-1\right)^3\left[x^3-3x^2+3x-1\right]=0\)

\(\Leftrightarrow\left(x-1\right)^3\left[x^3-x^2-2x^2+2x+x-1\right]=0\)

\(\Leftrightarrow\left(x-1\right)^4\left[x^2-2x+1\right]=0\Leftrightarrow\left(x-1\right)^6=0\Leftrightarrow x=1\)

a, \(\left(x+2\right)^3-x\left(x^2+6x-3\right)=0\Leftrightarrow x^3+4x^2+4x+2x^2+8x+8-x^3-6x^2+3x=0\)

\(\Leftrightarrow15x+8=0\Leftrightarrow x=-\frac{8}{15}\)

b, \(\left(x+4\right)^3-x\left(x+6\right)^2=7\Leftrightarrow12x+64=0\Leftrightarrow x=-\frac{19}{4}\)làm tắt:P 

Tự làm nốt nhé 

\(x^3-7x^2+15x-25=0\)

\(\Leftrightarrow\left(x^3-5x^2\right)-\left(2x^2-10x\right)+\left(5x-25\right)=0\)

\(\Leftrightarrow x^2\left(x-5\right)-2x\left(x-5\right)+5\left(x-5\right)=0\)

\(\Leftrightarrow\left(x-5\right)\left(x^2-2x+5\right)=0\)(*)

Ta thấy \(x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4>0\forall x\)

Dể pt (*) xảy ra \(\Leftrightarrow x-5=0\Leftrightarrow x=5\)

Vậy \(x=5\)

x^3 - 7x^2 + 15x - 25 = 0 

x3 + -7x2 + 15x + -25 = 0

Sắp xếp lại các điều khoản: -25 + 15x + -7x2 + x3 = 0

Giải quyết -25 + 15x + -7x2 + x3 = 0

Giải quyết cho biến 'x'.

P/s : Giải pháp cho phương trình này không thể xác định.

Mk nghĩ là vậy , chúc bạn thành công trong cuộc sống !

CM: 5x^2 +15x+20>0

Ta có: 5x^2 +15x +20

= 5( x^2 + 3x +4) 

=5[(x^2 + 2.x.3/2 +9/4) -9/4 +4 ]

=5(x+3/2)^2 -7/4

Vì (x+3/2)^2 >0 với mọi x

=>5(x+3/2)^2 >0 với mọi x

=> 5(x+3/2)^2 - 7/4 >0 với mọi x

2x³ - 15x² + 26x - 5 = 0

<=> 2x³ - 10x² - 5x² + 25x + x - 5 = 0

<=> 2x²( x - 5 ) - 5x( x - 5 ) + ( x - 5 ) = 0

<=> ( x - 5 )( 2x² - 5x + 1 ) = 0

<=> \(\orbr{\begin{cases}x-5=0\\2x^2-5x+1=0\end{cases}}\)

+) x - 5 = 0 <=> x = 5

+) 2x² - 5x + 1 = 0

Δ = b² - 4ac = (-5)² - 4.2.1 = 25 - 8 = 17

Δ > 0, áp dụng công thức nghiệm thu được \(x_1=\frac{5+\sqrt{17}}{4};x_2=\frac{5-\sqrt{17}}{4}\)

Vậy phương trình đã cho có ba nghiệm \(x_1=\frac{5+\sqrt{17}}{4};x_2=\frac{5-\sqrt{17}}{4};x_3=5\)