tìm m để pt: x2 - 2x - (m - 1)(m - 3) = 0 có 2 nghiệm x1, x2 sao cho A = (x1 + 1).x2 đạt GTLN
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




\(\Delta'=\left(m+1\right)^2-\left(5m+1\right)=m^2-3m\ge0\Rightarrow\left[{}\begin{matrix}m\ge3\\m\le0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=5m+1\end{matrix}\right.\)
a.
\(S=\left(x_1+x_2\right)^2-3x_1x_2=4\left(m+1\right)^2-3\left(5m+1\right)\)
\(=4m^2-7m+1=\dfrac{7}{3}\left(m^2-3m\right)+\dfrac{5}{3}m^2+1\ge1\)
\(S_{min}=1\) khi \(\dfrac{7}{3}\left(m^2-3m\right)+\dfrac{5}{3}m^2=0\Rightarrow m=0\)
b.
\(1< x_1< x_2\Rightarrow\left\{{}\begin{matrix}\left(x_1-1\right)\left(x_2-1\right)>0\\\dfrac{x_1+x_2}{2}>1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_1x_2-\left(x_1+x_2\right)+1>0\\x_1+x_2>2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}5m+1-2\left(m+1\right)+1>0\\2\left(m+1\right)>0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}m>0\\m>-1\end{matrix}\right.\) \(\Rightarrow m>0\)
Kết hợp điều kiện delta \(\Rightarrow m\ge3\)
\(a,\Leftrightarrow\Delta\ge0\Leftrightarrow\left(2m+2\right)^2-4\left(5m+1\right)\ge0\Leftrightarrow4m^2-12m\ge0\)
\(\Leftrightarrow\left[{}\begin{matrix}m\le0\\m\ge3\end{matrix}\right.\)
\(vi-ét\Rightarrow\left\{{}\begin{matrix}x1+x2=2m+2\\x1x2=5m+1\end{matrix}\right.\)
\(\Rightarrow S=x1^2+x2^2-x1x2=\left(x1+x2\right)^2-3x1x2\)
\(=\left(2m+2\right)^2-3\left(5m+1\right)=4m^2-7m+1\)
\(=\left(2m\right)^2-2.2.\dfrac{7}{4}.m+\left(\dfrac{7}{4}\right)^2-\dfrac{33}{16}=\left(2m-\dfrac{7}{4}\right)^2-\dfrac{33}{16}\left(1\right)\)
\(TH1:m\ge3\Rightarrow\left(1\right)\ge\left(2.3-\dfrac{7}{4}\right)^2-\dfrac{33}{16}=16\)
\(TH2:m\le0\Rightarrow\left(1\right)\ge\left(0-\dfrac{7}{4}\right)^2-\dfrac{33}{16}=1\)
\(\Rightarrow MinS=1\Leftrightarrow m=0\left(tm\right)\)
\(b,1< x1< x2\Leftrightarrow0< x1-1< x2-1\)
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta>0\\\left(x1-1\right)\left(x2-1\right)>0\\x1+x2-2>0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>3\\m< 0\end{matrix}\right.\\\left[{}\begin{matrix}\left\{{}\begin{matrix}x1>1\\x2>1\end{matrix}\right.\\\left\{{}\begin{matrix}x1 < 1\\x2< 1\end{matrix}\right.\end{matrix}\right.\\2m+2-2>0\\\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>3\\m< 0\end{matrix}\right.\\\left[{}\begin{matrix}x1x2>1\\x1x2< 1\end{matrix}\right.\\m>0\\\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>3\\m< 0\end{matrix}\right.\\\left[{}\begin{matrix}m>0\\m< 0\end{matrix}\right.\\m>0\\\end{matrix}\right.\Rightarrow m>3\)

Bài 1:
a, Thay m=-1 vào (1) ta có:
\(x^2-2\left(-1+1\right)x+\left(-1\right)^2+7=0\\
\Leftrightarrow x^2+1+7=0\\
\Leftrightarrow x^2+8=0\left(vô.lí\right)\)
Thay m=3 vào (1) ta có:
\(x^2-2\left(3+1\right)x+3^2+7=0\\ \Leftrightarrow x^2-2.4x+9+7=0\\ \Leftrightarrow x^2-8x+16=0\\ \Leftrightarrow\left(x-4\right)^2=0\\ \Leftrightarrow x-4=0\\ \Leftrightarrow x=4\)
b, Thay x=4 vào (1) ta có:
\(4^2-2\left(m+1\right).4+m^2+7=0\\ \Leftrightarrow16-8\left(m+1\right)+m^2+7=0\\ \Leftrightarrow m^2+23-8m-8=0\\ \Leftrightarrow m^2-8m+15=0\\ \Leftrightarrow\left(m^2-3m\right)-\left(5m-15\right)=0\\ \Leftrightarrow m\left(m-3\right)-5\left(m-3\right)=0\\ \Leftrightarrow\left(m-3\right)\left(m-5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}m=3\\m=5\end{matrix}\right.\)
c, \(\Delta'=\left[-\left(m+1\right)\right]^2-\left(m^2+7\right)=m^2+2m+1-m^2-7=2m-6\)
Để pt có 2 nghiệm thì \(\Delta'\ge0\Leftrightarrow2m-6\ge0\Leftrightarrow m\ge3\)
Theo Vi-ét:\(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=m^2+7\end{matrix}\right.\)
\(x_1^2+x_2^2=0\\ \Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=0\\ \Leftrightarrow\left(2m+2\right)^2-2\left(m^2+7\right)=0\\ \Leftrightarrow4m^2+8m+4-2m^2-14=0\\ \Leftrightarrow2m^2+8m-10=0\\ \Leftrightarrow\left[{}\begin{matrix}m=1\left(ktm\right)\\m=-5\left(ktm\right)\end{matrix}\right.\)
\(x_1-x_2=0\\ \Leftrightarrow\left(x_1-x_2\right)^2=0\\ \Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=0\\ \Leftrightarrow\left(2m+2\right)^2-4\left(m^2+7\right)=0\\ \Leftrightarrow4m^2+8m+4-4m^2-28=0\\ \Leftrightarrow8m=28=0\\ \Leftrightarrow m=\dfrac{7}{2}\left(tm\right)\)
Bài 2:
a,Thay m=-2 vào (1) ta có:
\(x^2-2x-\left(-2\right)^2-4=0\\ \Leftrightarrow x^2-2x-4-4=0\\ \Leftrightarrow x^2-2x-8=0\\ \Leftrightarrow\left[{}\begin{matrix}x=4\\x=-2\end{matrix}\right.\)
b, \(\Delta'=\left(-m\right)^2-\left(-m^2-4\right)\ge0=m^2+m^2+4=2m^2+4>0\)
Suy ra pt luôn có 2 nghiệm phân biệt
Theo Vi-ét:\(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=-m^2-4\end{matrix}\right.\)
\(x_1^2+x_2^2=20\\ \Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=20\\ \Leftrightarrow2^2-2\left(-m^2-4\right)=20\\ \Leftrightarrow4+2m^2+8-20=0\\ \Leftrightarrow2m^2-8=0\\ \Leftrightarrow m=\pm2\)
\(x_1^3+x_2^3=56\\ \Leftrightarrow\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)=56\\ \Leftrightarrow2^3-3\left(-m^2-4\right).2=56\\ \Leftrightarrow8-6\left(-m^2-4\right)-56\\ =0\\ \Leftrightarrow8+6m^2+24-56=0\\ \Leftrightarrow6m^2-24=0\\ \Leftrightarrow m=\pm2\)
\(x_1-x_2=10\\ \Leftrightarrow\left(x_1-x_2\right)^2=100\\ \Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2-100=0\\ \Leftrightarrow2^2-4\left(-m^2-4\right)-100=0\\ \Leftrightarrow4+4m^2+16-100=0\\ \Leftrightarrow4m^2-80=0\\ \Leftrightarrow m=\pm2\sqrt{5}\)

a, \(\Delta'=m^2-\left(m^2-4\right)=4>0\)
Vậy pt luôn có 2 nghiệm pb x1;x2
Theo Vi et \(\hept{\begin{cases}x_1+x_2=2m\\x_1x_2=m^2-4\end{cases}}\)
Ta có : \(2x_1-3x_2=-1\left(3\right)\)Từ (1) ;(3) ta có hệ
\(\hept{\begin{cases}2x_1+2x_2=4m\\2x_1-3x_2=-1\end{cases}}\Leftrightarrow\hept{\begin{cases}5x_2=4m+1\\x_1=2m-x_2\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x_2=\frac{4m+1}{5}\\x_1=\frac{10-4m-1}{5}=\frac{-4m+9}{5}\end{cases}}\)
Thay vào (2) ta được \(\frac{\left(4m+1\right)\left(-4m+9\right)}{25}=m^2-4\)
\(\Rightarrow-16m^2+36m-4m+9=25\left(m^2-4\right)\)
\(\Leftrightarrow41m^2-32m-109=0\)
bạn tự tính = delta' nhé, có gì sai bảo mình do số khá to và phức tạp á
b, Ta có \(\left|x_1\right|=\left|x_2\right|\)suy ra
\(\left|\frac{4m+1}{5}\right|=\left|\frac{9-4m}{5}\right|\Rightarrow\left|4m+1\right|=\left|9-4m\right|\)
TH1 : \(4m+1=9-4m\Leftrightarrow8m=8\Leftrightarrow m=1\)
TH2 : \(4m+1=4m-9\left(voli\right)\)
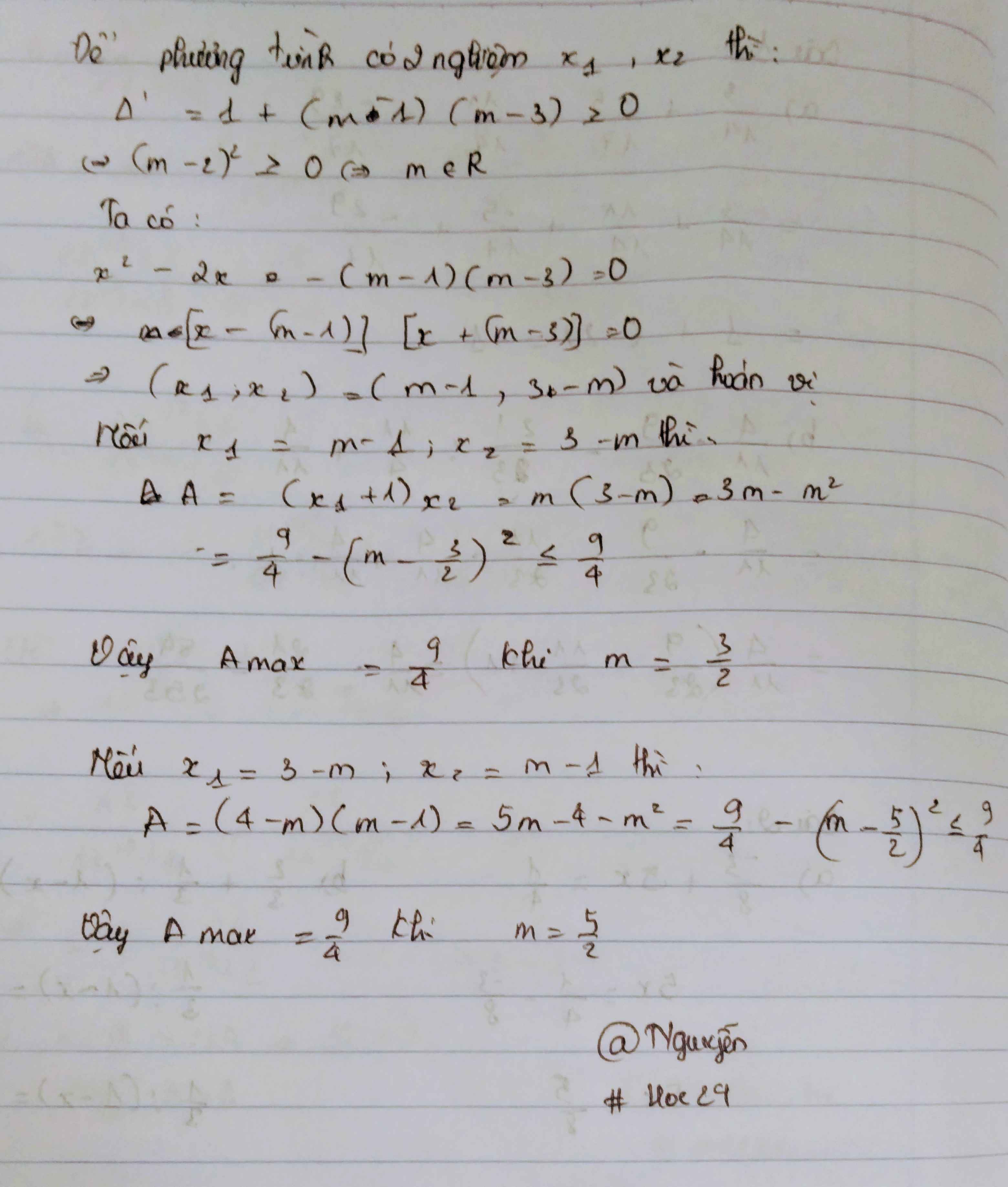
Lời giải:
Để pt có 2 nghiệm $x_1,x_2$ thì:
$\Delta'=1+(m-1)(m-3)\geq 0\Leftrightarrow (m-2)^2\geq 0\Leftrightarrow m\in\mathbb{R}$
Ta có:
$x^2-2x-(m-1)(m-3)=0$
$\Leftrightarrow [x-(m-1)][x+(m-3)]=0$
$\Rightarrow (x_1,x_2)=(m-1,3-m)$ và hoán vị
Nếu $x_1=m-1; x_2=3-m$ thì: $A=(x_1+1)x_2=m(3-m)=3m-m^2=\frac{9}{4}-(m-\frac{3}{2})^2\leq \frac{9}{4}$
Vậy $A_{\max}=\frac{9}{4}$ khi $m=\frac{3}{2}$
Nếu $x_1=3-m; x_2=m-1$ thì:
$A=(4-m)(m-1)=5m-4-m^2=\frac{9}{4}-(m-\frac{5}{2})^2\leq \frac{9}{4}$
Vậy $A_{\max}=\frac{9}{4}$ khi $m=\frac{5}{2}$
Vậy tóm lại $m=\frac{3}{2}$ hoặc $m=\frac{5}{2}$ thì $A_{\max}$