Tính giá trị của biểu thức tan 41° - cot 49°
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(tana-cota=2\sqrt{3}\Rightarrow\left(tana-cota\right)^2=12\)
\(\Rightarrow\left(tana+cota\right)^2-4=12\Rightarrow\left(tana+cota\right)^2=16\)
\(\Rightarrow P=4\)
\(sinx+cosx=\dfrac{1}{5}\Rightarrow\left(sinx+cosx\right)^2=\dfrac{1}{25}\)
\(\Rightarrow1+2sinx.cosx=\dfrac{1}{25}\Rightarrow sinx.cosx=-\dfrac{12}{25}\)
\(P=\dfrac{sinx}{cosx}+\dfrac{cosx}{sinx}=\dfrac{sin^2x+cos^2x}{sinx.cosx}=\dfrac{1}{sinx.cosx}=\dfrac{1}{-\dfrac{12}{25}}=-\dfrac{25}{12}\)

\(tan^2x+cot^2x=2=2.tanx.cotx\)
\(\Leftrightarrow tan^2x+cot^2x-2tanx.cotx=0\)
\(\Leftrightarrow\left(tanx-cotx\right)^2=0\Leftrightarrow tanx=cotx=\dfrac{1}{tanx}\)
\(\Leftrightarrow tanx=\pm1\)
\(P=\dfrac{1}{cosx}-\dfrac{cosx}{1+sinx}=\dfrac{1+sinx-cos^2x}{cosx\left(1+sinx\right)}=\dfrac{sin^2x+sinx}{cosx\left(1+sinx\right)}\)
\(=\dfrac{sinx\left(1+sinx\right)}{cosx\left(1+sinx\right)}=tanx=\pm1\)

\(a,\tan10.\tan11......\)
\(=\left(\tan10.tan80\right)\left(tan11.tan79\right)....\left(tan44.tan46\right).tan45\)
Mà 10 và 80, 11 và 79, ... là các góc phụ nhau .
\(=tan10.cot10....tan45=1\)
b, Ta có : \(\tan x+\cot x=2\)
\(\Rightarrow\tan^2x+2\tan x\cot x+\cot^2x=4\)
\(\Rightarrow\tan^2x+\cot^2x=4-2=2\)
Ta có : \(\tan^3x+\cot^3x=\left(\tan x+\cot x\right)\left(\tan^2x-\tan x\cot x+\cot^2x\right)=2\)

Ta có:
\(\dfrac{cot\alpha-tan\alpha}{cot\alpha+tan\alpha}=\dfrac{cot\alpha.cot\alpha-cot\alpha tan\alpha}{cot\alpha.cot\alpha+cot\alpha tan\alpha}=\dfrac{cot^2\alpha-1}{cot^2\alpha+1}\)
\(=\dfrac{\dfrac{1}{sin^2\alpha}-2}{\dfrac{1}{sin^2\alpha}}=1-2sin^2\alpha=1-2\left(\dfrac{2}{3}\right)^2=\dfrac{1}{9}\).

Ta có: \(\sin {70^o} = \cos {20^o};\;\cos {110^o} = - \cos {70^o} = - \sin {20^o}\)
\(\begin{array}{l} \Rightarrow A = {(\sin {20^o} + \cos {20^o})^2} + {(\cos {20^o} - \sin {20^o})^2}\\ = ({\sin ^2}{20^o} + {\cos ^2}{20^o} + 2\sin {20^o}\cos {20^o}) + ({\cos ^2}{20^o} + {\sin ^2}{20^o} - 2\sin {20^o}\cos {20^o})\\ = 2({\sin ^2}{20^o} + {\cos ^2}{20^o})\\ = 2\end{array}\)
Ta có: \(\tan {110^o} = - \tan {70^o} = - \cot {20^o};\;\cot {110^o} = - \cot {70^o} = - \tan {20^o}.\)
\( \Rightarrow B = \tan {20^o} + \cot {20^o} + ( - \cot {20^o}) + ( - \tan {20^o}) = 0\)

\(\left(\tan\alpha;\cot\alpha\right)=\left(a;b\right)\) cho gọn, trong đó \(b=\frac{1}{a}\)
\(B=a+b+\frac{4}{a+b}-\frac{3}{a+b}\ge2\sqrt{\frac{4\left(a+b\right)}{a+b}}-\frac{3}{a+\frac{1}{a}}\ge4-\frac{3}{2}=\frac{5}{2}\)
Dấu "=" xảy ra khi \(\tan\alpha=\cot\alpha=1\)

Ta có
\(\begin{array}{l}Q = {\tan ^2}\frac{\pi }{3} + {\sin ^2}\frac{\pi }{4} + \cot \frac{\pi }{4} + \cos \frac{\pi }{2}\\\,\,\,\,\, = \,{\left( {\sqrt 3 } \right)^2} + {\left( {\frac{{\sqrt 2 }}{2}} \right)^2} + 1 + 0 = \frac{7}{2}\end{array}\)
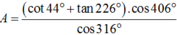
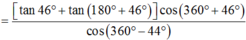
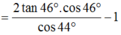

\(=\cot49^0-\cot49^0=0\)