cho biểu thức:B=x+12/căn x +2.Chứng minh B lớn hơn bằng 4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
30 tháng 4 2017
\(\sqrt{3x^2+6x+12}+\sqrt{5x^4-10x^2+9}\\ =\sqrt{3\left(x^2+2x+1\right)+9}+\sqrt{5\left(\left(x^2\right)^2-2x^2+1\right)+4}\\ =\sqrt{3\left(x+1\right)^2+9}+\sqrt{5\left(x^2-1\right)^2+4}\)
do: \(+\left(x+1\right)^2\ge0\Rightarrow3.\left(x+1\right)^2+9\ge9\Rightarrow\sqrt{3\left(x+1\right)^2+9}\ge\sqrt{9}=3\)(1)\(+\left(x^2-1\right)^2\ge0\Rightarrow5\left(x^2-1\right)^2+4\ge4\Rightarrow\sqrt{5\left(x^2-1\right)^2+4}\ge\sqrt{4}=2\)(2)
từ (1) và(2)\(\Rightarrow\sqrt{3\left(x+1\right)^2+9}+\sqrt{5\left(x^2-1\right)^2+4}\ge3+2=5\)
câu b bạn làm tương tự
21 tháng 9 2021
\(A=\dfrac{2\sqrt{x}}{\sqrt{x}-4}-\dfrac{x+12\sqrt{x}}{x-16}\left(x\ge0;x\ne16\right)\\ A=\dfrac{2\sqrt{x}\left(\sqrt{x}+4\right)-x-12\sqrt{x}}{\left(\sqrt{x}-4\right)\left(\sqrt{x}+4\right)}\\ A=\dfrac{2x+8\sqrt{x}-x-12\sqrt{x}}{\left(\sqrt{x}-4\right)\left(\sqrt{x}+4\right)}=\dfrac{\sqrt{x}\left(\sqrt{x}-4\right)}{\left(\sqrt{x}-4\right)\left(\sqrt{x}+4\right)}=\dfrac{\sqrt{x}}{\sqrt{x}+4}\)
AH
Akai Haruma
Giáo viên
4 tháng 5 2023
Bạn nên viết đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo) để được hỗ trợ tốt hơn.
HQ
Hà Quang Minh
Giáo viên
12 tháng 8 2023
\(a_1,\sqrt{x}< 7\\ \Rightarrow x< 49\\ a_2,\sqrt{2x}< 6\\ \Rightarrow x< 18\\ a_3,\sqrt{4x}\ge4\\ \Rightarrow4x\ge16\\ \Rightarrow x\ge4\\ a_4,\sqrt{x}< \sqrt{6}\\ \Rightarrow x< 6\)
HQ
Hà Quang Minh
Giáo viên
12 tháng 8 2023
\(b_1,\sqrt{x}>4\\ \Rightarrow x>16\\ b_2,\sqrt{2x}\le2\\ \Rightarrow2x\le4\\ \Rightarrow x\le2\\ b_3,\sqrt{3x}\le\sqrt{9}\\ \Rightarrow3x\le9\\ \Rightarrow x\le3\\ b_4,\sqrt{7x}\le\sqrt{35}\\ \Rightarrow7x\le35\\ \Rightarrow x\le5\)
TN
0
4 tháng 4 2015
Câu a)
Ta có a + b \(\ge\)1 => a \(\ge\) 1 - b
Nên a2 + b2 \(\ge\) (1 - b)2 + b2 = 2b2 - 2b + 1 = 2(b2 - 2b.1/2 + 1/4 + 1/2) = 2(b - 1/2)2 + 1 \(\ge\) 1
Câu b) Áp dụng BĐT Bunhiacopxki ta có
(x + y)2 = (1.x + 1.y)2 \(\le\) (12 + 12)(x2 + y2) = 2.1 = 2
Dấu "=" xảy ra <=> x = y
4 tháng 4 2015
câu1 : cần sửa lại là A2 + B2 \(\ge\frac{1}{2}\)
Ta chứng minh được : (A+B)2 \(\le2.\left(A^2+B^2\right)\) (*)
<=> A2 + B2 + 2A.B \(\le\) 2. (A2 + B2)
<=> 0 \(\le\) A2 + B2 - 2.A.B <=> 0 \(\le\) (A-B)2 luôn đúng => (*) đúng
b) Áp sung câu a => (x+y)2 \(\le\)2.(x2 + y2) = 2 => đpcm
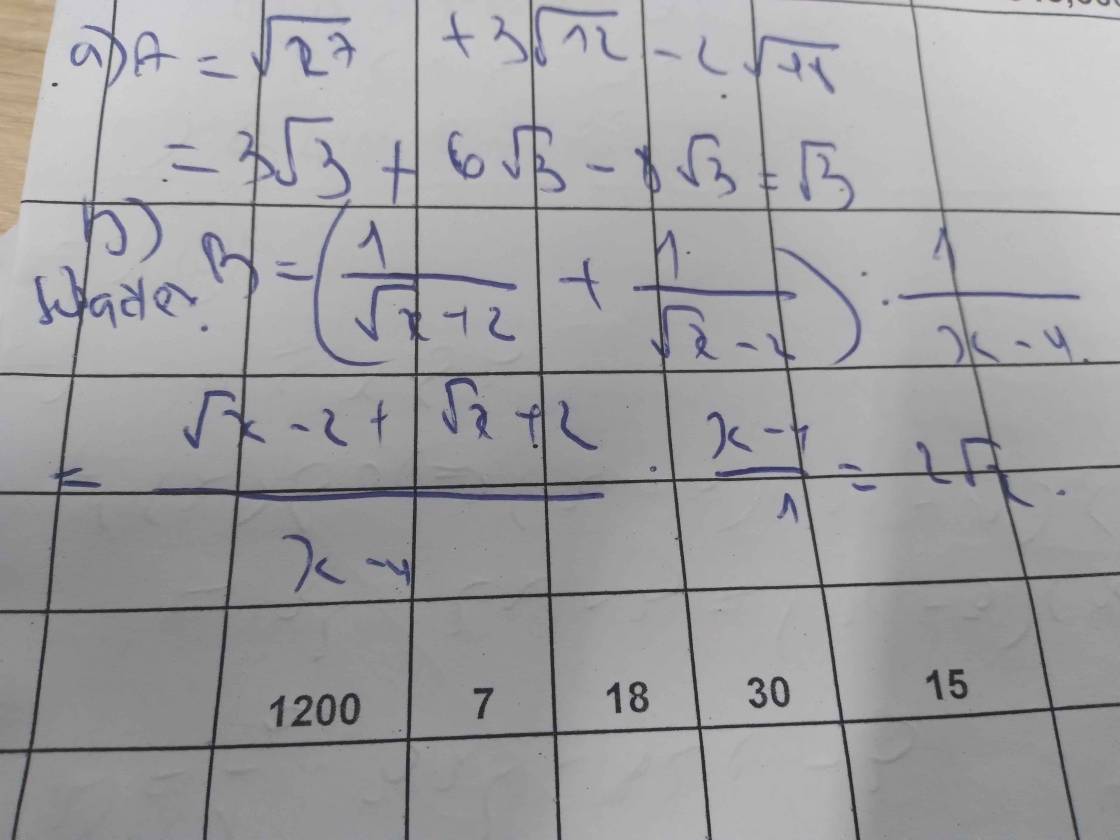
\(B=\dfrac{x+12}{\sqrt{x}+2}\) (điều kiện \(x\ge0\))
Đặt \(\sqrt{x}=t\left(t\ge0\right)\), khi đó \(B=\dfrac{t^2+12}{t+2}\) \(\Rightarrow\left(t+2\right)B=t^2+12\) \(\Leftrightarrow t^2-B.t+12-2B=0\) (*)
Phương trình (*) có \(\Delta=\left(-B\right)^2-4.1.\left(12-2B\right)\) \(=B^2+8B-48\)
Để pt (*) có nghiệm thì \(\Delta\ge0\) hay \(B^2+8B-48\ge0\) \(\Leftrightarrow\left(B+12\right)\left(B-4\right)\ge0\)
Ta xét 2 trường hợp:
TH1: \(\left\{{}\begin{matrix}B+12\ge0\\B-4\ge0\end{matrix}\right.\Leftrightarrow B\ge4\)
TH2: \(\left\{{}\begin{matrix}B+12\le0\\B-4\le0\end{matrix}\right.\Leftrightarrow B\le-12\)
Ta thấy do \(x\ge0\) nên \(x+12>0\) và \(\sqrt{x}+2>0\). Do đó \(B>0\). Vậy trường hợp 2 \(\left(B\le-12\right)\) là không thể xảy ra. Vậy ta chỉ nhận trường hợp \(B\ge4\) hay \(min_B=4\)
Khi \(B=4\) thì thay vào (*), ta có \(t^2-4t+12-2.4=0\) \(\Leftrightarrow t^2-4t+4=0\) \(\Leftrightarrow\left(t-2\right)^2=0\) \(\Leftrightarrow t=2\) (nhận)
\(\Leftrightarrow\sqrt{x}=2\) \(\Leftrightarrow x=4\) (nhận)
Vậy \(B\ge4\). Dấu "=" xảy ra khi \(x=4\)