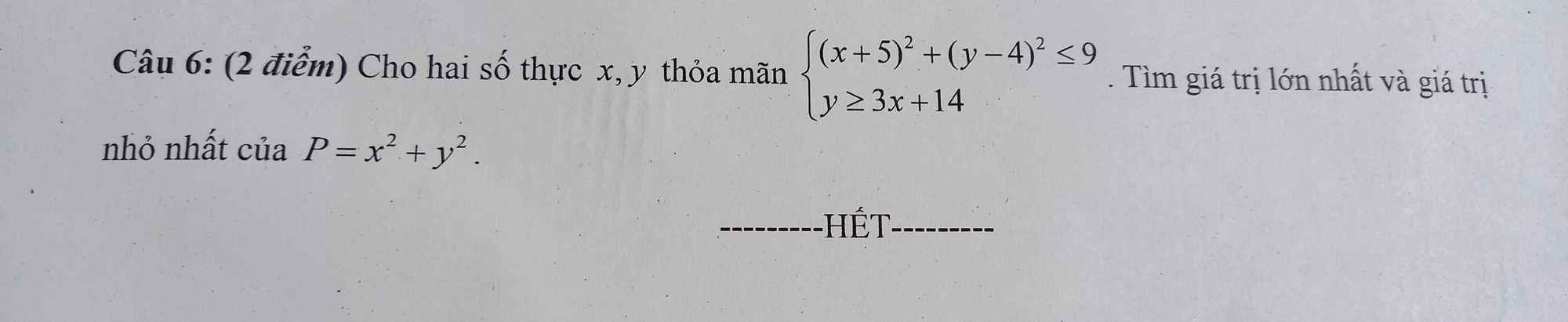Đk:\(y^2-2x-5y+6\ge0\)

Pt (1)\(\Leftrightarrow\left(x^2-1\right)-\left(xy-y\right)+\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+1\right)-y\left(x-1\right)+\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2-y\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\y=x+2\end{matrix}\right.\)

TH1: Thay x=1 vào pt (2) ta đc: \(3\sqrt{y^2-5y+4}=y+9\)

\(\Leftrightarrow\left\{{}\begin{matrix}y+9\ge0\\9\left(x^2-5y+4\right)=y^2+18y+81\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}y\ge-9\\8y^2-63y-45=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}y=\dfrac{63+3\sqrt{601}}{16}\\y=\dfrac{63-3\sqrt{601}}{16}\end{matrix}\right.\) (tm)

TH2: Thay y=x+2 vào pt (2) ta đc:

\(\left(x-1\right)^2+3\sqrt{\left(x+2\right)^2-2x-5\left(x+2\right)+6}=x+2+9\)

\(\Leftrightarrow x^2-3x-10+3\sqrt{x^2-3x}=0\)

Đặt \(t=\sqrt{x^2-3x}\left(t\ge0\right)\)

Pttt: \(t^2-10+3t=0\)\(\Leftrightarrow\left[{}\begin{matrix}t=2\left(tm\right)\\t=-5\left(ktm\right)\end{matrix}\right.\)

\(\Rightarrow2=\sqrt{x^2-3x}\)\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-1\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}y=6\\y=1\end{matrix}\right.\) (tm)

Vậy \(\left(x;y\right)=\text{​​}\left\{\left(1;\dfrac{63+3\sqrt{601}}{16}\right);\left(1;\dfrac{63-3\sqrt{601}}{16}\right),\left(4;6\right),\left(-1;1\right)\right\}\)

Xét pt đầu:

\(\left(x^2+x-2\right)-y\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2\right)-y\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2-y\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\y=x+2\end{matrix}\right.\)

- Với \(x=1\) thay xuống pt dưới:

\(3\sqrt{y^2-5y+4}=y+9\) \(\left(y\ge-9\right)\)

\(\Leftrightarrow9\left(y^2-5y+4\right)=y^2+18y+81\)

\(\Leftrightarrow8y^2-63y-45=0\)

\(\Rightarrow y=\dfrac{63\pm3\sqrt{601}}{16}\) (thỏa mãn)

- Với \(y=x+2\) thay xuống pt dưới:

\(\left(x-1\right)^2+3\sqrt{x^2-3x}=x+11\) (ĐKXĐ: ....)

\(\Leftrightarrow x^2-3x+3\sqrt{x^2-3x}-10=0\)

Đặt \(\sqrt{x^2-3x}=t\ge0\)

\(\Rightarrow t^2+3t-10=0\Rightarrow\left[{}\begin{matrix}t=2\\t=-5\left(loại\right)\end{matrix}\right.\)

\(\Rightarrow\sqrt{x^2-3x}=2\Leftrightarrow x^2-3x-4=0\)

\(\Leftrightarrow...\)

- Xét : \(x^2+8x-20\le0\)

\(\Rightarrow-10\le x\le2\)

Mà \(x>0\)

\(\Rightarrow0< x\le2\)

- Xét  \(x^2-2\left(m+3\right)x+m^2-2m< 0\)

Có : \(\Delta^,=b^{,2}-ac=\left(m+3\right)^2-\left(m^2-2m\right)\)

\(=m^2+6m+9-m^2+2m=8m+9\)

- Để bất phương trình có nghiệm

\(\Leftrightarrow\Delta>0\)

\(\Leftrightarrow m>-\dfrac{9}{8}\)

=> Bất phương trình có nghiệm \(S=\left(x_1;x_2\right)\)

Mà \(0< x\le2\)

\(\Rightarrow0< x_1< x_2\le2\)

\(TH1:x=2\)

\(\Rightarrow4-4\left(m+3\right)+m^2-2m< 0\)

\(\Rightarrow3-\sqrt{17}< m< 3+\sqrt{17}\)

\(TH2:0< x_1< x_2< 2\)

\(\Rightarrow\left\{{}\begin{matrix}m^2-2m>0\\m^2-6m-8>0\\0< 2\left(m+3\right)< 2\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m< 0\\m>2\end{matrix}\right.\\\left[{}\begin{matrix}m>3+\sqrt{17}\\m< 3-\sqrt{17}\end{matrix}\right.\\-3< m< -2\end{matrix}\right.\)

Vậy \(3-\sqrt{7}< m< 3+\sqrt{7}\)

Ban ơi :(( ngay chỗ dấu ngoặc nhọn đầu tiên của TH2 có công thức j k bạn?

Nếu \(y\le0\Rightarrow\left(y-4\right)^2\ge16>9\left(ktm\right)\Rightarrow y>0\)

Nếu \(x\ge0\Rightarrow\left(x+5\right)^2\ge25>9\left(ktm\right)\Rightarrow x< 0\)

Đặt \(\left\{{}\begin{matrix}-x=a>0\\y=b>0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\left(a-5\right)^2+\left(b-4\right)^2\le9\\3a+b\ge14\end{matrix}\right.\)

Ta có:

\(14^2\le\left(3a+b\right)^2\le\left(3^2+1\right)\left(a^2+b^2\right)\Rightarrow a^2+b^2\ge\dfrac{196}{10}=\dfrac{98}{5}\)

\(P_{min}=\dfrac{98}{5}\) khi \(\left(a;b\right)=\left(\dfrac{21}{5};\dfrac{7}{5}\right)\) hay \(\left(x;y\right)=\left(-\dfrac{21}{5};\dfrac{7}{3}\right)\)

Lại có:

\(\left(a-5\right)^2+\left(b-4\right)^2\le9\Leftrightarrow a^2+b^2\le10a+8b-32\le\sqrt{\left(10^2+8^2\right)\left(a^2+b^2\right)}-32\)

\(\Rightarrow P\le2\sqrt{41}\sqrt{P}-32\Leftrightarrow P-2\sqrt{41}\sqrt{P}+32\le0\)

\(\Rightarrow\left(\sqrt{P}-3-\sqrt{41}\right)\left(\sqrt{P}-3+\sqrt{41}\right)\le0\) (1)

Do \(P\ge\dfrac{98}{5}\Rightarrow\sqrt{P}-3+\sqrt{41}>0\)

Nên (1) tương đương: \(\sqrt{P}-3-\sqrt{41}\le0\Rightarrow P\le50+6\sqrt{41}\)

\(P_{max}=50+6\sqrt{41}\) khi \(\left(a;b\right)=\left(5+\dfrac{15}{\sqrt{41}};4+\dfrac{12}{\sqrt{41}}\right)\) 

Refer

1. “Your cousin speaks English very well” Paul told me

Paul said that ___________my cousin spoke English very well____________

2. “The man broke out of prison yesterday” said the policeman

The policeman told us_that the man had broken out of prison the day beforr__

3. “I’ll lend you this book as soon as I finish it” Owen said to me

Owen said __me that he would lend me that book as soon as he finished it___

4. “I think I forgot to turn off the lights this morning” Brenda told Brian

Brenda told Brian ____that he thought he had forgotten to turn off the lights that morning.____

5. “I work eight hours a day, except when the children are on holiday” said Mrs. Wood

Mrs. Wood said me that he worked eight hours a day, excepted when the children were on holiday

6. “You’ve been making good progress this semester” Miss Lynn told me

Miss Lynn said that _____I had been making good progress that semester_________

7. “If you bought all the tickets, you would win the lottery” the man said

The man told me ______that If I had bought all the tickets, I would win the lottery______________

8. “I like swimming but I don’t go very often” Jill said to Pam

Jill said that ______he liked swimming but he didn’t go very often___________________________

9. “I want to buy it, but I haven’t brought any money” said Patrick

Patrick told me _________that he wanted to buy it, but he hadn’t brought any money_______________________

10. “I’m going to visit my aunt in Hue, but I’m not sure when” said Mai

Mai told me _________that she was going to visit her aunt in Hue, but she was not sure when__________________

3: góc AMN=góic ACM

=>AM là tiếp tuyến của đường tròn ngoại tiếp ΔECM

=>góc AMB=90 độ

=>Tâm o1 của đường tròn ngoại tiếp ΔECM nằm trên BM

NO1 min khi NO1=d(N;BM)

=>NO1 vuông góc BM

Gọi O1 là chân đường vuông góc kẻ từ N xuống BM

=>O1 là tâm đường tròn ngoại tiếp ΔECM  có bán kính là O1M
=>d(N;tâm đường tròn ngoại tiếp ΔECM) nhỏ nhất khi C là giao của (O1;O1M) với (O) với O1 ;là hình chiếu vuông góc của N trên BM