Bài 3:

3: \(6x\left(x-y\right)-9y^2+9xy\)

\(=6x\left(x-y\right)+9xy-9y^2\)

\(=6x\left(x-y\right)+9y\left(x-y\right)\)

\(=\left(x-y\right)\left(6x+9y\right)\)

\(=3\left(2x+3y\right)\left(x-y\right)\)

Bài 4:

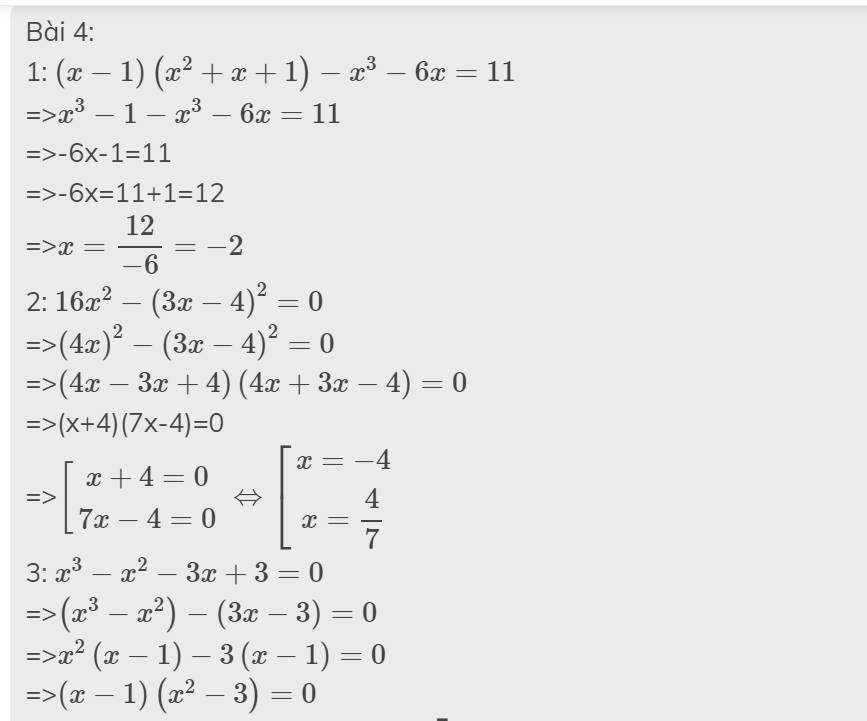
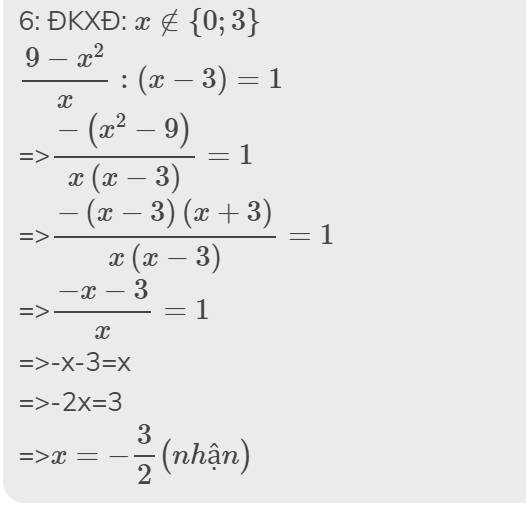
h) \(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=2\\\dfrac{3}{x}-\dfrac{4}{y}=-1\end{matrix}\right.\)\(\left(1\right)\)\(\left(đk:x,y\ne0\right)\)

Đặt \(a=\dfrac{1}{x},b=\dfrac{1}{y}\)

\(\left(1\right)\Leftrightarrow\) \(\left\{{}\begin{matrix}a+b=2\\3a-4b=-1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}3a+3b=6\\3a-4b=-1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}a+b=2\\7b=7\end{matrix}\right.\)\(\Leftrightarrow a=b=1\)

Thay a,b:

\(\Leftrightarrow\dfrac{1}{x}=\dfrac{1}{y}=1\Leftrightarrow x=y=1\left(tm\right)\)

Bài 1:

a) \(=\dfrac{8}{15}\left(\dfrac{7}{13}+\dfrac{6}{13}\right)=\dfrac{8}{15}.1=\dfrac{8}{15}\)

b) \(=\dfrac{3.3-7-2.4}{12}=-\dfrac{6}{12}=-\dfrac{1}{2}\)

Bài 2:

 \(\dfrac{x}{2,7}=-\dfrac{2}{3,6}\Rightarrow x=\dfrac{\left(-2\right).2,7}{3,6}\Rightarrow x=-\dfrac{3}{2}\)

Bài 3:

\(\dfrac{x}{2}=\dfrac{y}{5}=\dfrac{x+y}{2+5}=-\dfrac{21}{7}=-3\)

\(\Rightarrow\left\{{}\begin{matrix}x=\left(-3\right).2=-6\\y=\left(-3\right).5=-10\end{matrix}\right.\)

Tham khảo:

a)      \((4{x^2} - 5):(x - 2) = \dfrac{{4{x^2} - 5}}{{x - 2}} = 4x + 8 + \dfrac{{11}}{{x - 2}}\)

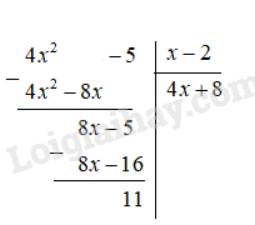
Vậy \( (4{x^2} - 5):(x - 2)= 4x + 8 + \dfrac{{11}}{{x - 2}}\)

b)      \((3{x^3} - 7x + 2):(2{x^2} - 3) = \dfrac{{3{x^3} - 7x + 2}}{{2{x^2} - 3}}\)

Vậy \( (3{x^3} - 7x + 2):(2{x^2} - 3)= \dfrac{3}{2}x + \dfrac{{\dfrac{-5}{2}x + 2}}{{2{x^2} - 3}}\)

Tham khảo:

a)      \((45{x^5} - 5{x^4} + 10{x^2}):5{x^2}\)\( = 9{x^3} - {x^2} + 2\)

b)      \((9{t^2} - 3{t^4} + 27{t^5}):3t = (27{t^5} - 3{t^4} + 9{t^2}):3t\\=(27t^5):(3t) - (3t^4):(3t)+(9t^2):(3t) = 9{t^4} - {t^3}+3t\)

Bài 3:

a) \(4x^2+4x+1=\left(2x+1\right)^2\)

b) \(9x^2-12x+4=\left(3x-2\right)^2\)

c) \(ab^2+\dfrac{1}{4}a^2b^4+1=\left(\dfrac{1}{2}ab^2+1\right)^2\)

Phần d bài 3 mk chưa lm dc

Tham khảo:

a) \((8{x^6} - 4{x^5} + 12{x^4} - 20{x^3}):4{x^3}\)

\( = (8{x^6}:4{x^3}) - (4{x^5}:4{x^3}) + (12{x^4}:4{x^3}) - (20{x^3}:4{x^3})\)

\( = 2{x^2} - {x^2} + 3x - 5\)

b) 

Vậy \((2{x^2} - 5x + 3):(2x - 3)= x - 1\)

Bài 1: 

c) \(\dfrac{1}{y}\sqrt{19y}=\sqrt{19y\cdot\dfrac{1}{y^2}}=\sqrt{\dfrac{19}{y}}\)

d) \(\dfrac{1}{3y}\cdot\sqrt{\dfrac{27}{y^2}}\cdot y=\sqrt{\dfrac{1}{9}\cdot\dfrac{27}{y^2}}=\sqrt{\dfrac{3}{y^2}}\)

Bài 3: 

a) Ta có: \(\left(\dfrac{2}{\sqrt{3}-1}+\dfrac{3}{\sqrt{3}-2}+\dfrac{15}{3-\sqrt{3}}\right)\cdot\dfrac{1}{\sqrt{3}+5}\)

\(=\left(\dfrac{2\left(\sqrt{3}+1\right)}{2}-\dfrac{3\left(2+\sqrt{3}\right)}{1}+\dfrac{15\left(3+\sqrt{3}\right)}{6}\right)\cdot\dfrac{1}{\sqrt{3}+5}\)

\(=\left(\sqrt{3}+1-2-\sqrt{3}+\dfrac{5\left(3+\sqrt{3}\right)}{2}\right)\cdot\dfrac{1}{\sqrt{3}+5}\)

\(=\left(-1+\dfrac{5\left(3+\sqrt{3}\right)}{2}\right)\cdot\dfrac{1}{5+\sqrt{3}}\)

\(=\dfrac{-2+15+5\sqrt{3}}{2\left(5+\sqrt{3}\right)}\)

\(=\dfrac{13+5\sqrt{3}}{10+2\sqrt{3}}\)