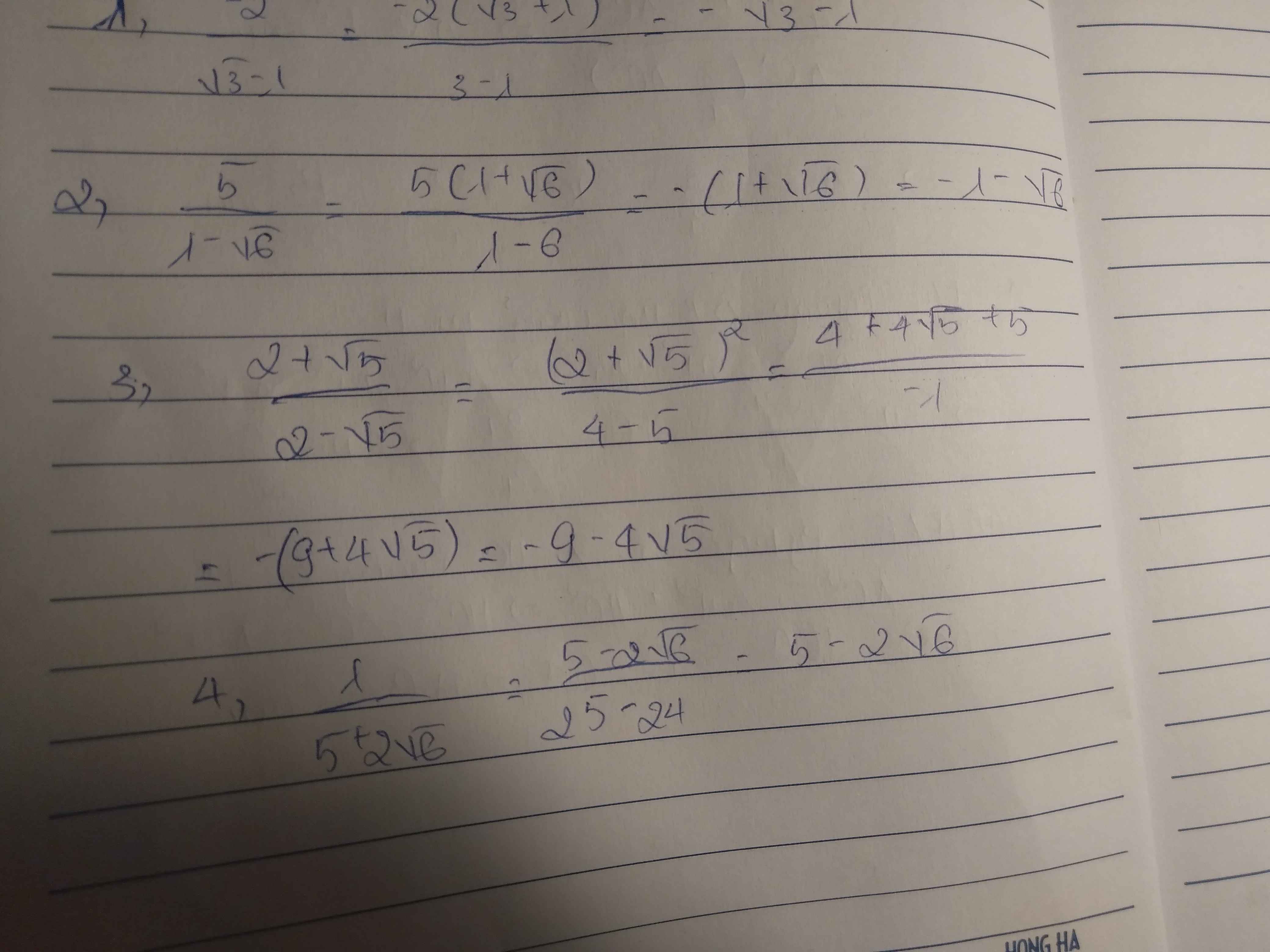Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1) \(\dfrac{\sqrt{5}+2}{\sqrt{5}-2}=9+4\sqrt{5}\)
2) \(\dfrac{5\sqrt{2}-2\sqrt{5}}{\sqrt{2}-\sqrt{5}}=\dfrac{\sqrt{10}\left(\sqrt{5}-\sqrt{2}\right)}{-\left(\sqrt{5}-\sqrt{2}\right)}=-\sqrt{10}\)
3) \(\dfrac{\sqrt{20}-3\sqrt{10}}{3-\sqrt{5}}=\dfrac{\sqrt{10}\left(\sqrt{5}-3\right)}{-\left(\sqrt{5}-3\right)}=-\sqrt{10}\)
4) \(\dfrac{6-2\sqrt{5}}{3+\sqrt{5}}=\dfrac{\left(6-2\sqrt{5}\right)\left(3-\sqrt{5}\right)}{4}=\dfrac{18-6\sqrt{5}-6\sqrt{5}+10}{4}=\dfrac{28-12\sqrt{5}}{4}=7-3\sqrt{5}\)
5)\(\dfrac{9+4\sqrt{5}}{\sqrt{5}+2}=\sqrt{5}+2\)

\(\dfrac{\sqrt{6}-\sqrt{3}}{\sqrt{2}-1}+\dfrac{3+\sqrt{3}}{\sqrt{3}+1}+\dfrac{2}{\sqrt{2}+1}-\dfrac{4}{\sqrt{2}}\)
\(=\dfrac{\sqrt{3}\left(\sqrt{2}-1\right)}{\sqrt{2}-1}+\dfrac{\sqrt{3}\left(\sqrt{3}+1\right)}{\sqrt{3}+1}+\dfrac{2\sqrt{2}}{2+\sqrt{2}}-\dfrac{4\sqrt{2}+4}{2+\sqrt{2}}\)
\(=\sqrt{3}+\sqrt{3}+\dfrac{-2\sqrt{2}-4}{2+\sqrt{2}}\)
\(=2\sqrt{3}+\dfrac{-2\left(2+\sqrt{2}\right)}{2+\sqrt{2}}\)
\(=2\sqrt{3}-2\)
\(------\)
\(\dfrac{4}{\sqrt{5}+1}+\dfrac{5}{\sqrt{5}+2}+\dfrac{5}{\sqrt{5}+3}\)
\(=\dfrac{4\left(\sqrt{5}-1\right)}{\left(\sqrt{5}+1\right)\left(\sqrt{5}-1\right)}+\dfrac{5\left(\sqrt{5}-2\right)}{\left(\sqrt{5}+2\right)\left(\sqrt{5}-2\right)}+\dfrac{5\left(\sqrt{5}-3\right)}{\left(\sqrt{5}+3\right)\left(\sqrt{5}-3\right)}\)
\(=\dfrac{4\sqrt{5}-4}{5-1}+\dfrac{5\sqrt{5}-10}{5-4}+\dfrac{5\sqrt{5}-15}{5-9}\)
\(=5\sqrt{5}-10+\left(\dfrac{4\sqrt{5}-4}{4}+\dfrac{5\sqrt{5}-15}{-4}\right)\)
\(=\dfrac{4\cdot\left(5\sqrt{5}-10\right)}{4}+\left(\dfrac{4\sqrt{5}-4}{4}-\dfrac{5\sqrt{5}-15}{4}\right)\)
\(=\dfrac{20\sqrt{5}-40}{4}+\dfrac{-\sqrt{5}+11}{4}\)
\(=\dfrac{19\sqrt{5}-29}{4}\)
#Ayumu

bạn nên tự nghiên cứu rồi giải đi chứ bạn đưa 1 loạt thế thì ai rảnh mà giải, với lại cứ bài gì không biết chưa chịu suy nghĩ đã hỏi rồi thì tiến bộ sao được, đúng không

5: Ta có: \(\dfrac{2-\sqrt{2}}{1-\sqrt{2}}+\dfrac{\sqrt{2}-\sqrt{6}}{\sqrt{3}-1}\)
\(=-\sqrt{2}-\sqrt{2}\)
\(=-2\sqrt{2}\)

\(2,\\ a,PT\Leftrightarrow\sqrt{\left(5x-1\right)^2}=\sqrt{4\left(x+1\right)^2}\\ \Leftrightarrow\left|5x-1\right|=2\left|x+1\right|\\ \Leftrightarrow\left[{}\begin{matrix}5x-1=2\left(x+1\right)\\1-5x=2\left(x+1\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=3\\7x=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{1}{7}\end{matrix}\right.\)
\(b,ĐK:x^2-3\ge0\\ PT\Leftrightarrow\sqrt{x^2-3}=x-1\\ \Leftrightarrow x^2-3=x^2-2x+1\\ \Leftrightarrow2x=4\Leftrightarrow x=2\left(tm\right)\\ c,ĐK:x\le\dfrac{7}{2}\\ PT\Leftrightarrow7-2x=x^2+7\\ \Leftrightarrow x^2+2x=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=2\left(tm\right)\end{matrix}\right.\\ d,ĐK:x\ge3\\ PT\Leftrightarrow3\sqrt{x-3}+\dfrac{1}{2}\cdot2\sqrt{x-3}-9\cdot\dfrac{1}{3}\sqrt{x-3}=2\\ \Leftrightarrow\sqrt{x-3}=2\\ \Leftrightarrow x-3=4\Leftrightarrow x=7\left(tm\right)\)

4: Ta có: \(\dfrac{6}{1-\sqrt{3}}-\dfrac{3\sqrt{3}+3}{\sqrt{3}+1}\)
\(=-3-3\sqrt{3}-3\)
\(=-6-3\sqrt{3}\)

1: ta có: \(\dfrac{1}{3-2\sqrt{2}}+\dfrac{1}{\sqrt{5}+2}\)
\(=3+2\sqrt{2}+\sqrt{5}-2\)
\(=2\sqrt{2}+\sqrt{5}+1\)
2: Ta có: \(\dfrac{1}{3-2\sqrt{2}}-\dfrac{1}{3+2\sqrt{2}}\)
\(=3+2\sqrt{2}-3+2\sqrt{2}\)
\(=4\sqrt{2}\)