Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: \(\Leftrightarrow2\cdot\cos2x\cdot\cos x+2\cdot\sin x\cdot\cos2x=\sqrt{2}\cdot\cos2x\)
\(\Leftrightarrow2\cdot\cos2x\left(\sin x+\cos x\right)=\sqrt{2}\cdot\cos2x\)
\(\Leftrightarrow\sqrt{2}\cdot\cos2x\cdot\left[\sqrt{2}\cdot\sqrt{2}\cdot\sin\left(x+\dfrac{\Pi}{4}\right)-1\right]=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\cos2x=0\\\sin\left(x+\dfrac{\Pi}{4}\right)=\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\Pi}{2}+k\Pi\\x+\dfrac{\Pi}{4}=\dfrac{\Pi}{6}+k2\Pi\\x+\dfrac{\Pi}{4}=\dfrac{5}{6}\Pi+k2\Pi\end{matrix}\right.\)
\(\Leftrightarrow x\in\left\{\dfrac{\Pi}{4}+\dfrac{k\Pi}{2};\dfrac{-1}{12}\Pi+k2\Pi;\dfrac{7}{12}\Pi+k2\Pi\right\}\)
c: \(\Leftrightarrow2\cdot\sin2x\cdot\cos x+\sin2x=2\cdot\cos2x\cdot\cos x+\cos2x\)
\(\Leftrightarrow\sin2x\left(2\cos x+1\right)=\cos2x\left(2\cos x+1\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}\sin2x=\cos2x=\sin\left(\dfrac{\Pi}{2}-2x\right)\\\cos x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Pi}{8}+\dfrac{k\Pi}{4}\\\\x=-\dfrac{2}{3}\Pi+k2\Pi\\x=\dfrac{2}{3}\Pi+k2\Pi\end{matrix}\right.\)

1.
\(sinx-\sqrt{2}cos3x=\sqrt{3}cosx+\sqrt{2}sin3x\)
\(\Leftrightarrow sinx-\sqrt{3}cosx=\sqrt{2}cos3x+\sqrt{2}sin3x\)
\(\Leftrightarrow\dfrac{1}{2}sinx-\dfrac{\sqrt{3}}{2}cosx=\dfrac{1}{\sqrt{2}}cos3x+\dfrac{1}{\sqrt{2}}sin3x\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{3}\right)=sin\left(3x+\dfrac{\pi}{4}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{3}=3x+\dfrac{\pi}{4}+k2\pi\\x-\dfrac{\pi}{3}=\pi-3x-\dfrac{\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{7\pi}{24}-k\pi\\x=-\dfrac{3}{4}x+\dfrac{13\pi}{48}+\dfrac{k\pi}{2}\end{matrix}\right.\)
Vậy phương trình đã cho có nghiệm \(x=-\dfrac{7\pi}{24}-k\pi;x=-\dfrac{3}{4}x+\dfrac{13\pi}{48}+\dfrac{k\pi}{2}\)
2.
\(sinx-\sqrt{3}cosx=2sin5\text{}x\)
\(\Leftrightarrow\dfrac{1}{2}sinx-\dfrac{\sqrt{3}}{2}cosx=sin5x\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{3}\right)=sin5x\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{3}=5x+k2\pi\\x-\dfrac{\pi}{3}=\pi-5x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{12}-\dfrac{k\pi}{2}\\x=\dfrac{2\pi}{9}+\dfrac{k\pi}{3}\end{matrix}\right.\)
Vậy phương trình đã cho có nghiệm \(x=-\dfrac{\pi}{12}-\dfrac{k\pi}{2};x=\dfrac{2\pi}{9}+\dfrac{k\pi}{3}\)

ĐKXĐ: ...
\(sin3x-cos3x+sinx+cosx=\dfrac{sin3x-cos3x+sinx+cosx}{\left(sin3x+cosx\right)\left(cos3x-sinx\right)}\)
\(\Rightarrow\left[{}\begin{matrix}sin3x-cos3x+sinx+cosx=0\left(1\right)\\\left(sin3x+cosx\right)\left(cos3x-sinx\right)=1\left(2\right)\end{matrix}\right.\)
(1) \(\Leftrightarrow3sinx-4sin^3x-4cos^3x+3cosx+sinx+cosx=0\)
\(\Leftrightarrow sinx+cosx+sin^3x+cos^3x=0\)
\(\Leftrightarrow sinx+cosx+\left(sinx+cosx\right)\left(1-sinx.cosx\right)=0\)
\(\Leftrightarrow\left(sinx+cosx\right)\left(2-sinx.cosx\right)=0\)
\(\Leftrightarrow sinx+cosx=0\) (loại)
(2) \(\Leftrightarrow sin3x.cos3x-sinx.cosx-sin3x.sinx+cos3x.cosx=1\)
\(\Leftrightarrow\dfrac{1}{2}sin6x-\dfrac{1}{2}sin2x+cos4x=1\)
\(\Leftrightarrow\dfrac{1}{2}\left(3sin2x-4sin^32x\right)-\dfrac{1}{2}sin2x+1-2sin^22x=1\)
\(\Leftrightarrow sin2x-2sin^32x-2sin^22x=0\)
\(\Leftrightarrow-sin2x\left(2sin^22x+2sin2x-1\right)=0\)
\(\Leftrightarrow...\)

\(sin3x-cos3x=\left(3sinx-4sin^3x\right)-\left(4cos^3x-3cosx\right)\)
\(=3\left(sinx+cosx\right)-4\left(sin^3x+cos^3x\right)\)
\(=2\left(sin^3x+cos^3x\right)-6\left(sin^3x+cos^3x\right)+3\left(sinx+cosx\right)\)
\(=2\left(sin^3x+cos^3x\right)-6\left(sinx+cosx\right)\left(1-sinx.cosx\right)+3\left(sinx+cosx\right)\)
\(=2\left(sin^3x+cos^3x\right)-3\left(sinx+cosx\right)\left(1-2sinx.cosx\right)\)
\(=2\left(sin^3x+cos^3x\right)+6sinx.cosx\left(sinx+cosx\right)-3\left(sinx+cosx\right)\)
\(=2\left(sinx+cosx\right)^3-3\left(sinx+cosx\right)\) (đpcm)

Chọn D
Ta sẽ biến đổi phương trình thành dạng tích
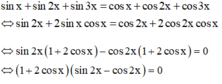
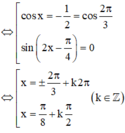
Chú ý: có thể dùng 4 đáp án thay vào phương trình để kiểm tra đâu là nghiệm
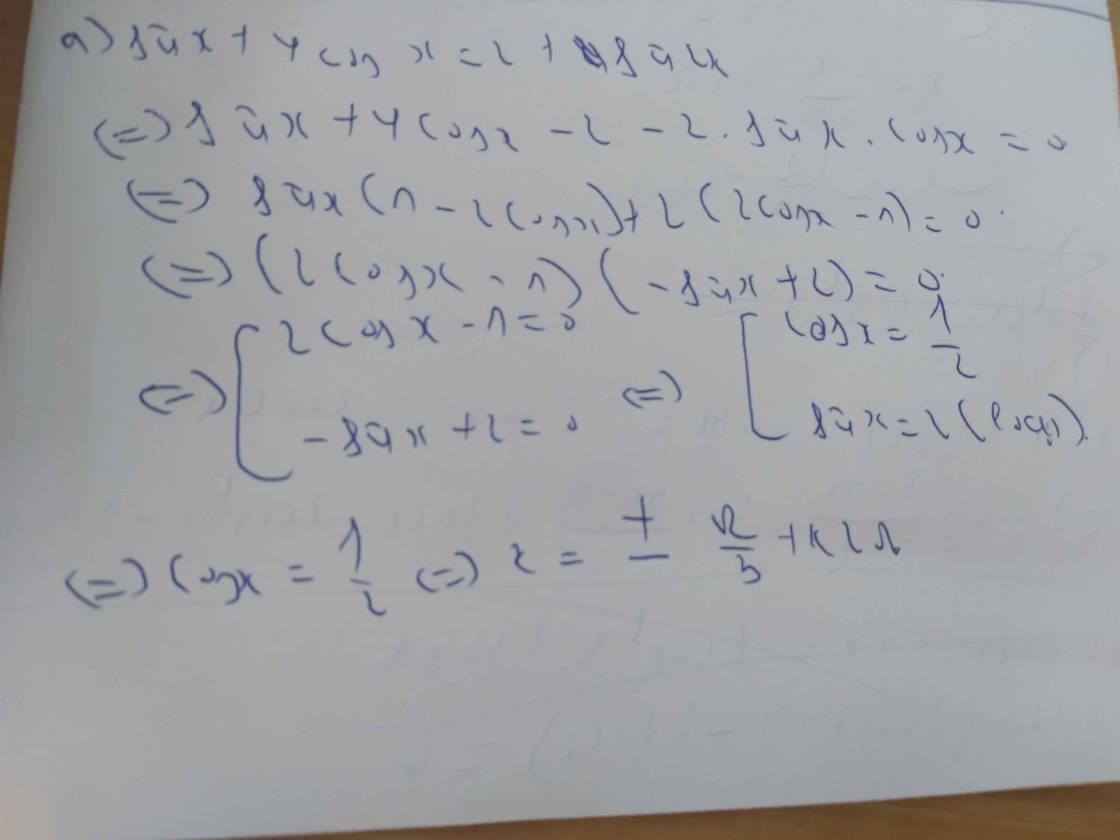

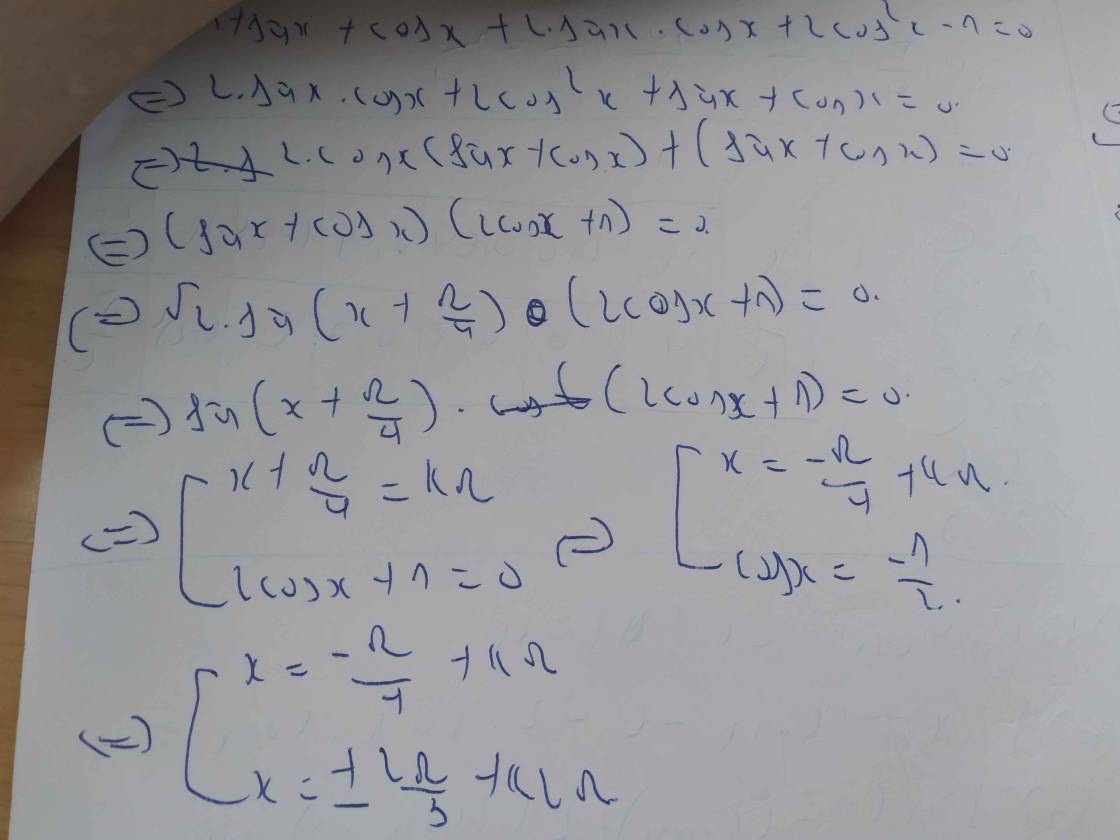



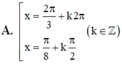
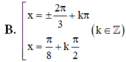
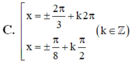
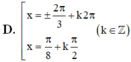
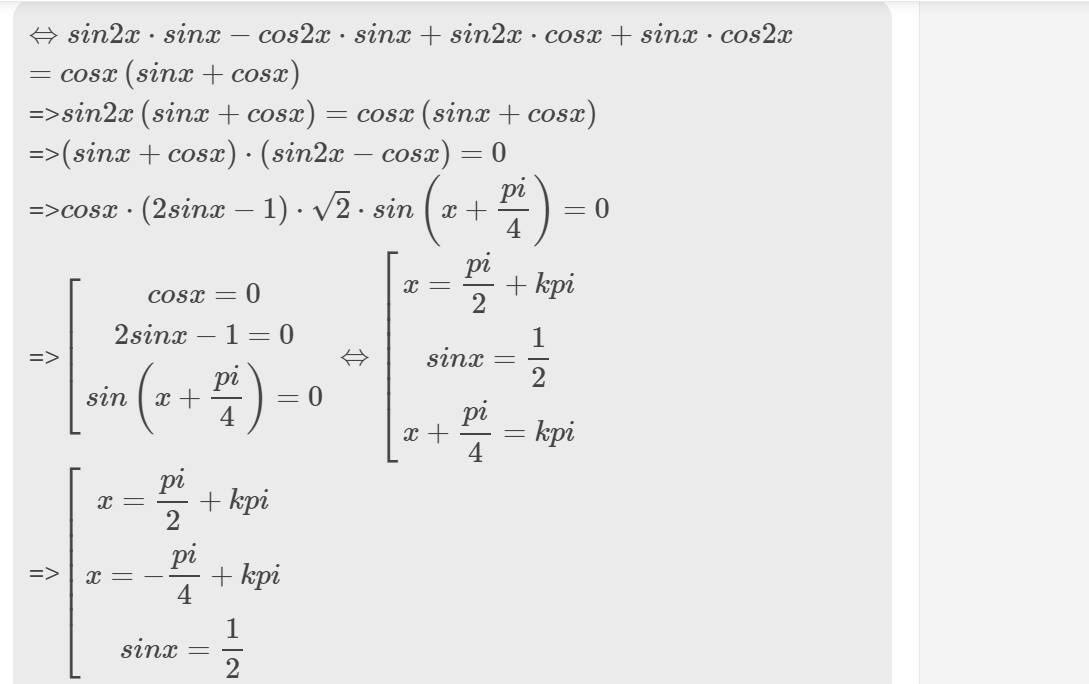

câu 1:xét sinx=o
xét sinx khác 0
chia phương trình cho cos3x
ta được 1 phương trình mới:
4+3tanx-\(\frac{1}{sin^2x}\)-tan3x=0
<=>4+3tanx-(1+cot2x)-tan3x=0
<=>4+3tanx-1-\(\frac{1}{tan^2x}\)-tan3x=o
nhân cho tan2x ta được 1 phương trình bậc 5 với tanx