
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


9)Ta có: \(\sqrt{x^2-6x+9}=5\)
\(\Leftrightarrow\sqrt{\left(x-3\right)^2}=5\)
\(\Leftrightarrow\left|x-3\right|=5\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=5\\x-3=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-2\end{matrix}\right.\)
Vậy: S={8;-2}
19) Ta có: \(\sqrt[3]{x^3+9x^2}=x+3\)
\(\Leftrightarrow x^3+9x^2=\left(x+3\right)^3\)
\(\Leftrightarrow x^3+9x^2=x^3+9x^2+27x+27\)
\(\Leftrightarrow27x=-27\)
hay x=-1
Vậy: S={-1}

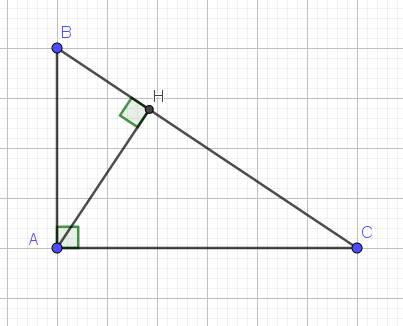
ta có sinB=\(\dfrac{AH}{AB}\)\(\Rightarrow\)AH=AB.sinB=3,6.sin62=3,18
BH=\(\sqrt{AB^2-AH^2}\)(pytago)=\(\sqrt{3,6^2-3,18^2}\)=1,69
\(_{\widehat{C}}\)=90-\(\widehat{B}\)=90-62=28\(^0\)
sinC=\(\dfrac{AB}{BC}\)\(\Rightarrow\)BC=\(\dfrac{AB}{sinC}\)=\(\dfrac{3,6}{sin28}\)=7,67
mà:CH=BC-BH=7,67-1,69=5,98
AC=\(\sqrt{BC^2-AB^2}\)(pytago)=\(\sqrt{7,67^2-3,6^2}\)=6.77

Lời giải:
Áp dụng định lý Pitago:
$HC=\sqrt{AC^2-AH^2}=\sqrt{8^2-4,8^2}=6,4$ (cm)
Áp dụng hệ thức lượng trong tam giác vuông:
$BH.CH=AH^2$
$\Rightarrow BH=\frac{AH^2}{CH}=\frac{4,8^2}{6,4}=3,6$ (cm)
$BC=BH+CH=3,6+6,4=10$ (cm)
$AB=\sqrt{BC^2-AC^2}=\sqrt{10^2-8^2}=6$ (cm) - Theo định lý Pitago

\(P=x-2\sqrt{2x-3}\) (ĐK: \(x\ge\dfrac{3}{2}\) )
\(2P=2x-4\sqrt{2x-3}\)
\(2P=2x-3-4\sqrt{2x-3}+4-1\)
\(2P=\left(\sqrt{2x-3}-2\right)^2-1\) \(\ge-1\)
\(\Rightarrow P\ge\dfrac{-1}{2}\)
Dấu bằng xảy ra khi :
\(\sqrt{2x-3}=2\Leftrightarrow x=\dfrac{7}{2}\) (nhận)
Vậy .................
\(P=x-2\sqrt{2x-3}\) (ĐK: \(x\ge\dfrac{3}{2}\) )
\(2P=2x-4\sqrt{2x-3}\)
\(2P=2x-3-4\sqrt{2x-3}+4-1\)
\(2P=\left(\sqrt{2x-3}-2\right)^2-1\) \(\ge-1\)
\(\Rightarrow P\ge\dfrac{-1}{2}\)
Dấu bằng xảy ra khi :
\(\sqrt{2x-3}=2\Leftrightarrow x=\dfrac{7}{2}\) (nhận)
Vậy .................




a: Ta có: \(A=\dfrac{2x-3\sqrt{x}-14}{x-7\sqrt{x}+12}-\dfrac{\sqrt{x}+4}{\sqrt{x}-3}-\dfrac{\sqrt{x}-1}{\sqrt{x}-4}\)
\(=\dfrac{2x-3\sqrt{x}-14-x+16-x+4\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\)
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\)
Ta có: \(B=\dfrac{x-2\sqrt{x}+1}{x-4\sqrt{x}+3}\)
\(=\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}-3}\)
b: Ta có: M=A:B
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}-1}\)
\(=\dfrac{1}{\sqrt{x}-4}\)

\(a,A=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1-3\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ A=\dfrac{2x-3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{\left(\sqrt{x}-1\right)\left(2\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}-1}{\sqrt{x}+1}\\ b,A=\dfrac{2\left(\sqrt{x}+1\right)-3}{\sqrt{x}+1}=2-\dfrac{3}{\sqrt{x}+1}\in Z\\ \Leftrightarrow\sqrt{x}+1\inƯ\left(3\right)=\left\{1;3\right\}\left(\sqrt{x}+1\ge1\right)\\ \Leftrightarrow\sqrt{x}\in\left\{0;2\right\}\\ \Leftrightarrow x\in\left\{0;4\right\}\left(tm\right)\)
a) \(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}-\dfrac{3\sqrt{x}+1}{x-1}\)
\(\Rightarrow A=\dfrac{\left(\sqrt{x}+1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{x+2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{x-2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1-3\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{2x-3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{\left(2x-2\sqrt{x}\right)-\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{2\sqrt{x}\left(\sqrt{x}-1\right)-\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{\left(2\sqrt{x}-1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow A=\dfrac{2\sqrt{x}-1}{\sqrt{x}+1}\)





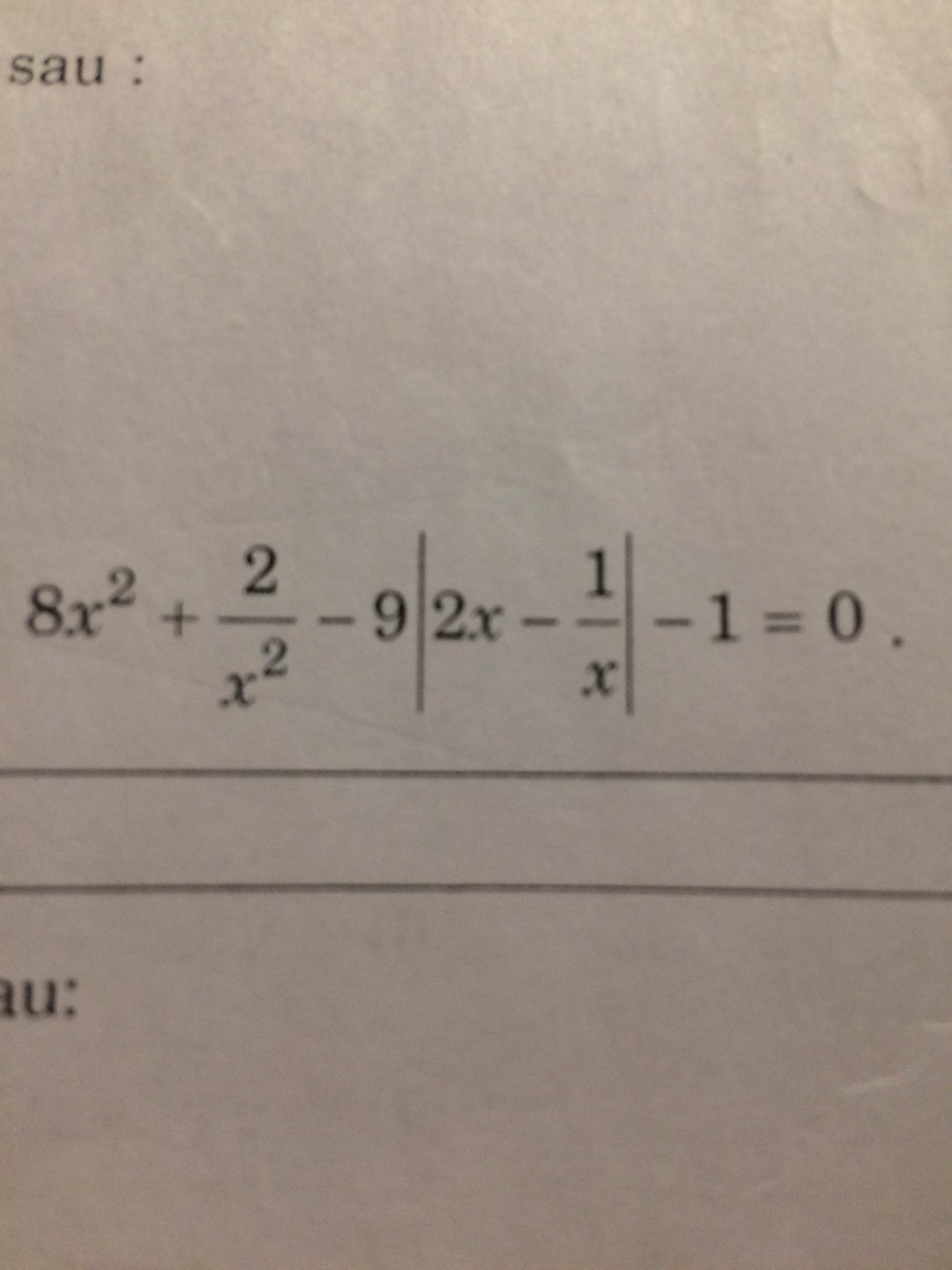
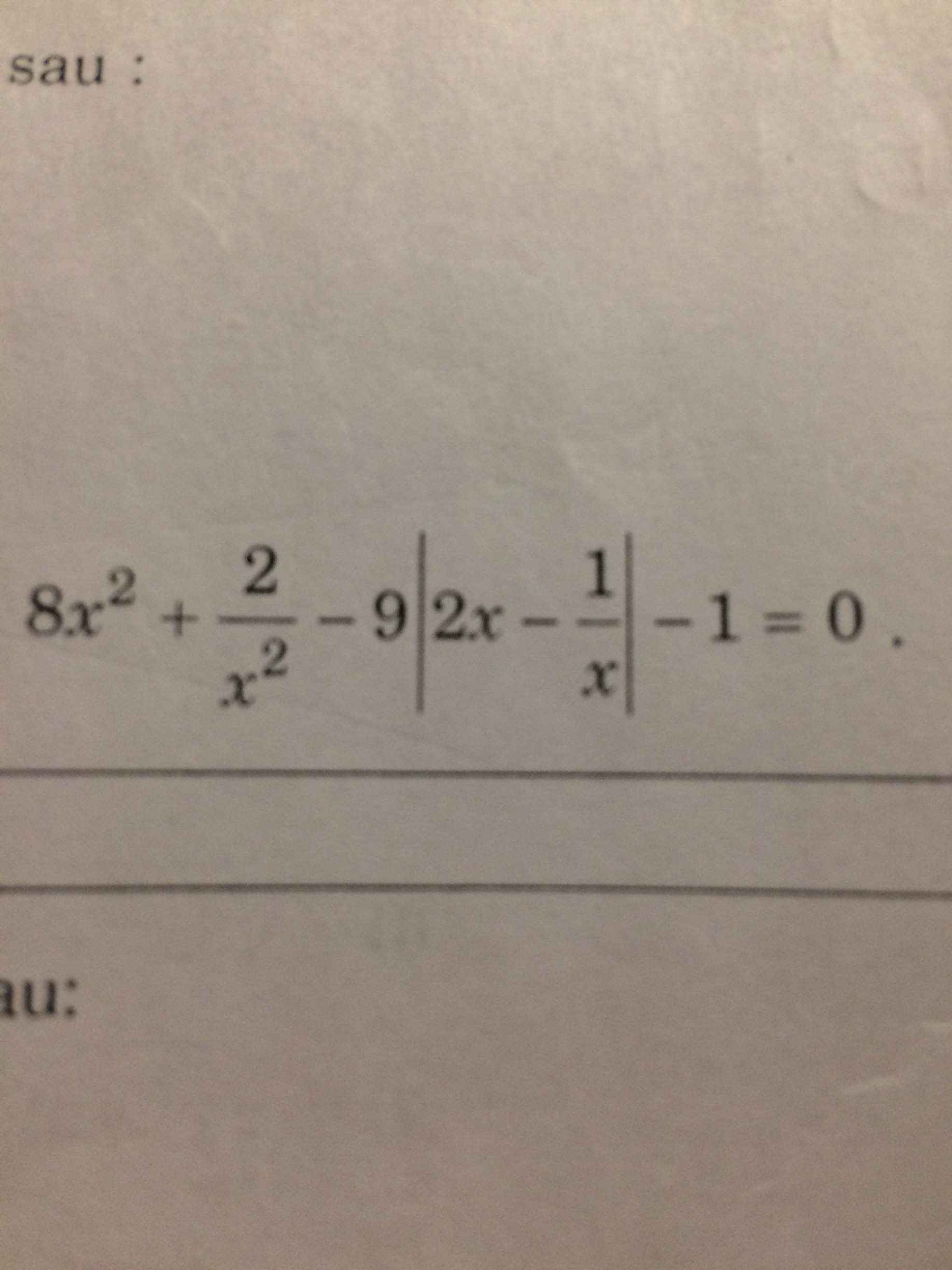


\(ab\left(2023-\dfrac{ab}{2}\right)=\dfrac{a^4+b^4}{4}-2024\ge\dfrac{2\sqrt{a^4b^4}}{4}-2024\)
\(\Rightarrow ab\left(2023-\dfrac{ab}{2}\right)\ge\dfrac{a^2b^2}{2}-2024\)
\(\Rightarrow2023ab-\dfrac{a^2b^2}{2}\ge\dfrac{a^2b^2}{2}-2024\)
\(\Rightarrow a^2b^2-2023ab-2024\le0\)
\(\Rightarrow\left(ab+1\right)\left(ab-2024\right)\le0\)
\(\Rightarrow-1\le ab\le2024\)
\(P_{max}=2024\) khi \(a=b=\sqrt{2024}\)
\(P_{min}=-1\) khi \(\left(a;b\right)=\left(1;-1\right);\left(-1;1\right)\)