Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(sigma\frac{a}{1+b-a}=sigma\frac{a^2}{a+ab-a^2}\ge\frac{\left(a+b+c\right)^2}{a+b+c+\frac{\left(a+b+c\right)^2}{3}-\frac{\left(a+b+c\right)^2}{3}}=1\)
Dấu "=" xảy ra khi \(a=b=c=\frac{1}{3}\)
\(\frac{1}{b^2+c^2}=\frac{1}{1-a^2}=1+\frac{a^2}{b^2+c^2}\le1+\frac{a^2}{2bc}\)
Tương tự cộng lại quy đồng ta có đpcm
Dấu "=" xảy ra khi \(a=b=c=\frac{1}{\sqrt{3}}\)

\(\Sigma\frac{b+1}{8-\sqrt{a}}\le\Sigma\frac{2\left(b+1\right)}{15-a}=\Sigma\frac{2\left(a+2b+c\right)}{4a+5b+5c}\)(AM-gm)
Đặt \(\left\{\begin{matrix}x=4a+5b+5c\\y=4b+5a+5c\\z=4c+5a+5b\end{matrix}\right.\)suy ra...

Đề bài hình như bị sai em, thay điểm rơi ko thỏa mãn
Biểu thức là \(a+b+\sqrt{2\left(a+c\right)}\) mới đúng

Đặt \(a\left(1-b\right)=x;b\left(1-c\right)=y;c\left(1-a\right)=x\)
\(\Rightarrow1-\left(a+b+c\right)+ab+bc+ca=1-a\left(1-b\right)-b\left(1-c\right)-c\left(1-a\right)=1-x-y-z\)
BĐT cần c/m trở thành:
\(\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}\ge\dfrac{3}{1-x-y-z}\)
\(\Leftrightarrow\left(1-x-y-z\right)\left(\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}\right)-3\ge0\)
\(\Leftrightarrow\dfrac{1-x-y-z}{x}+\dfrac{1-x-y-z}{y}+\dfrac{1-x-y-z}{z}-3\ge0\)
\(\Leftrightarrow\dfrac{1-y-z}{x}+\dfrac{1-z-x}{y}+\dfrac{1-x-y}{z}-6\ge0\) (1)
Lại có: \(1-y-z=1-b\left(1-c\right)-c\left(1-a\right)=1-b-c+bc+ca=\left(1-b\right)\left(1-c\right)+ca\)
Nên (1) tương đương:
\(\dfrac{\left(1-b\right)\left(1-c\right)+ca}{a\left(1-b\right)}+\dfrac{\left(1-a\right)\left(1-c\right)+ab}{b\left(1-c\right)}+\dfrac{\left(1-a\right)\left(1-b\right)+bc}{c\left(1-a\right)}-6\ge0\)
\(\Leftrightarrow\dfrac{1-c}{a}+\dfrac{c}{1-b}+\dfrac{1-a}{b}+\dfrac{a}{1-c}+\dfrac{1-b}{c}+\dfrac{b}{1-a}\ge6\)
BĐT trên hiển nhiên đúng theo AM-GM do:
\(\dfrac{1-c}{a}+\dfrac{c}{1-b}+\dfrac{1-a}{b}+\dfrac{a}{1-c}+\dfrac{1-b}{c}+\dfrac{b}{1-a}\ge6\sqrt[6]{\dfrac{abc\left(1-a\right)\left(1-b\right)\left(1-c\right)}{abc\left(1-a\right)\left(1-b\right)\left(1-c\right)}}=6\) (đpcm)
Dấu "=" xảy ra khi \(a=b=c=\dfrac{1}{2}\)
Cám ơn bài giải của thầy Lâm ạ!
Và từ bài bất đăng thức này, đã được chế thành bài toán hình học trong 1 kì thi học sinh giỏi toán cấp tỉnh thầy ạ!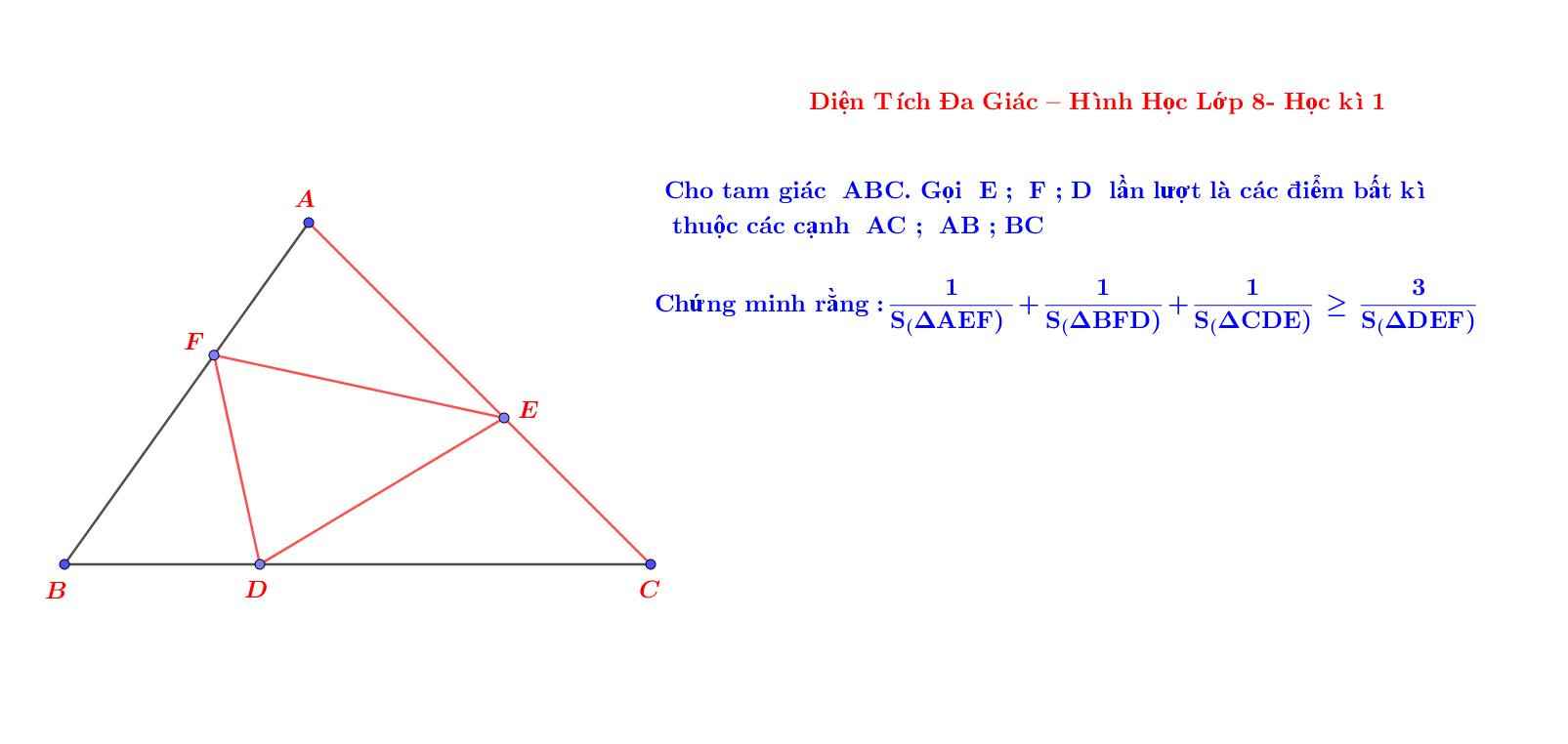

Bài 1:
Áp dụng BĐT Bunhiacopxky ta có:
$(a^2+b^2+c^2)(1+1+1)\geq (a+b+c)^2$
$\Leftrightarrow 3(a^2+b^2+c^2)\geq 1$
$\Leftrightarrow a^2+b^2+c^2\geq \frac{1}{3}$ (đpcm)
Dấu "=" xảy ra khi $a=b=c=\frac{1}{3}$
Bài 2:
Áp dụng BĐT Bunhiacopxky:
$(a^2+4b^2+9c^2)(1+\frac{1}{4}+\frac{1}{9})\geq (a+b+c)^2$
$\Leftrightarrow 2015.\frac{49}{36}\geq (a+b+c)^2$
$\Leftrightarrow \frac{98735}{36}\geq (a+b+c)^2$
$\Rightarrow a+b+c\leq \frac{7\sqrt{2015}}{6}$ chứ không phải $\frac{\sqrt{14}}{6}$ :''>>

\(\dfrac{1}{a^3}+a\ge2\sqrt{\dfrac{a}{a^3}}=\dfrac{2}{a}\) ; \(\dfrac{1}{b^3}+b\ge\dfrac{2}{b}\) ; \(\dfrac{1}{c^3}+c\ge\dfrac{2}{c}\)
\(\Rightarrow\dfrac{1}{a^3}+\dfrac{1}{b^3}+\dfrac{1}{c^3}+a+b+c\ge2\left(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\right)\) (1)
Lại có \(\dfrac{4a}{a^4+1}\le\dfrac{4a}{2\sqrt{a^4}}=\dfrac{4a}{2a^2}=\dfrac{2}{a}\)
Tương tự \(\dfrac{4b}{b^4+1}\le\dfrac{2}{b}\) ; \(\dfrac{4c}{c^4+1}\le\dfrac{2}{c}\)
\(\Rightarrow4\left(\dfrac{a}{a^4+1}+\dfrac{b}{b^4+1}+\dfrac{c}{c^4+1}\right)\le2\left(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\right)\) (2)
Từ (1),(2)\(\Rightarrow\dfrac{1}{a^3}+\dfrac{1}{b^3}+\dfrac{1}{c^3}+a+b+c\ge4\left(\dfrac{a}{a^4+1}+\dfrac{b}{b^4+1}+\dfrac{c}{c^4+1}\right)\)
Dấu "=" xảy ra khi a=b=c=1

1. Đề thiếu
2. BĐT cần chứng minh tương đương:
\(a^4+b^4+c^4\ge abc\left(a+b+c\right)\)
Ta có:
\(a^4+b^4+c^4\ge\dfrac{1}{3}\left(a^2+b^2+c^2\right)^2\ge\dfrac{1}{3}\left(ab+bc+ca\right)^2\ge\dfrac{1}{3}.3abc\left(a+b+c\right)\) (đpcm)
3.
Ta có:
\(\left(a^6+b^6+1\right)\left(1+1+1\right)\ge\left(a^3+b^3+1\right)^2\)
\(\Rightarrow VT\ge\dfrac{1}{\sqrt{3}}\left(a^3+b^3+1+b^3+c^3+1+c^3+a^3+1\right)\)
\(VT\ge\sqrt{3}+\dfrac{2}{\sqrt{3}}\left(a^3+b^3+c^3\right)\)
Lại có:
\(a^3+b^3+1\ge3ab\) ; \(b^3+c^3+1\ge3bc\) ; \(c^3+a^3+1\ge3ca\)
\(\Rightarrow2\left(a^3+b^3+c^3\right)+3\ge3\left(ab+bc+ca\right)=9\)
\(\Rightarrow a^3+b^3+c^3\ge3\)
\(\Rightarrow VT\ge\sqrt{3}+\dfrac{6}{\sqrt{3}}=3\sqrt{3}\)
4.
Ta có:
\(a^3+1+1\ge3a\) ; \(b^3+1+1\ge3b\) ; \(c^3+1+1\ge3c\)
\(\Rightarrow a^3+b^3+c^3+6\ge3\left(a+b+c\right)=9\)
\(\Rightarrow a^3+b^3+c^3\ge3\)
5.
Ta có:
\(\dfrac{a}{b}+\dfrac{b}{c}\ge2\sqrt{\dfrac{a}{c}}\) ; \(\dfrac{a}{b}+\dfrac{c}{a}\ge2\sqrt{\dfrac{c}{b}}\) ; \(\dfrac{b}{c}+\dfrac{c}{a}\ge2\sqrt{\dfrac{b}{a}}\)
\(\Rightarrow\sqrt{\dfrac{b}{a}}+\sqrt{\dfrac{c}{b}}+\sqrt{\dfrac{a}{c}}\le\dfrac{a}{b}+\dfrac{b}{c}+\dfrac{c}{a}=1\)

Bài 1:
Ta có:
\(\text{VT}=\frac{a^2}{a+2b^2}+\frac{b^2}{b+2c^2}+\frac{c^2}{c+2a^2}\)
\(=a-\frac{2ab^2}{a+2b^2}+b-\frac{2bc^2}{b+2c^2}+c-\frac{2ca^2}{c+2a^2}=(a+b+c)-2\left(\frac{ab^2}{a+2b^2}+\frac{bc^2}{b+2c^2}+\frac{ca^2}{c+2a^2}\right)\)
\(=3-2M(*)\)
Áp dụng BĐT Cauchy ta có:
\(M=\frac{ab^2}{a+b^2+b^2}+\frac{bc^2}{b+c^2+c^2}+\frac{ca^2}{c+a^2+a^2}\leq \frac{ab^2}{3\sqrt[3]{ab^4}}+\frac{bc^2}{3\sqrt[3]{bc^4}}+\frac{ca^2}{3\sqrt[3]{ca^4}}\)
\(\Leftrightarrow M\leq \frac{1}{3}(\sqrt[3]{a^2b^2}+\sqrt[3]{b^2c^2}+\sqrt[3]{c^2a^2})\)
Tiếp tục áp dụng BĐT Cauchy:
\(\sqrt[3]{a^2b^2}+\sqrt[3]{b^2c^2}+\sqrt[3]{c^2a^2}\leq \frac{ab+ab+1}{3}+\frac{bc+bc+1}{3}+\frac{ca+ca+1}{3}=\frac{2(ab+bc+ac)+3}{3}\)
Mà \(ab+bc+ac\leq \frac{(a+b+c)^2}{3}=3\) (quen thuộc)
\(\Rightarrow M\leq \frac{1}{3}.\frac{2.3+3}{3}=1(**)\)
Từ \((*);(**)\Rightarrow \text{VT}\geq 3-2.1=1\)
(đpcm)
Dấu bằng xảy ra khi $a=b=c=1$
Bài 2:
Áp dụng BĐT Cauchy -Schwarz:
\(\text{VT}=\frac{a^3}{a^2+a^2b^2}+\frac{b^3}{b^2+b^2c^2}+\frac{c^3}{c^2+a^2c^2}\geq \frac{(a\sqrt{a}+b\sqrt{b}+c\sqrt{c})^2}{a^2+a^2b^2+b^2+b^2c^2+c^2+c^2a^2}\)
hay:
\(\text{VT}\geq \frac{(a\sqrt{a}+b\sqrt{b}+c\sqrt{c})^2}{1+a^2b^2+b^2c^2+c^2a^2}(*)\)
Mặt khác, theo BĐT Cauchy ta dễ thấy:
\(a^4+b^4+c^4\geq a^2b^2+b^2c^2+c^2a^2\)
\(\Rightarrow (a^2+b^2+c^2)^2\geq 3(a^2b^2+b^2c^2+c^2a^2)\)
\(\Leftrightarrow 1\geq 3(a^2b^2+b^2c^2+c^2a^2)\Rightarrow a^2b^2+b^2c^2+c^2a^2\leq \frac{1}{3}(**)\)
Từ \((*);(**)\Rightarrow \text{VT}\geq \frac{(a\sqrt{a}+b\sqrt{b}+c\sqrt{c})^2}{1+\frac{1}{3}}=\frac{3}{4}(a\sqrt{a}+b\sqrt{b}+c\sqrt{c})^2\)
Ta có đpcm
Dấu bằng xảy ra khi \(a=b=c=\frac{1}{\sqrt{3}}\)
Bài 1. Mình nghĩ đề bài của bạn nhầm ở chỗ dấu "\(\ge\)" , bạn sửa lại thành "\(\le\)" nhé ^^
Áp dụng bất đẳng thức Bunhiacopxki : \(9=3\left(a+b+c\right)=\left(1^2+1^2+1^2\right)\left[\left(\sqrt{a}\right)^2+\left(\sqrt{b}\right)^2+\left(\sqrt{c}\right)^2\right]\ge\left(\sqrt{a}+\sqrt{b}+\sqrt{c}\right)^2\)
\(\Rightarrow\left(\sqrt{a}+\sqrt{b}+\sqrt{c}\right)^2\le9\Leftrightarrow\sqrt{a}+\sqrt{b}+\sqrt{c}\le3\)
\(\Leftrightarrow\sqrt{a}+\sqrt{b}+\sqrt{c}\le a+b+c\) (vì a+b+c = 3)
Bài 2.
Để chứng minh bất đẳng thức trên ta biến đổi : \(a+b+c=1\Leftrightarrow a+1=\left(1-b\right)+\left(1-c\right)\)
Tương tự : \(b+1=\left(1-a\right)+\left(1-c\right)\) ; \(c+1=\left(1-a\right)+\left(1-b\right)\)
Áp dụng bất đẳng thức Cosi, ta có : \(a+1=\left(1-b\right)+\left(1-c\right)\ge2\sqrt{\left(1-b\right)\left(1-c\right)}\left(1\right)\)
Tương tự : \(b+1\ge2\sqrt{\left(1-a\right)\left(1-c\right)}\left(2\right)\) ; \(c+1\ge2\sqrt{\left(1-a\right)\left(1-b\right)}\left(3\right)\)
Nhân (1), (2) , (3) theo vế : \(\left(1+a\right)\left(1+b\right)\left(1+c\right)\ge8\sqrt{\left(1-a\right)^2\left(1-b\right)^2\left(1-c\right)^2}=8\left(1-a\right)\left(1-b\right)\left(1-c\right)\)
\(\Rightarrow\left(1+a\right)\left(1+b\right)\left(1+c\right)\ge8\left(1-a\right)\left(1-b\right)\left(1-c\right)\) (đpcm)