Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đáp án: B
2 x x 2 + 1 ≥ 1 ⇔ 2 x - x 2 - 1 x 2 + 1 ≥ 0 ⇔ 2 x - x 2 - 1 ≥ 0 ⇔ - ( x - 1 ) 2 ≥ 0 ⇔ x = 1 ⇒ A = { 1 } .
∆ ' = b 2 - 4 . Để phương trình vô nghiệm thì
∆ ' < 0 ⇔ b 2 - 4 < 0 ⇔ b 2 < 4 ⇔ - 2 < b < 2 ⇒ B = { - 1 ; 0 ; 1 } . ⇒ A ⊂ B .

a: \(A\cap B=\left(-3;1\right)\)
\(A\cup B\)=[-5;4]
A\B=[1;4]
\(C_RA\)=R\A=(-∞;-3]\(\cap\)(4;+∞)
b: C={1;-1;5;-5}
\(B\cap C=\left\{-5;-1\right\}\)
Các tập con là ∅; {-5}; {-1}; {-5;-1}

\(\dfrac{2x}{x^2+1}\ge1\Leftrightarrow2x\ge x^2+1\Leftrightarrow x^2-2x+1\le0\\ \Leftrightarrow\left(x-1\right)^2\le0\)
Mà \(\left(x-1\right)^2\ge0\Leftrightarrow x-1=0\Leftrightarrow x=1\)
\(A=\left\{1\right\}\)
Để \(x^2-2bx+4=0\Leftrightarrow\Delta=4b^2-4\cdot4< 0\)
\(\Leftrightarrow b^2-4< 0\Leftrightarrow\left(b-2\right)\left(b+2\right)< 0\\ \Leftrightarrow x\le-2;x\ge2\)
\(\Leftrightarrow B=\left\{x\in R|x\le-2;x\ge2\right\}\)
Vậy \(A\cap B=\varnothing\)

Đặt \(\sqrt{x+m}=t\Rightarrow m=t^2-x\)
Pt trở thành:
\(x^2-2x-t=t^2-x\)
\(\Leftrightarrow x^2-t^2-x-t=0\)
\(\Leftrightarrow\left(x+t\right)\left(x-t-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}-x=t\\x-1=t\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}-x=\sqrt{x+m}\left(x\le0\right)\\x-1=\sqrt{x+m}\left(x\ge1\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-x=m\left(x\le0\right)\left(1\right)\\x^2-3x+1=m\left(x\ge1\right)\left(2\right)\end{matrix}\right.\)
TH1: (1) có nghiệm duy nhất và (2) vô nghiệm (sử dụng đồ thị hoặc BBT)
\(\Rightarrow\left\{{}\begin{matrix}m\ge0\\\left[{}\begin{matrix}m< -\dfrac{5}{4}\\\end{matrix}\right.\end{matrix}\right.\) (ko tồn tại m thỏa mãn)
TH2: (1) vô nghiệm và (2) có nghiệm duy nhất
\(\Rightarrow\left\{{}\begin{matrix}m< 0\\\left[{}\begin{matrix}m=-\dfrac{5}{4}\\m>-1\end{matrix}\right.\end{matrix}\right.\)\(\Rightarrow\left\{-\dfrac{5}{4}\right\}\cup\left(-1;0\right)\)
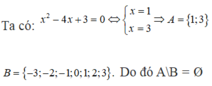
\(A=\left\{1;3\right\}\)
\(B=\left\{x\in R|-4< x< 4\right\}\)
Đề bài thiếu, 4 đáp án đều vô nghĩa