Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\overrightarrow {RJ} + \overrightarrow {IQ} + \overrightarrow {PS} = \left( {\overrightarrow {RA} + \overrightarrow {AJ} } \right) + \left( {\overrightarrow {IB} + \overrightarrow {BQ} } \right) + \left( {\overrightarrow {PC} + \overrightarrow {CS} } \right)\)
\( = \left( {\overrightarrow {RA} + \overrightarrow {CS} } \right) + \left( {\overrightarrow {AJ} + \overrightarrow {IB} } \right) + \left( {\overrightarrow {BQ} + \overrightarrow {PC} } \right) = \overrightarrow 0 + \overrightarrow 0 + \overrightarrow 0 = \overrightarrow 0 \)\(\)(đpcm)
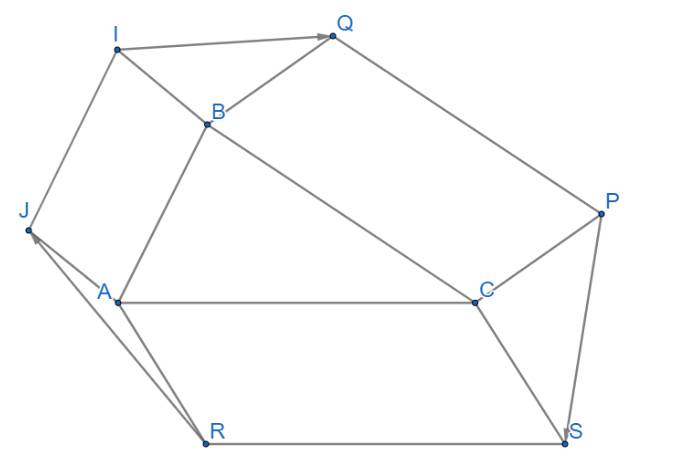

Có \(\overrightarrow{RJ}+\overrightarrow{IQ}+\overrightarrow{PS}=\overrightarrow{RA}+\overrightarrow{AJ}+\overrightarrow{IB}+\overrightarrow{BQ}+\overrightarrow{PC}+\overrightarrow{CS}\)
\(=\left(\overrightarrow{RA}+\overrightarrow{CS}\right)+\left(\overrightarrow{AJ}+\overrightarrow{IB}\right)+\left(\overrightarrow{BQ}+\overrightarrow{PC}\right)\)
\(=\overrightarrow{0}+\overrightarrow{0}+\overrightarrow{0}=\overrightarrow{0}\). ( Do tứ giác ABIJ, BCPQ, CARS là hình bình hành).
Vậy \(\overrightarrow{RJ}+\overrightarrow{IQ}+\overrightarrow{PS}=\overrightarrow{0}\).

AM vuông góc với DE chứ.
\(\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{AC}\right);\overrightarrow{DE}=\left(\overrightarrow{AE}-\overrightarrow{AD}\right)\)
\(\Rightarrow\overrightarrow{AM}.\overrightarrow{DE}=\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{AC}\right)\left(\overrightarrow{AE}-\overrightarrow{AD}\right)\)
\(=\dfrac{1}{2}\left(\overrightarrow{AB}.\overrightarrow{AE}-\overrightarrow{AC}.\overrightarrow{AD}-\overrightarrow{AB}.\overrightarrow{AD}+\overrightarrow{AC}.\overrightarrow{AE}\right)\)
\(=\dfrac{1}{2}\left[AB.AE.cos\left(\widehat{BAC}+90^o\right)-AC.AD.cos\left(\widehat{BAC}+90^o\right)-AB.AD.cos90^o+AC.AE.cos90^o\right]\)
\(=0\)
\(\Rightarrow AM\perp DE\)

Bài 1 : Ta có :
\(VT=\overrightarrow{RJ}+\overrightarrow{IQ}+\overrightarrow{PS}=\overrightarrow{RA}+\overrightarrow{AJ}+\overrightarrow{IB}+\overrightarrow{BQ}+\overrightarrow{PC}+\overrightarrow{CS}\)
Mà : \(\left\{{}\begin{matrix}\overrightarrow{RA}=\overrightarrow{SC}\\\overrightarrow{AJ}=\overrightarrow{BI}\\\overrightarrow{BQ}=\overrightarrow{CP}\end{matrix}\right.\) ( Tính chất hình bình hành )
\(\Rightarrow VT=\overrightarrow{SC}+\overrightarrow{CS}+\overrightarrow{BI}+\overrightarrow{IB}+\overrightarrow{CP}+\overrightarrow{PC}\)
\(=\overrightarrow{SC}-\overrightarrow{SC}+\overrightarrow{BI}-\overrightarrow{BI}+\overrightarrow{CP}-\overrightarrow{CP}=0\) ( đpcm )
Câu 2 : Gọi \(AM\) là đường trung tuyến của \(\Delta ABC\) và lấy D đối xứng với G qua M .
Theo quy tắc hình bình hành ta có :
\(\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{GD}\left(1\right)\)
Mà : \(\overrightarrow{GA}=\overrightarrow{DG}\Rightarrow\overrightarrow{GA}+\overrightarrow{GD}=0\left(2\right)\)
Từ \(\left(1\right);\left(2\right)\Rightarrow\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{GA}+\overrightarrow{GD}=0\) (đpcm)
2)
Gọi I là giao điểm của AG và BC
=> I là trung điểm BC
Tên tia đối tia IG lấy H sao cho I là trung điểm GH
=> Tứ giác BGCH là hình bình hành
\(\text{Ta có : }AG=\frac{2}{3}AI=2\cdot\frac{1}{3}AI=2GI\left(\text{Tính chát trọng tâm }\Delta\right)\\ GH=2GI\left(I\text{ là trung điểm }GH\right)\\ \Rightarrow AG=GH\\ \Rightarrow\overrightarrow{GA}+\overrightarrow{GH}=0\\ \text{Mà theo quy tắc hình bình hành }:\overrightarrow{GB}+\overrightarrow{GC}=GH\\ \Rightarrow\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{GA}+\overrightarrow{GH}=0\)
Ta có:
AJIB là hình bình hành nên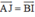
Tương tự như vậy:
BCPQ là hình bình hành nên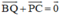
CARS là hình bình hành nên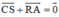
Do đó: