\(\overrightarrow{NP}=\overrightarrow{NC}+\overrightarrow{CP}\)

\(=\dfrac{2}{3}\overrightarrow{BC}+\dfrac{1}{3}\overrightarrow{CA}\)

\(=-\dfrac{2}{3}\overrightarrow{CB}+\dfrac{1}{3}\overrightarrow{CA}\)

\(\overrightarrow{PM}=\overrightarrow{PA}+\overrightarrow{AM}\)

\(=\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{AB}\)

\(=\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{3}\left(\overrightarrow{AC}+\overrightarrow{CB}\right)\)

\(=\dfrac{1}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{CB}\)

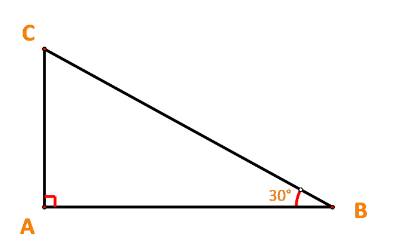
Ta có: \(BC = \frac{{AB}}{{\cos {{30}^o}}} = 3:\frac{{\sqrt 3 }}{2} = 2\sqrt 3 \); \(AC = BC.\sin \widehat {ABC} = 2\sqrt 3 .\sin {30^o} = \sqrt 3 .\)

\(\overrightarrow {BA} .\overrightarrow {BC}  = \left| {\overrightarrow {BA} } \right|.\left| {\overrightarrow {BC} } \right|\cos (\overrightarrow {BA} ,\overrightarrow {BC} ) = 3.2\sqrt 3 .\cos \widehat {ABC} = 6\sqrt 3 .\cos {30^o} = 6\sqrt 3 .\frac{{\sqrt 3 }}{2} = 9.\)

\(\overrightarrow {CA} .\overrightarrow {CB}  = \left| {\overrightarrow {CA} } \right|.\left| {\overrightarrow {CB} } \right|\cos (\overrightarrow {CA} ,\overrightarrow {CB} ) = \sqrt 3 .2\sqrt 3 .\cos \widehat {ACB} = 6.\cos {60^o} = 6.\frac{1}{2} = 3.\)

Ta có: AB+ BC =AC nên ba điểm A, B,C thẳng hàng và B nằm giữa A, C

Khi đó C A → . C B → = C A . C B . cos C A → , C B → = 3.5. cos 0 0 = 15.  

Chọn B.

Cách khác. Ta có  A B 2 = A B → 2 = C B → − C A → 2 = C B 2 − 2 C B → .    C A → + C A 2

⇒ C B → C A → = 1 2 C B 2 + C A 2 − A B 2 = 1 2 3 2 + 5 2 − 2 2 = 15.

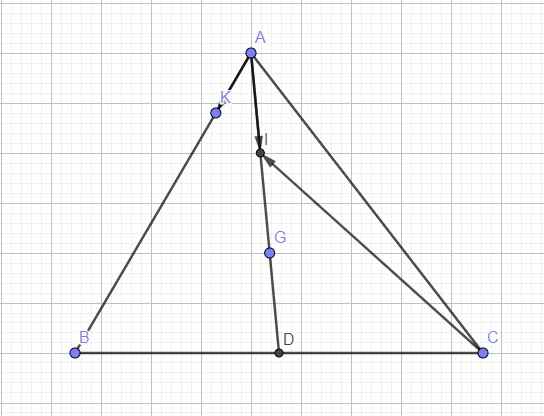
Do G là trọng tâm tam giác 

\(\Rightarrow\overrightarrow{AG}=\dfrac{2}{3}\overrightarrow{AD}=\dfrac{2}{3}\left(\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}\right)=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{1}{3}\overrightarrow{AC}=\dfrac{1}{3}\overrightarrow{AC}+\dfrac{1}{3}\overrightarrow{CB}+\dfrac{1}{3}\overrightarrow{AC}\)

\(=\dfrac{2}{3}\overrightarrow{AC}+\dfrac{1}{3}\overrightarrow{CB}=-\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{CB}\)

Do I là trung điểm AG

\(\Rightarrow\overrightarrow{AI}=\dfrac{1}{2}\overrightarrow{AG}=\dfrac{1}{2}\left(-\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{CB}\right)=-\dfrac{1}{3}\overrightarrow{CA}+\dfrac{1}{6}\overrightarrow{CB}\)

\(\overrightarrow{AK}=\dfrac{1}{5}\overrightarrow{AB}=\dfrac{1}{5}\left(\overrightarrow{AC}+\overrightarrow{CB}\right)=-\dfrac{1}{5}\overrightarrow{CA}+\dfrac{1}{5}\overrightarrow{CB}\)

\(\overrightarrow{CI}=\overrightarrow{CA}+\overrightarrow{AI}=\overrightarrow{CA}-\dfrac{1}{3}\overrightarrow{CA}+\dfrac{1}{6}\overrightarrow{CB}=\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{6}\overrightarrow{CB}\)

\(\overrightarrow{CK}=\overrightarrow{CA}+\overrightarrow{AK}=\overrightarrow{CA}-\dfrac{1}{5}\overrightarrow{CA}+\dfrac{1}{5}\overrightarrow{CB}=\dfrac{4}{5}\overrightarrow{CA}+\dfrac{1}{5}\overrightarrow{CB}\)

Chọn C.

Ta có: AB + BC = AC nên  ba điểm A; B; C  thẳng hàng và B nằm giữa A; C

Khi đó