
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Tính giá trị của biểu thức :
a) \(2\sin30^0+3\cos45^0-\sin60^0\)
b) \(2\cos30^0+3\sin45^0-\cos60^0\)

a)
\(2sin30+3sin45^o-sin60^o=2.\dfrac{1}{2}+3.\dfrac{\sqrt{2}}{2}-\dfrac{\sqrt{3}}{2}\)\(=\dfrac{2+3\sqrt{2}-\sqrt{3}}{2}\).
b)\(2cos30^o+3sin45^o-cos60^o=2.\dfrac{\sqrt{3}}{2}+3.\dfrac{\sqrt{2}}{2}-\dfrac{1}{2}\)\(=\dfrac{2\sqrt{3}+3\sqrt{2}-1}{2}\).

Chú ý rằng: sin450 = cos450, sin400 = cos500, sin500 = cos400
Ta được:
\(\dfrac{\cos50^0-\cos45^0+\cos50^0}{\cos40^0-\cos45^0+\cos50^0}-\dfrac{6\times3\left(\dfrac{\sqrt{3}}{3}+\tan15^0\right)}{3\left(1-\dfrac{\sqrt{3}}{3}\tan15^0\right)}\)
\(=1-6\left(\dfrac{\tan30^0+\tan15^0}{1-\tan30^0\times\tan15^0}\right)\)
\(=1-6\tan45^0=-5\)

M thuộc d nên: \(a-2b-2=0\Rightarrow2b=a-2\)
\(\left\{{}\begin{matrix}\overrightarrow{MA}=\left(-a;1-b\right)\\\overrightarrow{MB}=\left(3-a;4-b\right)\end{matrix}\right.\) \(\Rightarrow\overrightarrow{MA}+\overrightarrow{MB}=\left(3-2a;5-2b\right)=\left(3-2a;9-2a\right)\)
Đặt \(T=\left|\overrightarrow{MA}+\overrightarrow{MB}\right|=\sqrt{\left(3-2a\right)^2+\left(9-2a\right)^2}=\sqrt{8a^2-48a+90}=\sqrt{8\left(a-3\right)^2+18}\ge\sqrt{18}\)
Dấu "=" xảy ra khi \(a-3=0\Leftrightarrow a=3\Rightarrow b=\dfrac{1}{2}\)

a)
\(A=cos^230^o-sin^230^o=\left(\dfrac{\sqrt{3}}{2}\right)^2-\left(\dfrac{1}{2}\right)^2=\dfrac{1}{2}\);
\(B=cos60^o+sin45^o=\dfrac{1}{2}+\dfrac{\sqrt{2}}{2}\).
Vì vậy \(A< B\).
b)
\(C=\dfrac{2tan30^o}{1-tan^230^o}=\dfrac{2\dfrac{\sqrt{3}}{2}}{1-\left(\dfrac{\sqrt{3}}{2}\right)^2}=\sqrt{3}\).
\(D=\left(-tan135^o\right)tan60^o=-\left(-1\right).\sqrt{3}=\sqrt{3}\).
Vậy \(C=D\).

(d): VTPT là (m;1)
(d'): VTPT là (m;-4)
(d) vuông góc (d')
=>m^2-4=0
=>m=2 hoặc m=-2
=>Có 2 số nguyên m thỏa mãn
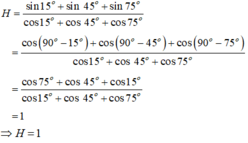
Ta có cos45 độ + sin45 độ=√2/2+√2/2=√2
Chọn (B).