
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


d) Điều kiện x>0. Áp dụng công thức đổi cơ số, ta có :
\(\log_2x+\log_3x+\log_4x=\log_{20}x\)
\(\Leftrightarrow\log_2x+\frac{\log_2x}{\log_23}+\frac{\log_2x}{\log_24}=\frac{\log_2x}{\log_220}\)
\(\Leftrightarrow\log_2x\left(1+\frac{1}{\log_23}+\frac{1}{2}+\frac{1}{\log_220}\right)=0\)
\(\Leftrightarrow\log_2x\left(\frac{3}{2}+\log_22-\log_{20}2\right)=0\)
Ta có \(\frac{3}{2}+\log_22-\log_{20}2>\frac{3}{2}+0-1>0\)
Do đó, từ phương trình trên, ta phải có \(\log_2x=0\) hay \(x=2^0=1\)
Vậy nghiệm duy nhất của phương trình là \(x=1\)
c) Điều kiện x>0, đưa về cùng cơ số 5, ta có :
\(\log_5x^3+3\log_{25}x+\log_{\sqrt{25}}\sqrt{x^3}=\frac{11}{2}\)
\(\Leftrightarrow3\log_5x+3\log_{5^2}x+\log_{5^{\frac{3}{2}}}x^{\frac{3}{2}}=\frac{11}{2}\)
\(\Leftrightarrow3\log_5x+3\frac{1}{2}\log_5x+\frac{3}{2}.\frac{2}{3}\log_5x=\frac{11}{2}\)
\(\Leftrightarrow\frac{11}{2}\log_5x=\frac{11}{2}\)
\(\Leftrightarrow\log_5x=1\)
\(\Leftrightarrow x=5^1=5\) thỏa mãn
Vậy phương trình chỉ có 1 nghiệ duy nhất \(x=5\)

1.
xx=2
=> x=21/x = e(ln2)/x
=> ln2=((ln2)/x). e(ln2)/x
Gọi A là hàm ngược của f(x),
=> A(ln2)=A((ln2)/x). e(ln2)/x)=ln2/x (tính chất hàm ngược)
=> x= ln2/A(ln2) = 1.55961
2.
x2=2x
<=> x2.1/2.1/x=2x.1/2.1/x
<=> x1/x=21/2
hàm đặc trưng
...
x=2

\(\Leftrightarrow3^{x^2}.4^{x+1}=3^{-x}\)
Lấy logarit cơ số 3 hai vế:
\(\Rightarrow log_3\left(3^{x^2}.4^{x+1}\right)=log_3\left(3^{-x}\right)\)
\(\Leftrightarrow x^2+\left(x+1\right)log_34=-x\)
\(\Leftrightarrow x^2+x+\left(x+1\right)log_34=0\)
\(\Leftrightarrow x\left(x+1\right)+\left(x+1\right)log_34=0\)
\(\Leftrightarrow\left(x+1\right)\left(x+log_34\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-log_34=-2log_32\end{matrix}\right.\)


\(log_2\left(5^{x+1}-25^x\right)=2\) \(\Leftrightarrow5^{x+1}-25^x=2^2\)\(\Leftrightarrow-\left(5^x\right)^2+5.5^x-4=0\).
Đặt \(5^x=t\left(t>0\right)\), phuyowng trình trở thành:
\(-t^2+5t-4=0\) \(\Leftrightarrow\left[{}\begin{matrix}t=1\\t=4\end{matrix}\right.\).
\(t=1\Leftrightarrow5^x=1\) \(\Leftrightarrow x=0\).
\(t=4\Leftrightarrow5^x=4\Leftrightarrow x=log_54\).
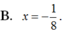
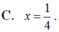
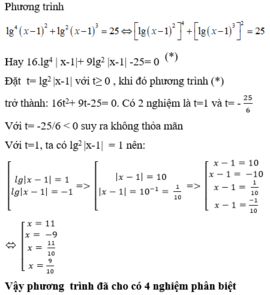


Đáp án C