Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


`2x^3 +6x^2 =x^2 +3x`
`<=> 2x^3 +6x^2 -x^2 -3x=0`
`<=> 2x^3 +5x^2 -3x=0`
`<=> x(2x^2 +5x-3)=0`
`<=> x(2x^2 +6x-x-3)=0`
`<=> x[2x(x+3)-(x+3)]=0`
`<=> x(2x-1)(x+3)=0`
\(< =>\left[{}\begin{matrix}x=0\\2x-1=0\\x+3=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x=\dfrac{1}{2}\\x=-3\end{matrix}\right.\)
b)
`(2+x)^2 -(2x-5)^2=0`
`<=> (2+x-2x+5)(2+x+2x-5)=0`
`<=> (-x+7)(3x-3)=0`
\(< =>\left[{}\begin{matrix}-x+7=0\\3x-3=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=7\\x=1\end{matrix}\right.\)
`a) 2x^3 + 6x^2 = x^2 + 3x`
`=> 2x^3 + 6x^2 - x^2 - 3x = 0`
`=> 2x^3 + 5x^2 - 3x = 0`
`=> x(2x^2 + 5x - 3) = 0`
`=> x (2x^2 + 6x - x - 3) = 0`
`=> x [(2x^2 + 6x) - (x+3)] = 0`
`=> x [2x(x+3) - (x+3)] = 0`
`=> x (2x - 1)(x+3) = 0`
`=> x = 0` hoặc `2x - 1 = 0` hoặc `x + 3 = 0`
`=> x = 0` hoặc `x = 1/2` hoặc `x = -3`
`b) (2+x)^2 - (2x-5)^2 = 0`
`=> (2+x+2x-5)(2+x-2x+5) = 0`
`=> (3x - 3)(7-x) = 0`
`=> 3x - 3 = 0` hoặc `7 - x = 0`
`=> x = 1` hoặc `x = 7`

a, \(x^4-x^2-2=0\Leftrightarrow x^4-2x^2+x^2-2=0\)
\(\Leftrightarrow x^2\left(x^2-2\right)+\left(x^2-2\right)=0\Leftrightarrow\left(x^2+1>0\right)\left(x^2-2\right)=0\Leftrightarrow x=\pm\sqrt{2}\)
b, \(\Leftrightarrow x^2\left(x^2+2x+1\right)=0\Leftrightarrow x^2\left(x+1\right)^2=0\Leftrightarrow x=0;x=-1\)
c, \(\Leftrightarrow\left(x-1\right)\left(x^2+x+1>0\right)=0\Leftrightarrow x=1\)
d, \(\Leftrightarrow6x^2-3x-4x+2=0\Leftrightarrow\left(3x-2\right)\left(2x-1\right)=0\Leftrightarrow x=\dfrac{2}{3};x=\dfrac{1}{2}\)
a)
/ \(x^4+x^2-2=0\)
\(\Leftrightarrow\left(x^2\right)^2-x^2+2x^2-2=0\\ \Leftrightarrow x^2\left(x^2-1\right)+2\left(x^2-1\right)=0\\ \Leftrightarrow\left(x^2+2\right)\left(x^2-1\right)=0\\ \Leftrightarrow\left(x^2+2\right)\left(x-1\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x^2+2=0\\x+1=0\\x-1-0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)

Câu 6:Thực hiện phép nhân -2x(x2 + 3x - 4) ta được:
A.-2x3 - 6x2 – 8x B. 2x3 -6x2 – 8x C. -2x3 - 6x2 + 8x D. -2x3 + 3x2 -4
Câu 7 : Phân tích đa thức x2 + 2xy + y2 – 9z2 thành nhân tử ta được:
A. (x+y+3z)(x+y–3z)
B. (x-y+3z)(x+y–3z)
C.(x - y +3z)(x - y – 3z)
D. (x + y +3z)(x -y – 3z)
Câu 9: Phân tích đa thức x2 + 7x + 12 thành nhân tử ta được:
A. (x - 3)( x + 4 ) B. (x + 3)( x + 4 ) C.(x + 5)( x + 2 ) D. (x -5)( x + 2 )
Câu 10: Giá trị của biểu thức (x2 + 4x + 4) tại x = - 2 là:
A. 4 B. -2 C. 0 D. -8
Mấy câu còn lại bị lỗi r nhé

1) Ta có: \(x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
hay x=2
Vậy: S={2}

\(a,6x^2-5x+3=2x-3x\left(3-2x\right)\)
\(\Leftrightarrow6x^2-5x+3=2x-9x+6x^2\)
\(\Leftrightarrow6x^2-6x^2-5x-2x+9x=-3\)
\(\Leftrightarrow2x=-3\)
\(\Leftrightarrow x=-\dfrac{3}{2}\)
\(b,\left(3x-1\right)\left(4x+3\right)=2\left(3x-1\right)\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3\right)-2\left(3x-1\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\\4x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=-\dfrac{1}{4}\end{matrix}\right.\)
\(6x^2-5x+3=2x-3x\left(3-2x\right)\)
\(\Leftrightarrow6x^2-5x+3=2x-9x+6x^2\)
\(\Leftrightarrow6x^2-5x+3-2x+9x-6x^2=0\)
\(\Leftrightarrow2x+3=0\)
\(\Leftrightarrow2x=-3\)
\(\Leftrightarrow x=\dfrac{-3}{2}\)
\(\text{Vậy phương trình có tập nghiệm là }S=\left\{\dfrac{-3}{2}\right\}\)
\(\left(3x-1\right)\left(4x+3\right)=2\left(3x-1\right)\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3\right)-2\left(3x-1\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3-2\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\\4x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=1\\4x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=\dfrac{1}{4}\end{matrix}\right.\)
\(\text{Vậy phương trình có tập nghiệm là }S=\left\{\dfrac{1}{3};\dfrac{1}{4}\right\}\)


2x3 + 5x2 – 3x = 0
⇔ x(2x2 + 5x – 3) = 0
⇔ x.(2x2 + 6x – x – 3) = 0
⇔ x. [2x(x + 3) – (x + 3)] = 0
⇔ x.(2x – 1)(x + 3) = 0
⇔ x = 0 hoặc 2x – 1 = 0 hoặc x + 3 = 0
+ 2x – 1 = 0 ⇔ 2x = 1 ⇔ x = 1/2.
+ x + 3 = 0 ⇔ x = -3.
Vậy phương trình có tập nghiệm 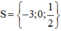
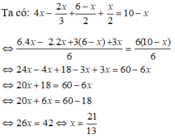
2x3 + 6x2 = x2 + 3x
⇔ (2x3 + 6x2) – (x2 + 3x) = 0
⇔ 2x2(x + 3) – x(x + 3) = 0
⇔ x(x + 3)(2x – 1) = 0
(Nhân tử chung là x(x + 3))
⇔ x = 0 hoặc x + 3 = 0 hoặc 2x – 1 = 0
+ x + 3 = 0 ⇔ x = -3.
+ 2x – 1 = 0 ⇔ 2x = 1 ⇔ x = 1/2.
Vậy tập nghiệm của phương trình là