
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) Ta có: \(x^3-9x^2+19x-11=0\)
\(\Leftrightarrow x^3-x^2-8x^2+8x+11x-11=0\)
\(\Leftrightarrow x^2\left(x-1\right)-8x\left(x-1\right)+11\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2-8x+11\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x^2-8x+11=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\sqrt{5}+4\\x=-\sqrt{5}+4\end{matrix}\right.\)
Vậy: \(S=\left\{1;\sqrt{5}+4;-\sqrt{5}+4\right\}\)

\(a,9\left(2x+1\right)=4\left(x-5\right)^2\)
\(4x^2-40x+100=18x+9\)
\(4x^2-58x+91=0\)
\(\Rightarrow\orbr{\begin{cases}x=\frac{29+3\sqrt{53}}{4}\\x=\frac{29-3\sqrt{53}}{4}\end{cases}}\)
\(b,x^3-4x^2-12x+27=0\)
\(\left(x+3\right)\left(x^2-7x+9\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x+3=0\\x^2-7x+9=0\end{cases}\Rightarrow\orbr{\begin{cases}x=-3\\x=\frac{7\pm\sqrt{13}}{2}\end{cases}}}\)
\(c,x^3+3x^2-6x-8=0\)
\(\left(x+4\right)\left(x-2\right)\left(x+1\right)=0\)
\(Th1:x+4=0\Leftrightarrow x=-4\)
\(Th2:x-2=0\Leftrightarrow x=2\)
\(Th3:x+1=0\Leftrightarrow x=-1\)
\(a,9.\left(2x+1\right)=4.\left(x-5\right)^2\)
\(< =>4x^2-40x+100=18x+9\)
\(< =>4x^2+58x+91=0\)
\(< =>\orbr{\begin{cases}x=\frac{29-3\sqrt{53}}{4}\\x=\frac{29+3\sqrt{53}}{4}\end{cases}}\)
\(b,x^3-4x^2-12x+27=0\)
\(< =>\left(x+3\right)\left(x^2-7x+9\right)=0\)
\(< =>\orbr{\begin{cases}x+3=0\\x^2-7x+9=0\end{cases}}\)
\(< =>\orbr{\begin{cases}x=-3\\x=\frac{7\pm\sqrt{13}}{2}\end{cases}}\)


a) \(x^3-9x^2+27x-27=-8\Leftrightarrow\left(x-3\right)^3=\left(-2\right)^3\)
\(\Rightarrow x-3=-2\Leftrightarrow x=1\) vậy \(x=1\)
b) \(64x^3+48x^2+12x+1=0\Leftrightarrow\left(4x+1\right)^3=3^3\)
\(\Rightarrow4x+1=3\Leftrightarrow4x=2\Leftrightarrow x=\dfrac{2}{4}=\dfrac{1}{2}\) vậy \(x=\dfrac{1}{2}\)
c) \(x^3-3x^2+3x-1=0\Leftrightarrow\left(x-1\right)^3=0\)
\(\Leftrightarrow x-1=0\Leftrightarrow x=1\) vậy \(x=1\)
d) \(x^3+6x^2+12x+8=0\Leftrightarrow\left(x+2\right)^3=0\)
\(\Leftrightarrow x+2=0\Leftrightarrow x=-2\) vậy \(x=-2\)
e) \(x^3-6x^2+12x-8=0\Leftrightarrow\left(x-2\right)^3=0\)
\(\Leftrightarrow x-2=0\Leftrightarrow x=2\) vậy \(x=2\)

Bài 1:
\(D=\dfrac{5x^2-30x+53}{x^2-6x+10}=\dfrac{5\left(x^2-6x+10\right)+3}{x^2-6x+10}=5+\dfrac{3}{x^2-6x+10}\)
\(=5+\dfrac{3}{\left(x-3\right)^2+1}\)
Ta có: \(\left(x+3\right)^2+1\ge1\Rightarrow\dfrac{3}{\left(x-3\right)^2+1}\le3\)
\(\Rightarrow D\le3+5=8\)
Vậy max D= 8 <=> x=3
Bài 2:
\(8\left(x-3\right)^3+x^3=6x^2-12x+8\)
\(\Leftrightarrow\left[2\left(x-3\right)^3\right]=-x^3+3.2x^2-3.2^2x+2^3\)
\(\Leftrightarrow\left(2x-6\right)^3=\left(2-x\right)^3\)
\(\Leftrightarrow2x-6=2-x\)
\(\Leftrightarrow3x=8\Leftrightarrow x=\dfrac{8}{3}\)
Vậy tập nghiệm : \(S=\left\{\dfrac{8}{3}\right\}\)
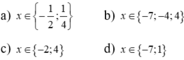
\(x^6-6x^4-64x^3+12x^2-8=0\)
\(\Leftrightarrow\left(x^2-4x-2\right)\left(x^4+4x^3+12x^2-8x+4\right)=0\)
\(\Leftrightarrow\left(x^2-4x-2\right)\left[\left(x^4+4x^3+4x^2\right)+\left(8x^2-8x+\frac{8}{4}\right)+2\right]=0\)
\(\Leftrightarrow\left(x^2-4x-2\right)\left[\left(x^2+2x\right)^2+8\left(x-\frac{1}{2}\right)^2+2\right]=0\)
\(\Leftrightarrow x^2-4x-2=0\)
\(\Leftrightarrow x=2\pm\sqrt{6}\)