AB<AC

=>góc B>góc C

=>90 độ-góc B<90 độ-góc C

=>góc HAB<góc HAC

\(AB< AC\\ \Rightarrow\widehat{C}< \widehat{B}\)

Xét tam giác \(AHB\) và \(AHC\) có

\(\left\{{}\begin{matrix}\widehat{HAB}=90^o-\widehat{B}\\\widehat{HAC}=90^o-\widehat{C}\end{matrix}\right.\)

mà \(\widehat{B}>\widehat{C}\)

\(\Rightarrow\widehat{HAB}< \widehat{HAC}\)

Đặt \(\dfrac{x}{4}=\dfrac{y}{7}=k\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=4k\\y=7k\end{matrix}\right.\)

Ta có: xy=112

\(\Leftrightarrow28k^2=112\)

\(\Leftrightarrow k^2=4\)

Trường hợp 1: k=2

\(\Leftrightarrow\left\{{}\begin{matrix}x=4k=4\cdot2=8\\y=7k=7\cdot2=14\end{matrix}\right.\)

Trường hợp 2: x=-2

\(\Leftrightarrow\left\{{}\begin{matrix}x=4k=-8\\y=7k=-14\end{matrix}\right.\)

10: Chọn B

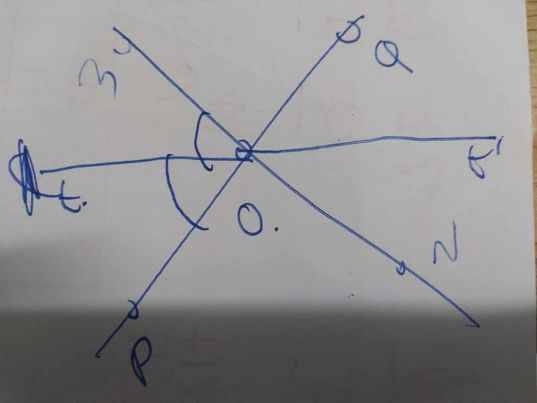
Ot là phân giác của \(\widehat{MOP}\)

=>\(\widehat{MOP}=2\cdot\widehat{tOP}\)

\(\widehat{MOP}=\widehat{NOQ}\)

=>\(\widehat{NOQ}=2\cdot\widehat{tOP}\)

mà \(\widehat{tOP}=\widehat{t'OQ}\)(hai góc đối đỉnh)

nên \(\widehat{NOQ}=2\cdot\widehat{t'OQ}\)

=>Ot' là phân giác của góc NOQ

11:

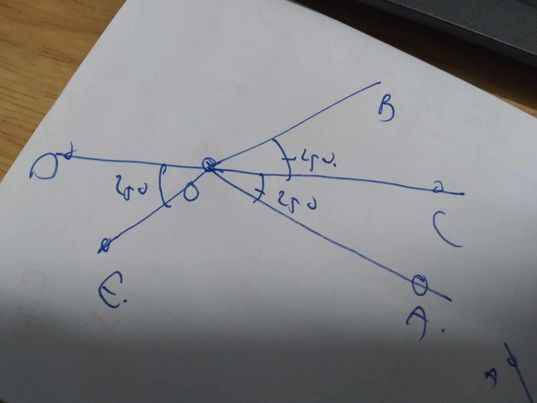
OC là phân giác của góc AOB

=>\(\widehat{AOC}=\widehat{BOC}=\dfrac{50^0}{2}=25^0\)

\(\widehat{DOE}=\widehat{BOC}\left(=25^0\right)\)

=>\(\widehat{DOE}+\widehat{DOB}=180^0\)

=>OB và OE là hai tia đối nhau

=>Hai góc đối đỉnh là \(\widehat{BOC};\widehat{DOE}\)

=>Chọn D

12:

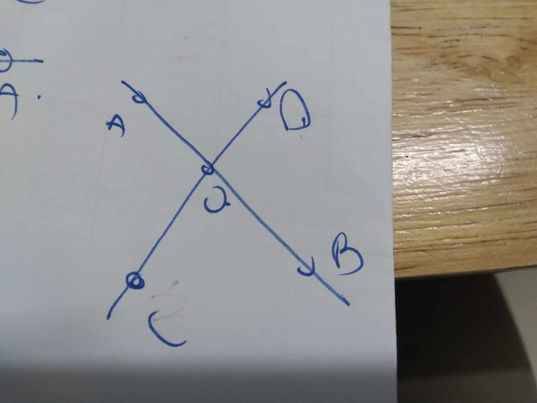
\(\widehat{AOC}+\widehat{AOD}=180^0\)

\(\widehat{AOC}-\widehat{AOD}=50^0\)

Do đó: \(\widehat{AOC}=\dfrac{180^0+50^0}{2}=115^0;\widehat{AOD}=115^0-50^0=65^0\)

=>\(\widehat{BOC}=\widehat{AOD}=65^0\)

=>Chọn B

a: \(\widehat{ACB}=30^0\)

a: Xét ΔABD và ΔHBD có 

BA=BH

\(\widehat{ABD}=\widehat{HBD}\)

BD chung

Do đó: ΔABD=ΔHBD

b: Ta có: ΔABD=ΔHBD

nên \(\widehat{BAD}=\widehat{BHD}\)

mà \(\widehat{BAD}=90^0\)

nên \(\widehat{BHD}=90^0\)

hay DH\(\perp\)BC

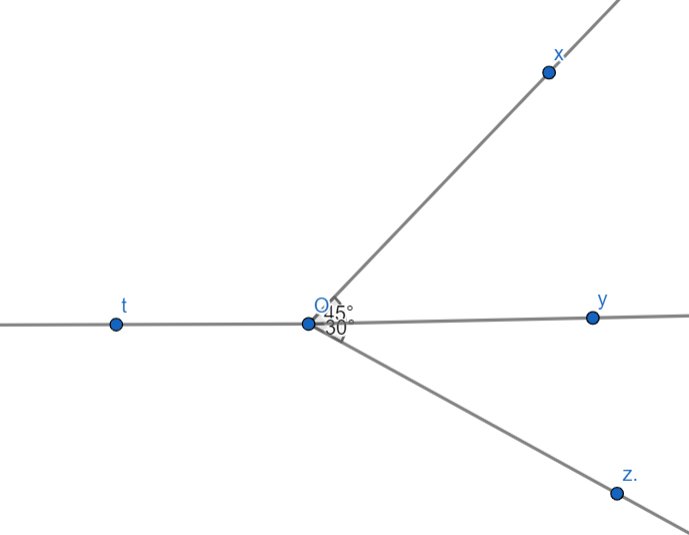
a) Các góc kề bù nhau là: 

1. \(\widehat{xOy}\) và \(\widehat{xOt}\)

2. \(\widehat{yOz}\) và \(\widehat{zOt}\)    

b) Ta có: \(\widehat{yOt}\) là góc bẹt \(\Rightarrow\widehat{yOt}=180^o\)

Mà \(\widehat{xOy}\) và \(\widehat{xOt}\) kề bù \(\Rightarrow\widehat{xOy}+\widehat{xOt}=\widehat{yOt}\)

\(\Rightarrow\widehat{xOt}=\widehat{yOt}-\widehat{xOy}=180^o-45^o=135^o\) 

Ta có: \(\widehat{xOz}=\widehat{xOy}+\widehat{yOz}=45^o+30^o=75^o\)

Mà \(\widehat{yOz}\) và \(\widehat{zOt}\) kề bù \(\Rightarrow\widehat{yOz}+\widehat{zOt}=\widehat{yOt}=180^o\)

\(\Rightarrow\widehat{zOt}=\widehat{yOt}-\widehat{yOz}=180^o-30^o=150^o\)

Lời giải:

$OE\parallel MN\Rightarrow \widehat{MOE}=\widehat{OMN}=45^0$ (hai góc so le trong)

$OE\parallel QP\Rightarrow \widehat{EOP}+\widehat{OPQ}=180^0$ (hai góc trong cùng phía)

$\Rightarrow \widehat{EOP}=180^0-\widehat{OPQ}=180^0-135^0=45^0$

Ta có:

$\widehat{MOP}=\widehat{MOE}+\widehat{EOP}=45^0+45^0=90^0$

b.

Có, vì tia $OE$ nằm giữa $OM, OP$ và $\widehat{MOE}=\widehat{EOP}=45^0$

Bài 2: Chọn C

Bài 4: 

a: \(\widehat{C}=180^0-80^0-50^0=50^0\)

Xét ΔABC có \(\widehat{A}=\widehat{C}< \widehat{B}\)

nên BC=AB<AC

b: Xét ΔABC có AB<BC<AC

nên \(\widehat{C}< \widehat{A}< \widehat{B}\)