
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\sqrt{4x^2-4x+9}=3\\ \Rightarrow4x^2-4x+9=9\\ \Rightarrow4x\left(x-1\right)=0\\ \Rightarrow\left[{}\begin{matrix}4x=0\\x-1=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
Ta có: \(\sqrt{4x^2-4x+9}=3\)
\(\Leftrightarrow4x^2-4x=0\)
\(\Leftrightarrow4x\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)

b) Ta có: \(9x^4+8x^2-1=0\)
\(\Leftrightarrow9x^4+9x^2-x^2-1=0\)
\(\Leftrightarrow9x^2\left(x^2+1\right)-\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)\left(9x^2-1\right)=0\)
mà \(x^2+1>0\forall x\)
nên \(9x^2-1=0\)
\(\Leftrightarrow9x^2=1\)
\(\Leftrightarrow x^2=\dfrac{1}{9}\)
hay \(x\in\left\{\dfrac{1}{3};-\dfrac{1}{3}\right\}\)
Vậy: \(S=\left\{\dfrac{1}{3};-\dfrac{1}{3}\right\}\)

a: \(Q=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)-2\sqrt{x}\left(\sqrt{x}-2\right)-5\sqrt{x}-2}{x-4}:\dfrac{\sqrt{x}\left(3-\sqrt{x}\right)}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{x+3\sqrt{x}+2-2x+4\sqrt{x}-5\sqrt{x}-2}{x-4}\cdot\dfrac{\left(\sqrt{x}+2\right)^2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-x+2\sqrt{x}}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-\sqrt{x}\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\cdot\left(-1\right)}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}-3}=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\)
b: Khi x=4-2căn 3 thì \(Q=\dfrac{\sqrt{3}-1+2}{\sqrt{3}-1-3}=\dfrac{\sqrt{3}+1}{\sqrt{3}-4}=\dfrac{-7-5\sqrt{3}}{13}\)
c: Q>1/6
=>Q-1/6>0
=>\(\dfrac{\sqrt{x}+2}{\sqrt{x}-3}-\dfrac{1}{6}>0\)
=>\(\dfrac{6\sqrt{x}+12-\sqrt{x}+3}{6\left(\sqrt{x}-3\right)}>0\)
=>\(\dfrac{5\sqrt{x}+9}{6\left(\sqrt{x}-3\right)}>0\)
=>căn x-3>0
=>x>9

\(Q=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{\sqrt{x}-1+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ Q=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}}=\dfrac{\left(\sqrt{x}+1\right)^2}{x}\)

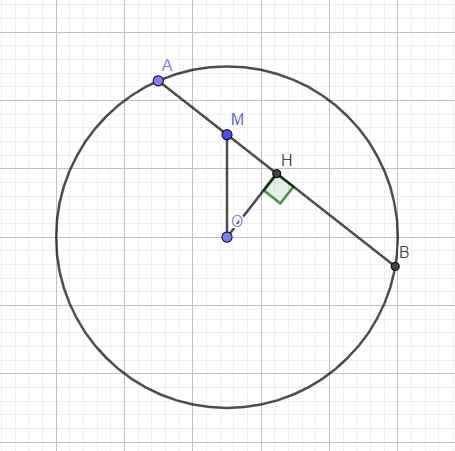
Gọi AB là dây cung qua M, H là trung điểm AB \(\Rightarrow OH\perp AB\) và \(OH\le OM=3\)
Áp dụng định lý Pitago:
\(OH^2+AH^2=OA^2=25\)
\(\Rightarrow AB=2AH=2\sqrt{25-OH^2}\)
AB nguyên khi \(25-OH^2=\dfrac{k^2}{4}\)
\(\Rightarrow OH^2=25-\dfrac{k^2}{4}\)
\(0\le OH\le3\Rightarrow0\le OH^2\le9\)
\(\Rightarrow0\le25-\dfrac{k^2}{4}\le9\)
\(\Rightarrow64\le k^2\le100\Rightarrow8\le k\le10\)
\(\Rightarrow k=\left\{8;9;10\right\}\) có 3 giá trị nguyên

Gọi chiều dài của mảnh đất là x (25<x<50)
Chiều rộng của mảnh đất là: \(50-x\) (m)
Diện tích mảnh đất ban đầu: \(x\left(50-x\right)\)
Chiều dài và chiều rộng mảnh đất sau khi mở lối đi lần lượt là: \(x-2\) và \(48-x\)
Diện tích phần đất trồng rau: \(\left(x-2\right)\left(48-x\right)\)
Ta có pt:
\(\left(x-2\right)\left(48-x\right)=\dfrac{84}{100}x\left(50-x\right)\)
\(\Rightarrow x^2-50x+600=0\Rightarrow\left[{}\begin{matrix}x=20\left(loại\right)\\x=30\end{matrix}\right.\)
Vậy chiều rộng mảnh đất là 20 (m)


Đề bài không chính xác, pt này không giải được
Pt hợp lý cần có dạng:
\(\dfrac{2x}{3x^2-5x+2}+\dfrac{13x}{3x^2+x+2}=...\)

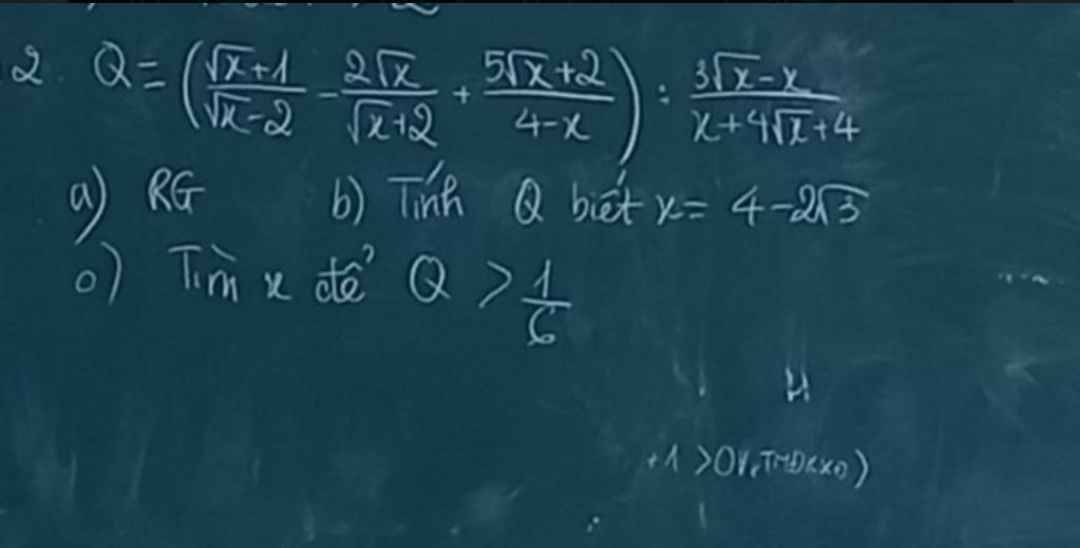




Chọn B