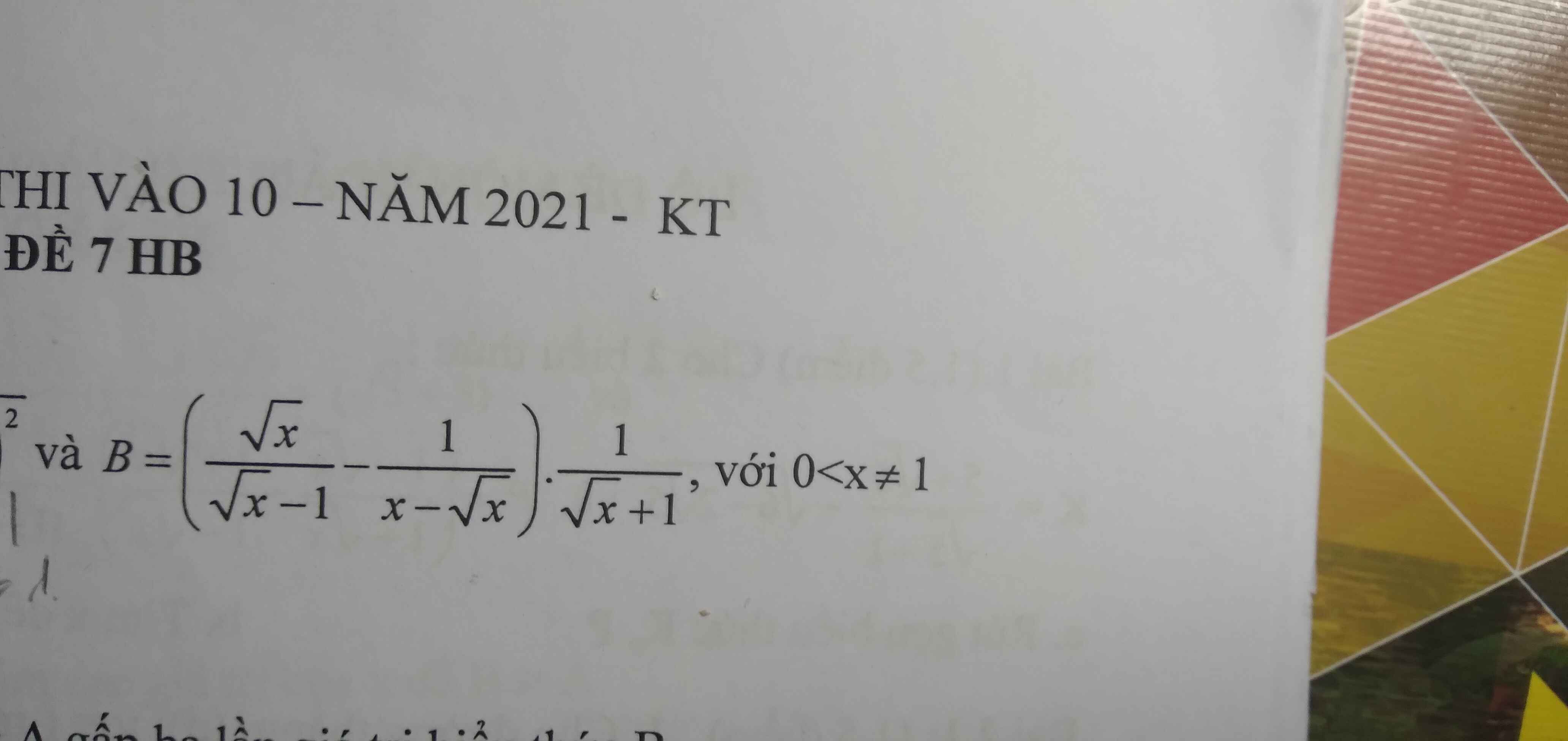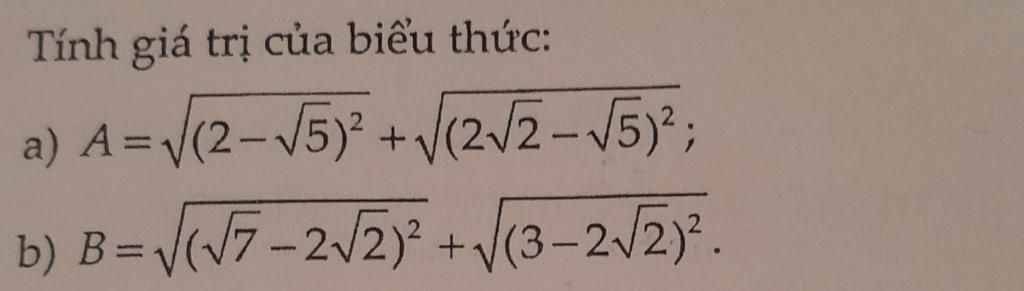\(b,\text{PT hoành độ giao điểm: }-x-7=-3x+1\Leftrightarrow x=4\Leftrightarrow y=-11\Leftrightarrow B\left(4;-11\right)\\ c,\text{Gọi }\left(D_3\right):y=ax+b\left(a\ne0\right)\\ \left(D_3\right)\text{//}\left(D_1\right)\Leftrightarrow a=-1;b\ne-7\Leftrightarrow\left(D_3\right):y=-x+b\\ \left(D_3\right)\cap\left(D_2\right)\text{tại điểm có tung độ }4\Leftrightarrow\left\{{}\begin{matrix}4=-3x+1\\4=-x+b\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\b=3\end{matrix}\right.\\ \text{Vậy }\left(D_3\right):y=-x+3\)

\(d,\Leftrightarrow B\left(4;-11\right)\in\left(D_4\right)\\ \Leftrightarrow8m-4-5m-2=-11\\ \Leftrightarrow3m=-5\Leftrightarrow m=-\dfrac{5}{3}\\ e,\Leftrightarrow\left\{{}\begin{matrix}-x-7=0\\\left(2m-1\right)x-5m-2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-7\\7-14m-5m-2=0\end{matrix}\right.\\ \Leftrightarrow m=\dfrac{5}{19}\)

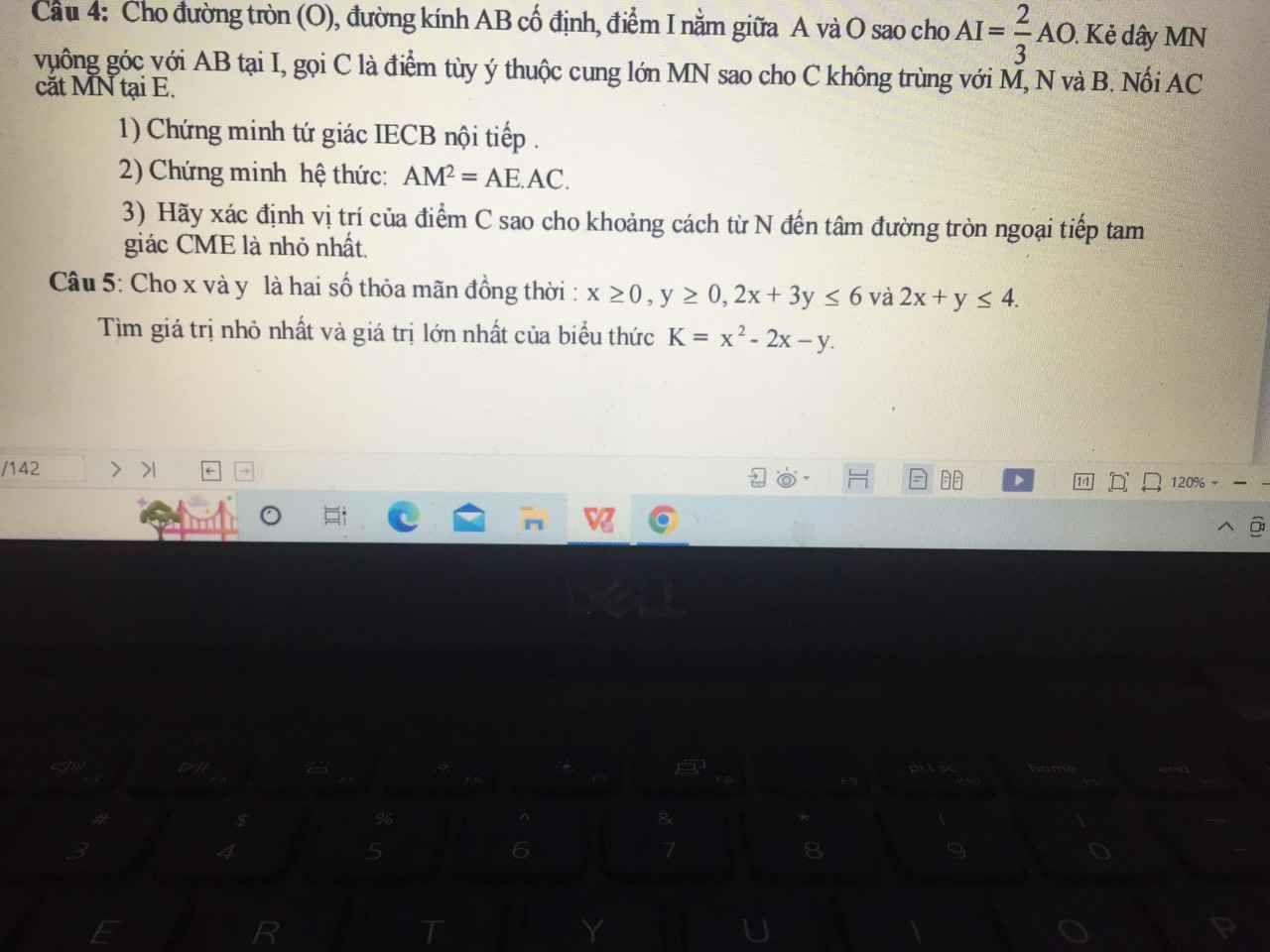
3: góc AMN=góic ACM

=>AM là tiếp tuyến của đường tròn ngoại tiếp ΔECM

=>góc AMB=90 độ

=>Tâm o1 của đường tròn ngoại tiếp ΔECM nằm trên BM

NO1 min khi NO1=d(N;BM)

=>NO1 vuông góc BM

Gọi O1 là chân đường vuông góc kẻ từ N xuống BM

=>O1 là tâm đường tròn ngoại tiếp ΔECM  có bán kính là O1M
=>d(N;tâm đường tròn ngoại tiếp ΔECM) nhỏ nhất khi C là giao của (O1;O1M) với (O) với O1 ;là hình chiếu vuông góc của N trên BM

\(a,A=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1-3\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ A=\dfrac{2x-3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{\left(\sqrt{x}-1\right)\left(2\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}-1}{\sqrt{x}+1}\\ b,A=\dfrac{2\left(\sqrt{x}+1\right)-3}{\sqrt{x}+1}=2-\dfrac{3}{\sqrt{x}+1}\in Z\\ \Leftrightarrow\sqrt{x}+1\inƯ\left(3\right)=\left\{1;3\right\}\left(\sqrt{x}+1\ge1\right)\\ \Leftrightarrow\sqrt{x}\in\left\{0;2\right\}\\ \Leftrightarrow x\in\left\{0;4\right\}\left(tm\right)\)

a) \(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}-\dfrac{3\sqrt{x}+1}{x-1}\)

\(\Rightarrow A=\dfrac{\left(\sqrt{x}+1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{x+2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{x-2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1-3\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{2x-3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{\left(2x-2\sqrt{x}\right)-\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{2\sqrt{x}\left(\sqrt{x}-1\right)-\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{\left(2\sqrt{x}-1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow A=\dfrac{2\sqrt{x}-1}{\sqrt{x}+1}\)

Xét (O) có

AB là tiếp tuyến có B là tiếp điểm

AC là tiếp tuyến có C là tiếp điểm

Do đó: AB=AC

Ta có: OB=OC

nên O nằm trên đường trung trực của BC(1)

Ta có: AB=AC

nên A nằm trên đường trung trực của BC(2)

Từ (1) và (2) suy ra OA là đường trung trực của BC

hay OA\(\perp\)BC

\(B=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{1}{x-\sqrt{x}}\right).\dfrac{1}{\sqrt{x}+1}\)

\(\Rightarrow B=\left(\dfrac{x}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right).\dfrac{1}{\sqrt{x}+1}\)

\(\Rightarrow B=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{1}{\sqrt{x}+1}\)

\(\Rightarrow B=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(\Rightarrow B=\dfrac{1}{\sqrt{x}}\)

Bạn cần làm j với B

\(a,A=\sqrt{\left(2-\sqrt{5}\right)^2}+\sqrt{\left(2\sqrt{2}-\sqrt{5}\right)^2}\\ =\left|2-\sqrt{5}\right|+\left|2\sqrt{2}-\sqrt{5}\right|\\ =\sqrt{5}-2+2\sqrt{2}-5\\ =2\sqrt{2}-2\)

\(b,B=\sqrt{\left(\sqrt{7}-2\sqrt{2}\right)^2}+\sqrt{\left(3-2\sqrt{2}\right)^2}\\ =\left|\sqrt{7}-2\sqrt{2}\right|+\left|3-2\sqrt{2}\right|\\ =2\sqrt{2}-\sqrt{7}+3-2\sqrt{2}\\ =-\sqrt{7}+3\)

a)

\(A=\sqrt{\left(2-\sqrt{5}\right)^2}+\sqrt{\left(2\sqrt{2}-\sqrt{5}\right)^2}\\ =\left|2-\sqrt{5}\right|+\left|2\sqrt{2}-\sqrt{5}\right|\)

\(=\sqrt{5}-2+2\sqrt{2}-\sqrt{5}\) (vì \(2-\sqrt{5}< 0;2\sqrt{2}-\sqrt{5}>0\) )

\(=2\sqrt{2}-2\)

b)

\(B=\sqrt{\left(\sqrt{7}-2\sqrt{2}\right)^2}+\sqrt{\left(3-2\sqrt{2}\right)^2}\\ =\left|\sqrt{7}-2\sqrt{2}\right|+\left|3-2\sqrt{2}\right|\)

\(=2\sqrt{2}-\sqrt{7}+3-2\sqrt{2}\) (vì \(\sqrt{7}-2\sqrt{2}< 0;3-2\sqrt{2}>0\) )

\(=3-\sqrt{7}\)