
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(9,=\dfrac{\sqrt{5}+1-\sqrt{5}+1}{\left(\sqrt{5}-1\right)\left(\sqrt{5}+1\right)}=\dfrac{2}{4}=\dfrac{1}{2}\\ 10,=\dfrac{\sqrt{5}+2+\sqrt{5}-2}{\left(\sqrt{5}-2\right)\left(\sqrt{5}+2\right)}=2\sqrt{5}\\ 11,=\dfrac{8+6\sqrt{2}-8+6\sqrt{2}}{\left(4-3\sqrt{2}\right)\left(4+3\sqrt{2}\right)}=\dfrac{12\sqrt{2}}{-2}=-6\sqrt{2}\\ 12,=\dfrac{2+\sqrt{6}+2-\sqrt{6}}{\left(2-\sqrt{6}\right)\left(2+\sqrt{6}\right)}=\dfrac{4}{-2}=-2\\ 13,=\sqrt{2}-1+\sqrt{2}+3=2\sqrt{2}+2\\ 14,=2-\sqrt{3}+\sqrt{3}-1=1\\ 15,=3-\sqrt{5}+\sqrt{5}-2=1\)

a, \(2\sqrt{3}-\sqrt{4+x^2}=0\Leftrightarrow\sqrt{4+x^2}=2\sqrt{3}\)
\(\Leftrightarrow x^2+4=12\Leftrightarrow x^2=8\Leftrightarrow x=\pm2\sqrt{2}\)
b, \(\sqrt{16x+16}-\sqrt{9x+9}=0\)ĐK : x >= -1
\(\Leftrightarrow4\sqrt{x+1}-3\sqrt{x+1}=0\Leftrightarrow\sqrt{x+1}=0\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
c, \(\sqrt{4\left(x+2\right)^2}=8\Leftrightarrow2\left|x+2\right|=8\Leftrightarrow\left|x+2\right|=4\)
TH1 : \(x+2=4\Leftrightarrow x=2\)
TH2 : \(x+2=-4\Leftrightarrow x=-6\)
c: Ta có: \(\sqrt{4\left(x+2\right)^2}=8\)
\(\Leftrightarrow\left|x+2\right|=4\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=4\\x+2=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-6\end{matrix}\right.\)

Bài 4:
a: Xét tứ giác OBAC có
\(\widehat{OBA}+\widehat{OCA}=180^0\)
Do đó: OBAC là tứ giác nội tiếp
hay O,B,A,C cùng thuộc 1 đường tròn
Bài 5:
\(\sqrt{x+2021}-y^3=\sqrt{y+2021}-x^3\\ \Leftrightarrow\left(\sqrt{x+2021}-\sqrt{y+2021}\right)+\left(x^3-y^3\right)=0\\ \Leftrightarrow\dfrac{x-y}{\sqrt{x+2021}+\sqrt{y+2021}}+\left(x-y\right)\left(x^2+xy+y^2\right)=0\\ \Leftrightarrow\left(x-y\right)\left(\dfrac{1}{\sqrt{x+2021}+\sqrt{y+2021}}+x^2+xy+y^2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-y=0\\\dfrac{1}{\sqrt{x+2021}+\sqrt{y+2021}}+x^2+xy+y^2=0\left(1\right)\end{matrix}\right.\)
Dễ thấy \(\left(1\right)>0\) với mọi x,y
Do đó \(x-y=0\) hay \(x=y\)
\(\Leftrightarrow M=x^2+2x^2-2x^2+2x+2022=x^2+2x+1+2021\\ \Leftrightarrow M=\left(x+1\right)^2+2021\ge2021\)
Dấu \("="\Leftrightarrow x=y=-1\)

b: Xét ΔAHB vuông tại H có HE là đường cao ứng với cạnh huyền AB
nên \(AE\cdot AB=AH^2\left(1\right)\)
Xét ΔAHC vuông tại H có HF là đường cao ứng với cạnh huyền AC
nên \(AF\cdot AC=AH^2\left(2\right)\)
Từ (1) và (2) suy ra \(AE\cdot AB=AF\cdot AC\)


a) \(\sqrt{\left(\sqrt{2}-1\right)^2}-\sqrt{11+6\sqrt{2}}\)
\(=\sqrt{2}-1-3-\sqrt{2}\)
=-4
b) \(\sqrt{\left(1-\sqrt{5}\right)^2}+\sqrt{14-6\sqrt{5}}\)
\(=\sqrt{5}-1+3-\sqrt{5}\)
=2
c) \(\sqrt{21-12\sqrt{3}}-\sqrt{13-4\sqrt{3}}\)
\(=2\sqrt{3}-3-2\sqrt{3}+1\)
=-2

Với \(x\ge\dfrac{5}{2}\)có: \(A=x+\sqrt{2x-5}\ge\dfrac{5}{2}+0=\dfrac{5}{2}\)
Dấu '=' xảy ra \(\Leftrightarrow x=\dfrac{5}{2}\)
\(\Rightarrow A_{min}=\dfrac{5}{2}\)


a) Ta có: \(P=\dfrac{a\sqrt{a}-1}{a-\sqrt{a}}-\dfrac{a\sqrt{a}+1}{a+\sqrt{a}}+\left(\sqrt{a}-\dfrac{1}{\sqrt{a}}\right)\left(\dfrac{3\sqrt{a}}{\sqrt{a}-1}-\dfrac{\sqrt{a}+2}{\sqrt{a}+1}\right)\)
\(=\dfrac{\left(\sqrt{a}-1\right)\left(a+\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}-1\right)}-\dfrac{\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}+1\right)}+\dfrac{a-1}{\sqrt{a}}\cdot\dfrac{3\sqrt{a}\left(\sqrt{a}+1\right)-\left(\sqrt{a}+2\right)\left(\sqrt{a}-1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\dfrac{a+\sqrt{a}+1-a+\sqrt{a}-1}{\sqrt{a}}+\dfrac{3a+3\sqrt{a}-\left(a-\sqrt{a}+2\sqrt{a}-2\right)}{\sqrt{a}}\)
\(=2+\dfrac{3a+3\sqrt{a}-a+\sqrt{a}-2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\sqrt{a}+2a+2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\left(a+2\sqrt{a}+1\right)}{\sqrt{a}}\)
\(=\dfrac{2\left(\sqrt{a}+1\right)^2}{\sqrt{a}}\)
b) Ta có: \(P-6=\dfrac{2\left(\sqrt{a}+1\right)^2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2a+4\sqrt{a}+2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2\left(a-\sqrt{a}+1\right)}{\sqrt{a}}>0\forall a\) thỏa mãn ĐKXĐ
hay P>6
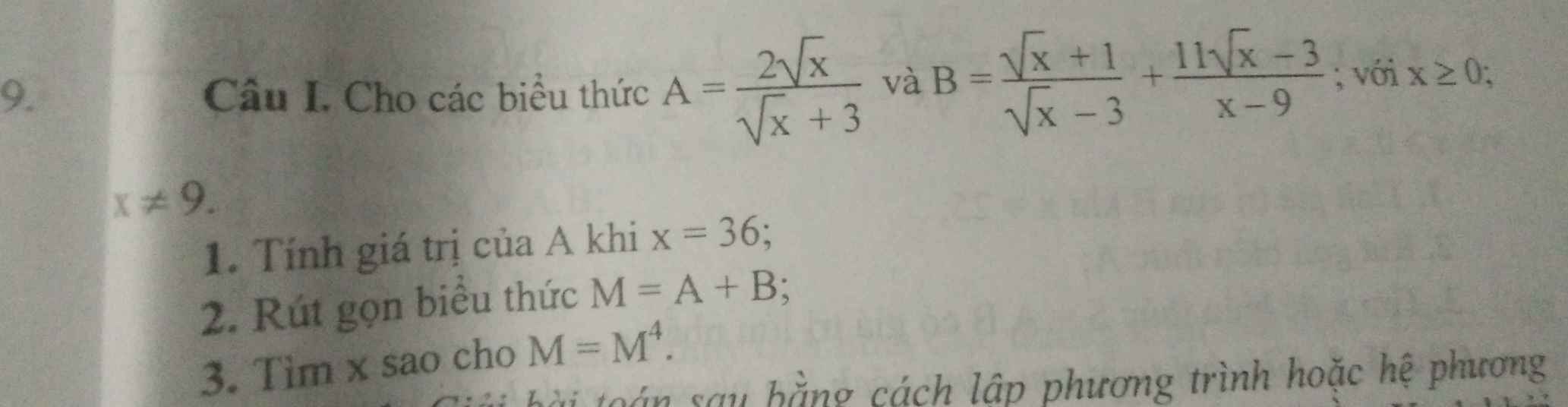
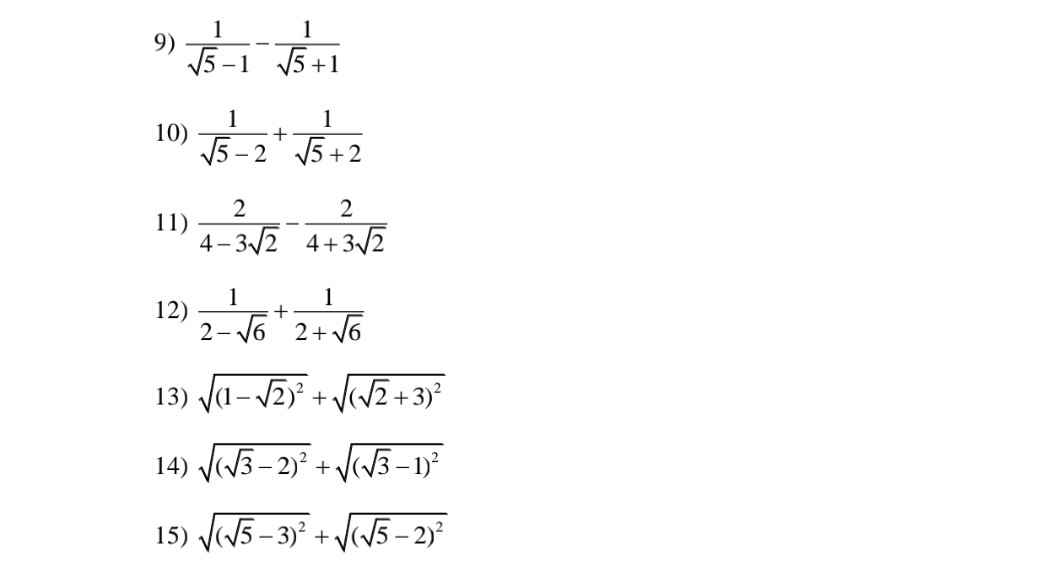
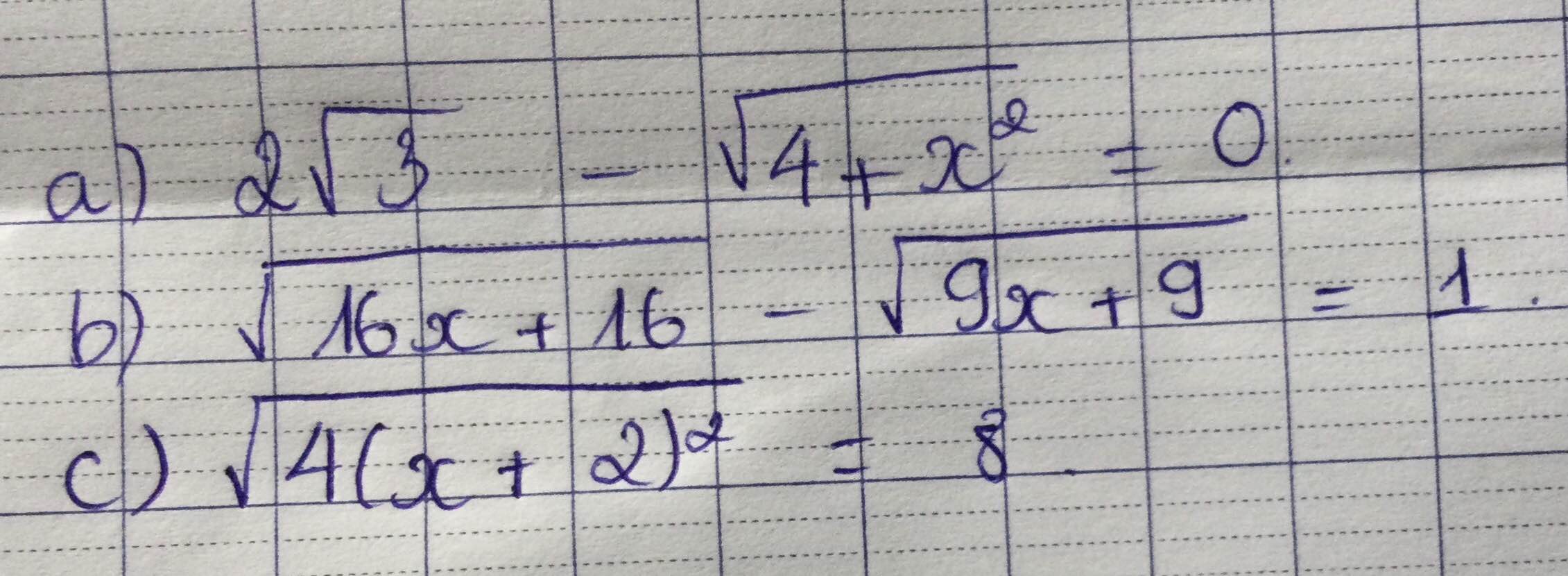 Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.
Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.

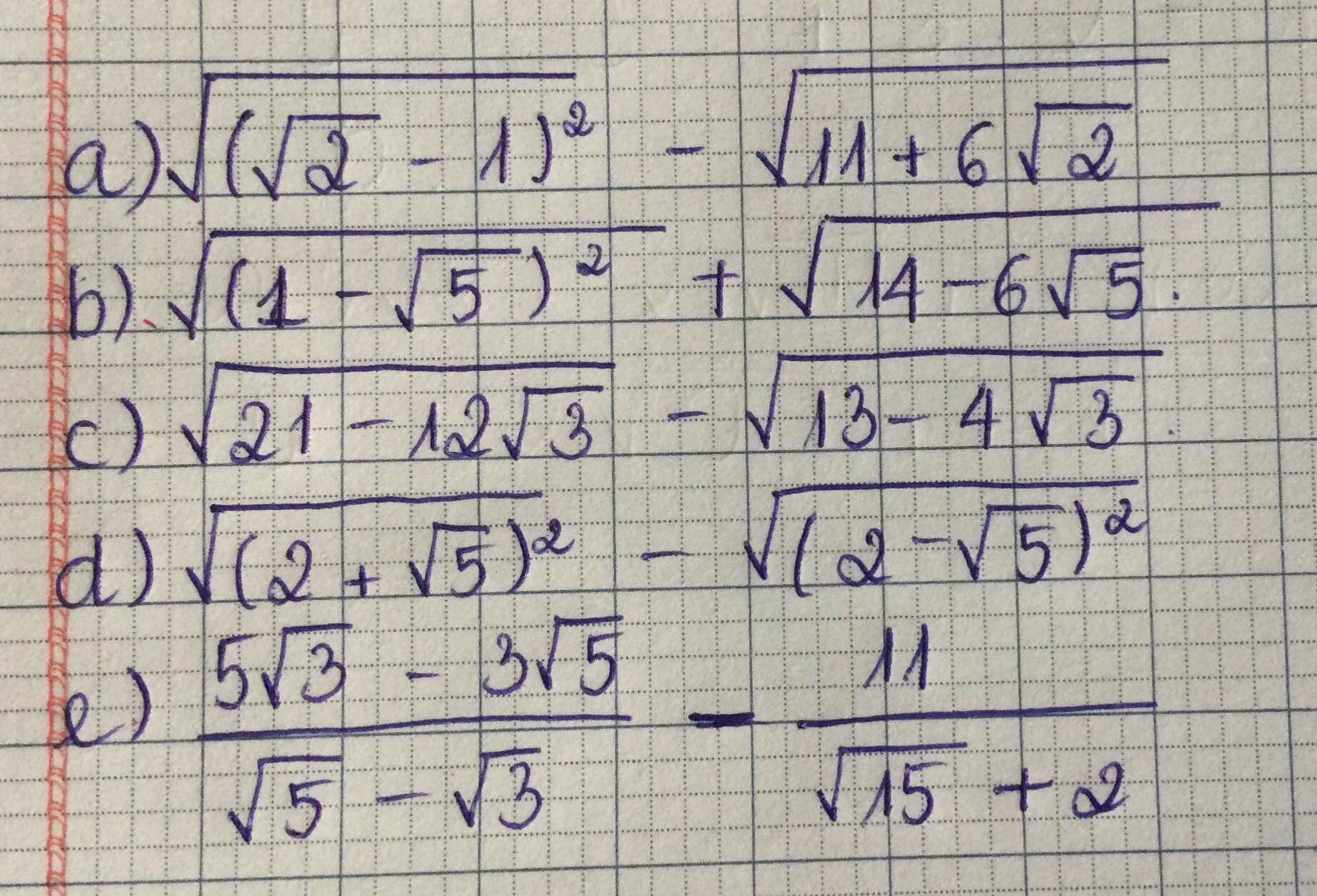 Tính và thu gọn biểu thức.
Tính và thu gọn biểu thức.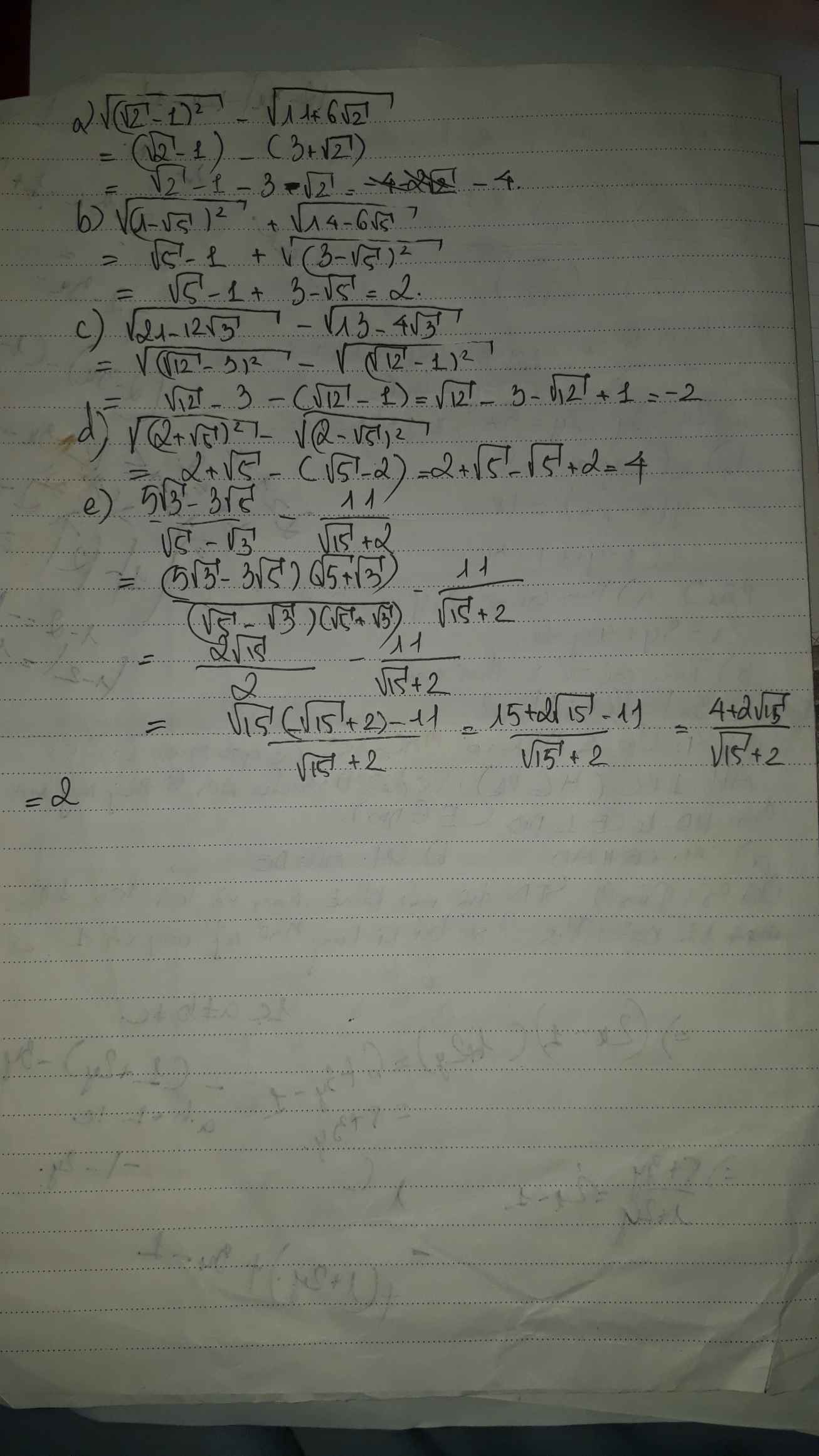


Mình cảm ơn bạn nhiều nhá!!!