
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


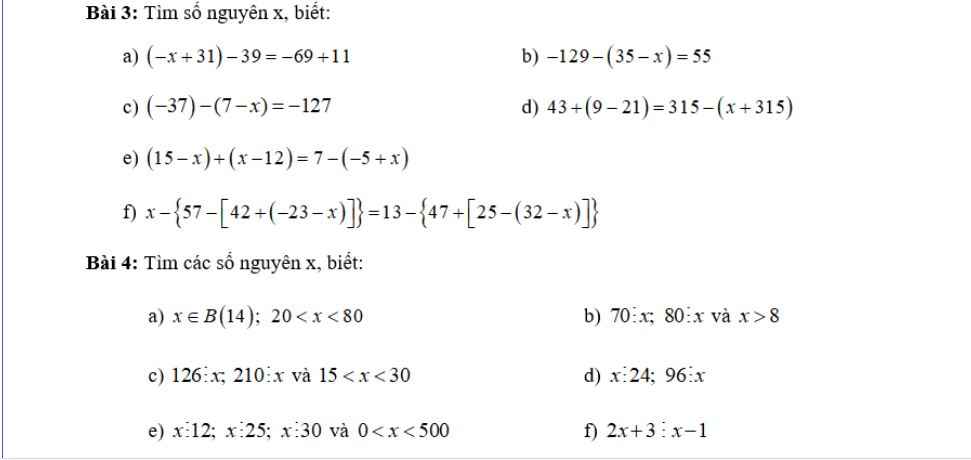
Bài 4:
a: \(x\in\left\{28;42;56;70\right\}\)
b: x=10

ƯC của(−15;+20) là: {±1;±5}
đây là theo hiểu biết của mk thôi nha, ko chép ai đâu^^

Ox và Ox' là 2 tia đối nhau
=> xOx' = 1800
Oy và Oy' là 2 tia đối nhau
=> yOy' = 1800
\(xOx'+3\times yOx'=240^0\)
\(180^0+3\times yOx'=240^0\)
\(3\times yOx'=240^0-180^0\)
\(3\times yOx'=60^0\)
\(yOx'=\frac{60^0}{3}\)
\(yOx'=20^0\)
mà y'Ox = yOx'
=> y'Ox = 200

Khi chia 2021 số bất kỳ cho 2020 ta sẽ có mỗi số có một số dư trong 2020 số dư: 0, 1, 2,…, 2020. Do đó theo nguyên lý Dirichlet phải tồn tại ít nhất hai số có cùng số dư. Hiệu của hai số đó sẽ chia hết cho 2020.

a) \(\Rightarrow\left(n+2\right)+3⋮\left(n+2\right)\)
\(\Rightarrow\left(n+2\right)\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\)
\(\Rightarrow n\in\left\{-5;-3;-1;1\right\}\)
b) \(\Rightarrow\left(n+1\right)+6⋮\left(n+1\right)\)
\(\Rightarrow\left(n+1\right)\inƯ\left(6\right)=\left\{-6;-3;-2;-1;1;2;3;6\right\}\)
\(\Rightarrow n\in\left\{-7;-4;-3;-2;0;1;2;5\right\}\)
c) \(\Rightarrow\left(n+1\right)^2-\left(n+1\right)+13⋮\left(n+1\right)\)
\(\Rightarrow\left(n+1\right)\inƯ\left(13\right)=\left\{-13;-1;1;13\right\}\)
\(\Rightarrow n\in\left\{-14;-2;0;12\right\}\)
d) \(\Rightarrow\left(n+2\right)^2-\left(n+2\right)+7⋮\left(n+2\right)\)
\(\Rightarrow\left(n+2\right)\inƯ\left(7\right)=\left\{-7;-1;1;7\right\}\)
\(\Rightarrow n\in\left\{-9;-3;-1;5\right\}\)


Bài 4:
a. Ta có: $-18a+15b=3(-6a+5b)\vdots 3$
b. Vì $-18a+15b$ chia hết cho $3$ với $a,b$ nguyên, mà $-2015\not\vdots 3$ nên không tồn tại hai số $a,b$ nguyên thỏa mãn $-18a+15b=-2015$
Bài 5:
a.
$23\vdots x-2$
$\Rightarrow x-2\in$ Ư$(23)$
$\Rightarrow x-2\in\left\{\pm 1;\pm 23\right\}$
$\Rightarrow x\in\left\{3; 1; 25; -21\right\}$
b.
$2x+1\in$ Ư$(-12)$, mà $2x+1$ lẻ nên:
$2x+1\in\left\{\pm 1; \pm 3\right\}$
$\Rightarrow x\in\left\{0; -1; 1; -2\right\}$
c.
$x-1\vdots x+2$
$(x+2)-3\vdots x+2$
$3\vdots x+2$
$\Rightarrow x+2\in$ Ư$(3)$
$\Rightarrow x+2\in\left\{\pm 1;\pm 3\right\}$
$\Rightarrow x\in\left\{-1; -3; -5; 1\right\}$

(x - 5) x (x - 7) = 0
=> x - 5 = 0 => x =5
hoặc x - 7 = 0 => x=7
........
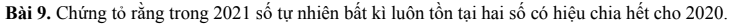


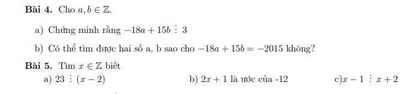
Đặt \(d=\left(16n+3,12n+2\right)\)
Suy ra
\(\hept{\begin{cases}16n+3⋮d\\12n+2⋮d\end{cases}}\Rightarrow3\left(16n+3\right)-4\left(12n+2\right)=1⋮d\Rightarrow d=1\)
Suy ra đpcm.