
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đặt y = f(x) = - 2x2 có đồ thị (C)
và y = g(x) = - 2x2 - 6x + 3 có đồ thị (C')
Ta có :
g(x) = - 2x2 - 6x + 3
= - 2\(\left(x^2+3x-\dfrac{3}{2}\right)\)
= - 2\(\left(x+\dfrac{3}{2}\right)^2\) + \(\dfrac{15}{2}\)
= \(f\left(x+\dfrac{3}{2}\right)+\dfrac{15}{2}\)
Vậy tịnh tiến (C) sang trái \(\dfrac{3}{2}\) đơn vị rồi kéo (C) lên trên \(\dfrac{15}{4}\) đơn vị ta được (C')



\(A\cap B=\varnothing\Leftrightarrow2m-7\le13m+1\)
\(\Leftrightarrow11m\ge-8\Rightarrow m\ge-\dfrac{8}{11}\)
\(\Rightarrow\) Số nguyên m nhỏ nhất là \(m=0\)

Hàm bậc 2 có \(\left\{{}\begin{matrix}a=1>0\\-\dfrac{b}{2a}=6-m\end{matrix}\right.\) nên nghịch biến trên khoảng \(\left(-\infty;6-m\right)\)
Hàm nghịch biến trên khoảng đã cho khi:
\(6-m\ge2\Rightarrow m\le4\)
\(\Rightarrow\) Có 4 giá trị nguyên dương của m

Câu 11:
b: -x^2+x-m<=0 với mọi x
Δ=1^2-4*(-1)*(-m)=1-4m
Để BPT luôn đúng thì 1-4m<=0 và -1<0
=>4m>=1
=>m>=1/4
c: mx^2+mx-1>=0
TH1: m=0
=>-1>=0(vô lý)
=>Nhận)
TH2: m<>0
Δ=m^2-4*m*(-1)=m^2+4m
Để BPT vô nghiệm thì m^2+4m<=0 và m<0
=>-4<=m<=0 và m<0
=>-4<=m<0

\(\left(x\ne3;x\ne\dfrac{1}{2}\right)\)\(\left\{{}\begin{matrix}\dfrac{2}{2x-1}\le\dfrac{1}{3-x}\\\left|x\right|< 1\Leftrightarrow-1< x< 1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2\left(3-x\right)-2x+1}{\left(2x-1\right)\left(3-x\right)}\le0\left(1\right)\\-1< x< 1\end{matrix}\right.\)\(\)
\(\left(1\right)\Leftrightarrow\dfrac{-4x+7}{\left(2x-1\right)\left(3-x\right)}\le0\)\(\Leftrightarrow\dfrac{-4x+7}{-2x^2+7x-3}\le0\Leftrightarrow x\in\left(-\infty;\dfrac{1}{2}\right)\cup[\dfrac{7}{4};3)\)
\(kết\) \(hợp:-1< x< 1\)\(\Rightarrow x\in\left(-1;\dfrac{1}{2}\right)\cup[\dfrac{7}{4};3)\)
\(b,\)\(\left(x-1\right)\left(x-4\right)\left(x-5\right)\left(x-8\right)+35>0\)
\(\Leftrightarrow\left(x^2-9x+8\right)\left(x^2-9x+20\right)+35>0\)
\(đặt:x^2-9x+8=t\ge-\dfrac{49}{4}\)
\(bpt\Leftrightarrow t\left(t+12\right)+35>0\Leftrightarrow t^2+12t+35>0\Leftrightarrow\left[{}\begin{matrix}t< -7\\t>-5\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x^2-9x+8< -7\\x^2-9x+8>-5\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{9-\sqrt{21}}{2}< x< \dfrac{9+\sqrt{21}}{2}\\x\in\left(-\infty;\dfrac{9-\sqrt{29}}{2}\right)\cup\left(\dfrac{9+\sqrt{29}}{2};+\infty\right)\end{matrix}\right.\)
\(c;\)\(\left(x^2+x+4\right)^2+2.4x\left(x^2+x+4\right)+16x^2-x^2>0\)
\(\Leftrightarrow\left(x^2+x+4+4x\right)^2-x^2>0\)
\(\Leftrightarrow\left(x+2\right)^2\left(x^2+6x+4\right)>0\)
\(\Leftrightarrow x^2+6x+4>0\Leftrightarrow....\)
ý d; giống ý b
\(e;bpt\Leftrightarrow\left(x-2\right)\left(x-1\right)\left(x+7\right)\left(x+8\right)+8>0\)
\(\Leftrightarrow\left(x^2+6x-16\right)\left(x^2+6x-7\right)+8>0\)
\(đặt:x^2+6x-7=t\ge-16\Rightarrow t\left(t-9\right)+8>0\)
(làm giống ý b)
\(f;x^4-2x^3+x-2>0\Leftrightarrow\left(x+1\right)\left(x-2\right)\left(x^2-x+1\right)>0\left(do:x^2-x+1=\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0\right)\)
\(\Rightarrow bpt\Leftrightarrow\left(x+1\right)\left(x-2\right)>0\Leftrightarrow\left[{}\begin{matrix}x< -1\\x>2\end{matrix}\right.\)
\(g;h\) dùng bảng phá giá trị tuyệt đối để làm
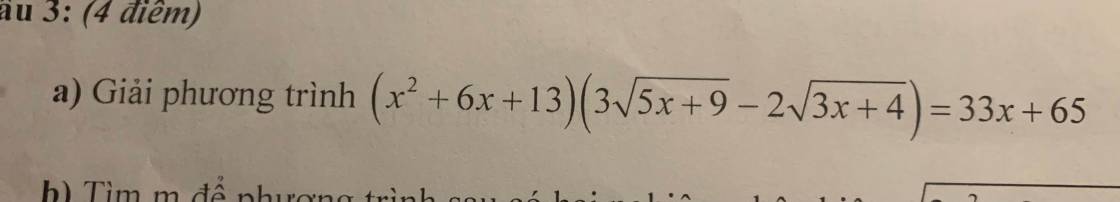





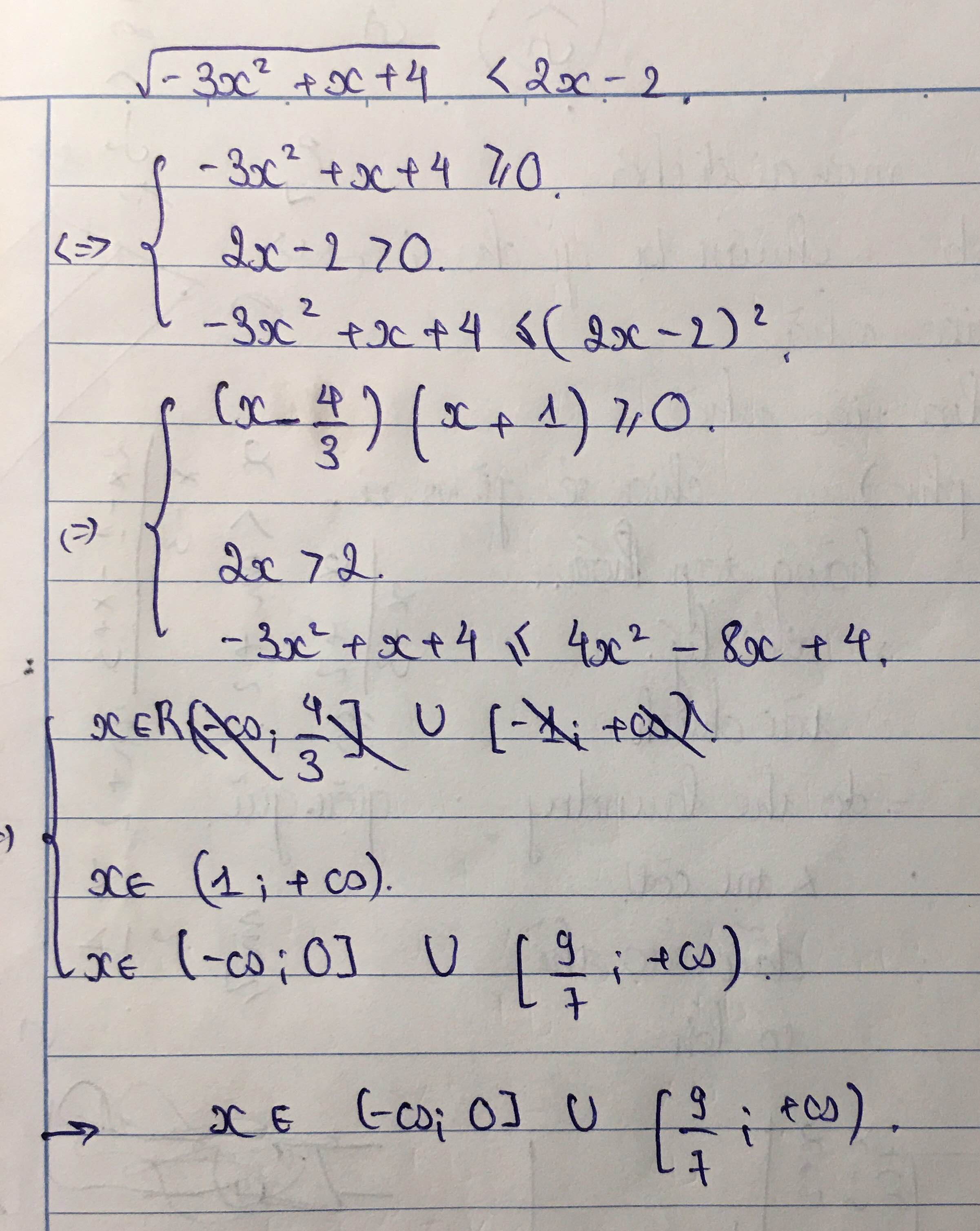

ĐKXĐ: \(x\ge-\dfrac{4}{3}\)
\(\left(x^2+6x+13\right)\left(\dfrac{9\left(5x+9\right)-4\left(3x+4\right)}{3\sqrt{5x+9}+2\sqrt{3x+4}}\right)=33x+65\)
\(\Leftrightarrow\dfrac{\left(x^2+6x+9\right)\left(33x+65\right)}{3\sqrt{5x+9}+2\sqrt{3x+4}}=33x+65\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{65}{33}< -\dfrac{4}{3}\left(ktm\right)\\x^2+6x+9=3\sqrt{5x+9}+2\sqrt{3x+4}\left(1\right)\end{matrix}\right.\)
Xét (1)
\(\Leftrightarrow x^2+x+3\left(x+3-\sqrt{5x+9}\right)+2\left(x+2-\sqrt{3x+4}\right)=0\)
\(\Leftrightarrow x^2+x+\dfrac{3\left(x^2+x\right)}{x+3+\sqrt{5x+9}}+\dfrac{2\left(x^2+x\right)}{x+2+\sqrt{3x+4}}=0\)
\(\Leftrightarrow\left(x^2+x\right)\left(1+\dfrac{3}{x+3+\sqrt{5x+9}}+\dfrac{2}{x+2+\sqrt{3x+4}}\right)=0\)
\(\Leftrightarrow x^2+x=0\) (ngoặc phía sau luôn dương khi \(x\ge-\dfrac{4}{3}\))
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)