
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


x + 3y = 10 <=> x = 10 - 3y thay vào D ta được:
D = (10 - 3y)2 + y2 = 100 - 60y + 9y2 + y2
D = 10y2 - 60y + 100 = 10(y2 - 6y + 10)
D = 10(y2 -2y3 + 9 + 1) = 10[(y - 3)2 + 1]
D = 10(y - 3)2 + 10 \(\ge\)10
Dấu "=" xảy ra khi: y - 3 = 0 <=> y = 3
=> x = 10 - 3y = 10 - 3.3= 1
Vậy gtnn D = 10 khi x = 1, y = 3

Bài 1:
\(A=x^2+6x+9+x^2-10x+25\)
\(=2x^2+4x+34\)
\(=2\left(x^2+2x+17\right)\)
\(=2\left(x+1\right)^2+32>=32\forall x\)
Dấu '=' xảy ra khi x=-1

A = -x² - 6x + 1
= -(x² + 6x - 1)
= -(x² + 6x + 9 - 10)
= -[(x + 3)² - 10]
= -(x + 3)² + 10
Do (x + 3)² ≥ 0 với mọi x ∈ R
⇒ -(x + 3)² ≤ 0 với mọi x ∈ R
⇒ -(x + 3)² + 10 ≤ 10 với mọi x ∈ R
Vậy GTLN của A là 10 khi x = -3
\(A=-x^2-6x+1\)
\(A=-\left(x^2+6x-1\right)\)
\(A=-\left(x^2+6x+9-10\right)\)
\(A=-\left(x^2+2\cdot x\cdot3+3^2\right)+10\)
\(A=-\left(x+3\right)^2+10\)
Có: \(\left(x+3\right)^2\ge0\forall x\Rightarrow-\left(x+3\right)^2\le0\)
\(\Rightarrow-\left(x+3\right)^2+10\le10\)
\(\Rightarrow A\le10\)
Dấu "=" xảy ra khi \(\left(x+3\right)^2=0\Leftrightarrow x+3=0\)
\(\Leftrightarrow x=-3\)
Vậy: \(A_{min}=10\Leftrightarrow x=-3\)

-x^2+6x-11
=-(x^2-6x+11)
=-(x^2-6x+9+2)
=-(x-3)^2-2<=-2
Dấu = xảy ra khi x=3

A+1 = x^2+6x+9/x^2+1 = (x+3)^2/x^2+1 >= 0
=> A >= -1
Dấu "=" xảy ra <=> x+3=0 <=> x=-3
Vậy GTNN của A = -1 <=> x=-3
Tk mk nha

\(A=\left[\frac{6x^2}{x^3-1}-\frac{2x-2}{x^2+x+1}-\frac{1}{x-1}\right]:\frac{x^2+9}{\left(x-1\right)\left(9-4x\right)}\)
\(=\left[\frac{6x^2}{x^3-1}-\frac{\left(2x-2\right)\left(x-1\right)}{\left(x^2+x+1\right)\left(x-1\right)}-\frac{x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\right]\cdot\frac{\left(x-1\right)\left(9-4x\right)}{x^2+9}\)
\(=\frac{6x^2-\left(2x^2-4x+2\right)-x^2-x-1}{\left(x^2+x+1\right)\left(x-1\right)}\cdot\frac{\left(x-1\right)\left(9-4x\right)}{x^2+9}\)
\(=\frac{5x^2-2x^2+4x-2-x-1}{\left(x^2+x+1\right)}\cdot\frac{\left(9-4x\right)}{x^2+9}\)
\(=\frac{3x^2+3x-3}{\left(x^2+x+1\right)}\cdot\frac{\left(9-4x\right)}{x^2+9}\)
Biểu thức A bạn viết đúng chưa?
Tìm giá trị nhỏ nhất của biểu thức:
\(A=4x^2+3y^2-6xy+6x-12y+20\)
Mình cần gấp, các bạn giúp mình nhé.

\(A=4x^2+3y^2-6xy+6x-12y+20\)
\(A=3\left(x^2-2xy+y^2\right)+6x-12y+x^2+20\)
\(A=3\left[\left(x-y\right)^2+4\left(x-y\right)+4\right]+\left(x^2-6x+9\right)-1\)
\(A=3\left(x-y+2\right)^2+\left(x-3\right)^2-1\ge-1\)
Dấu bằng xảy ra tại x=3;y=5

A=x^2-2.x.3+9+2000
=(x-3)^2+2000
Bước này bạn tự suy luận ra rồi kết luận là xong
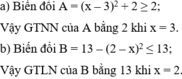
Ta có A = x2 - 6x + 10
= x2 - 6x + 9 + 1
= (x - 3)2 + 1 \(\ge\)1
Dấu "=" xảy ra <=>x - 3 = 0 => x = 3
Vậy Min A = 1 <=> x = 3