Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a)4x2-9=0
⇔ (2x-3)(2x+3)=0
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{3}{2}\\x=-\dfrac{3}{2}\end{matrix}\right.\)
b)(x+5)2-(x-1)2=0
⇔ (x+5-x+1)(x+5+x-1)=0
⇔ 12(x+2)=0
⇔ x=-2
c)x2-6x-7=0
⇔ x2-7x+x-7=0
⇔ x(x-7)+(x-7)=0
⇔ (x-7)(x+1)=0
\(\Leftrightarrow\left\{{}\begin{matrix}x=7\\x=-1\end{matrix}\right.\)
d)(x+1)2-(2x-1)2=0
⇔ (x+1-2x+1)(x+1+2x-1)=0
⇔3x(2-x)=0
\(\Leftrightarrow\left\{{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
a, 4x2 - 9 = 0
<=> 4x2 = 9
<=> x2 = \(\dfrac{9}{4}\) => x = \(\sqrt{\dfrac{9}{4}}\)
b, (x + 5 )2 - ( x - 1 )2 = 0
<=> ( x+5-x+1 )(x+5+x-1) = 0
<=> 6(2x+4) = 0
<=> 12x+24=0
<=> 12x = -24
<=> x = -2
c, x2-6x-7=0
<=> x2+x-7x-7=0
<=> x(x+1)-7(x+1)=0
<=> (x-7)(x+1)=0
=> x+7=0 hoặc x+1=0
+ x-7=0 => x=7
+ x+1=0 => x=-1
d, \(\left(x+1\right)^2-\left(2x-1\right)^2=0\)
<=> \(\left(x+1-2x+1\right)\left(x+1+2x-1\right)=0\)
<=> (-x+2).3x=0
=> x=0 hoặc (-x+2).3=0
+ (-x+2).3=0 => -3x+6=0 => x=-2

a)\(6x^2+5x-6=0\)
\(\Leftrightarrow6x^2-4x+9x-6=0\)
\(\Leftrightarrow2x\left(3x-2\right)+3\left(3x-2\right)=0\)
\(\Leftrightarrow\left(2x+3\right)\left(3x-2\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}2x+3=0\\3x-2=0\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x=-\frac{3}{2}\\x=\frac{2}{3}\end{array}\right.\)
b)\(6x^2-13x+6=0\)
\(\Leftrightarrow6x^2-4x-9x+6=0\)
\(\Leftrightarrow2x\left(3x-2\right)-3\left(3x-2\right)=0\)
\(\Leftrightarrow\left(2x-3\right)\left(3x-2\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}2x-3=0\\3x-2=0\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x=\frac{3}{2}\\x=\frac{2}{3}\end{array}\right.\)
c)\(10x^2-13x-3=0\)
\(\Leftrightarrow10x^2-15x+2x-3=0\)
\(\Leftrightarrow5x\left(2x-3\right)+\left(2x-3\right)=0\)
\(\Leftrightarrow\left(2x-3\right)\left(5x+1\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}2x-3=0\\5x+1=0\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x=\frac{3}{2}\\x=-\frac{1}{5}\end{array}\right.\)
d)\(20x^2+19x-3=0\)
\(\Delta=19^2-\left(-4\left(20.3\right)\right)=601\)
\(\Rightarrow x_{1,2}=\frac{-19\pm\sqrt{601}}{40}\)
e)\(3x^2-x+6=0\)
\(\Delta=\left(-1\right)^2-4\left(3.6\right)=-71< 0\)
Suy ra vô nghiệm


a)
\(\begin{array}{l}6x + 4 = 0\\\,\,\,\,\,\,\,\,6x = - 4\\\,\,\,\,\,\,\,\,\,\,x = \left( { - 4} \right):6\\\,\,\,\,\,\,\,\,\,\,x = - \frac{2}{3}.\end{array}\)
Vậy phương trình có nghiệm \(x = - \frac{2}{3}.\)
b)
\(\begin{array}{l} - 14x - 28 = 0\\\,\,\,\,\,\,\,\,\, - 14x = 28\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = 28:\left( { - 14} \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = - 2\end{array}\)
Vậy phương trình có nghiệm \(x = - 2.\)
c)
\(\begin{array}{l}\frac{1}{3}x - 5 = 0\\\,\,\,\,\,\,\,\frac{1}{3}x = 5\\\,\,\,\,\,\,\,\,\,\,\,x = 5:\frac{1}{3}\\\,\,\,\,\,\,\,\,\,\,\,x = 15.\end{array}\)
Vậy phương trình có nghiệm \(x = 15\).
d)
\(\begin{array}{l}\,3y - 1 = - y + 19\\3y + y = 19 + 1\\\,\,\,\,\,\,\,4y = 20\\\,\,\,\,\,\,\,\,\,\,y = 20:5\\\,\,\,\,\,\,\,\,\,\,y = 4.\end{array}\)
Vậy phương trình có nghiệm \(y = 4\).
e)
\(\begin{array}{l} - 2\left( {z + 3} \right) - 5 = z + 4\\\,\,\, - 2z - 6 - 5 = z + 4\\\,\,\,\,\,\,\,\, - 2z - 11 = z + 4\\\,\,\,\,\,\,\,\,\,\, - 2z - z = 4 + 11\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - 3z = 15\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,z = 15:\left( { - 3} \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,z = - 5.\end{array}\)
Vậy phương trình có nghiệm \(z = - 5\).
g)
\(\begin{array}{l}3\left( {t - 10} \right) = 7\left( {t - 10} \right)\\\,\,\,\,3t - 30 = 7t - 70\\\,\,\,\,\,3t - 7t = - 70 + 30\\\,\,\,\,\,\,\,\,\,\, - 4t = - 40\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,t = \left( { - 40} \right):\left( { - 4} \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,t = 10.\end{array}\)
Vậy phương trình có nghiệm \(t = 10\).

a, \(x^2-x-14x+14=0\)
\(=>x\left(x-1\right)-14\left(x-1\right)=0\)
\(=>\left(x-14\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-14=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=14\\x=1\end{matrix}\right.\)
b, \(x^2+2x+7x+14=0\)
\(=>x\left(x+2\right)+7\left(x+2\right)=0\)
\(=>\left(x+7\right)\left(x+2\right)=0\)
\(< =>\left\{{}\begin{matrix}x+7=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-7\\x=-2\end{matrix}\right.\)
c, \(6x^2-6x-5x+5=0\)
\(=>6x\left(x-1\right)-5\left(x-1\right)=0\)
\(=>\left(6x-5\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}6x-5=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{5}{6}\\x=1\end{matrix}\right.\)
d, \(6x^2+3x+10x+5=0\)
\(=>3x\left(2x+1\right)+5\left(2x+1\right)=0\)
\(=>\left(3x+5\right)\left(2x+1\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x+5=0\\2x+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{-5}{3}\\x=\dfrac{-1}{2}\end{matrix}\right.\)
e, \(10x^2+10x+3x+3=0\)
\(=>10x\left(x+1\right)+3\left(x+1\right)=0\)
\(=>\left(10x+3\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}10x+3=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{-3}{10}\\x=-1\end{matrix}\right.\)
CHÚC BẠN HỌC TỐT...

Giải như sau.
(1)+(2)⇔x2−2x+1+√x2−2x+5=y2+√y2+4⇔(x2−2x+5)+√x2−2x+5=y2+4+√y2+4⇔√y2+4=√x2−2x+5⇒x=3y(1)+(2)⇔x2−2x+1+x2−2x+5=y2+y2+4⇔(x2−2x+5)+x2−2x+5=y2+4+y2+4⇔y2+4=x2−2x+5⇒x=3y
⇔√y2+4=√x2−2x+5⇔y2+4=x2−2x+5, chỗ này do hàm số f(x)=t2+tf(x)=t2+t đồng biến ∀t≥0∀t≥0
Công việc còn lại là của bạn !
\(\left(x+6\right)\left(2x+1\right)=0\)
<=> \(\orbr{\begin{cases}x+6=0\\2x+1=0\end{cases}}\)
<=> \(\orbr{\begin{cases}x=-6\\x=-\frac{1}{2}\end{cases}}\)
Vậy....
hk tốt
^^

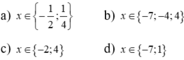
a) \(5x^3-125=0\)
\(\Leftrightarrow5x^3=125\)
\(\Leftrightarrow x^3=25\)
\(\Leftrightarrow x^3=25\)
\(\Leftrightarrow x=\sqrt[3]{25}\)
c) \(6x+13x+5=0\)
\(\Leftrightarrow19x+5=0\)
\(\Leftrightarrow19x=-5\)
\(\Leftrightarrow x=\dfrac{-5}{19}\)