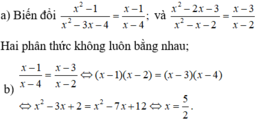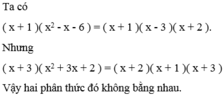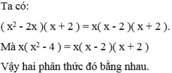
a) `(x^3-x^2)/(x^3-2x^2+x)`

`=(x^2(x-1))/(x(x-1)(x-1))`

`=x/(x-1)`

`=>` 2 phân thức bằng nhau.

b) `(x^2+2x+1)/(2x^2-2)`

`=((x+1)(x+1))/(2(x+1)(x-1))`

`=(x+1)/(2(x-1))`

`=(x+1)/(2x-2)`

`=>` 2 phân thức bằng nhau

a) Ta có: \(\dfrac{x^3-x^2}{x^3-2x^2+x}\)

\(=\dfrac{x^2\left(x-1\right)}{x\left(x^2-2x+1\right)}\)

\(=\dfrac{x\cdot\left(x-1\right)}{\left(x-1\right)^2}=\dfrac{x}{x-1}\)

b) Ta có: \(\dfrac{x^2+2x+1}{2x^2-2}\)

\(=\dfrac{\left(x+1\right)^2}{2\left(x+1\right)\left(x-1\right)}\)

\(=\dfrac{x+1}{2x-2}\)

\(\frac{2}{6}x-4=\frac{1}{3}x-4\ne\frac{1}{3}x-2\)

\(\left\{{}\begin{matrix}\dfrac{x^2-2x-3}{x^2+x}=\dfrac{\left(x+1\right)\left(x-3\right)}{x\left(x+1\right)}=\dfrac{x-3}{x}\\\dfrac{x-3}{x}\\\dfrac{x^2-4x+3}{x^2-x}=\dfrac{\left(x-3\right)\left(x-1\right)}{x\left(x-1\right)}=\dfrac{x-3}{x}\end{matrix}\right.\)

Vậy \(\dfrac{x^2-2x-3}{x^2+x}=\dfrac{x-3}{x}=\dfrac{x^2-4x+3}{x^2-x}\)

\(ĐK:x\ne0;x\ne\pm1\\ \dfrac{x^2-2x-3}{x^2+x}=\dfrac{\left(x+1\right)\left(x-3\right)}{x\left(x+1\right)}=\dfrac{x-3}{x}\\ \dfrac{x^2-4x+3}{x^2-x}=\dfrac{\left(x-1\right)\left(x-3\right)}{x\left(x-1\right)}=\dfrac{x-3}{x}\)

Do đó 3 phân thức trên bằng nhau

Ta có:

\(\dfrac{x^2-4}{x+1}\)

\(=\dfrac{\left(x+2\right)\left(x-2\right)}{x+1}\)

Và:

\(\dfrac{x+2}{2x}\)

\(=\dfrac{\left(x+2\right)\left(x-2\right)}{2x\left(x-2\right)}\)

Vậy ta đã biến đổi hai phân thức đó để chúng bằng phân thức cũ và có tủ bằng nhau

Mik cảm ơn ạ

Bài 1: 

c: ĐKXĐ: \(x\notin\left\{-1;3\right\}\)

`a, (xy^2)/(xy+y) = (xy^2)/(y(x+1))`

`=(xy)/(x+1)`

Vậy `2` cặp phân thức bằng nhau.

`b, (xy-y)/x = (y(x-1))/x = (y^2(x-1))/(xy)`

`(xy-x)/y = (x(y-1))/y = (x^2(y-1))/(xy)`

Vậy `2` đa thức không bằng nhau