Giúp mình giải 4 bài này với mừng cần gấp lam
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Thời gian Làm làm hai bài toán 1 và 2 lầ: 5 phút 12 giây + 6 phút 18 giây = 11 phút 30 giây
Trung bình mỗi bài Lam làm trong : (11 phút 30 giây - 1 phút 28 giây) : 2 = 5 phút 1 giây
Thời gian Lam làm bài 3 là: 5 phút 1 giây - 1 phút 28 giây = 3 phút 33 giây

\(sin\left(\dfrac{\pi}{2}-x\right)+cot^2x=cosx+\dfrac{cos^2x}{sin^2x}=cosx+\dfrac{cos^2x}{1-cos^2x}=a+\dfrac{a^2}{1-a^2}\)
\(=\dfrac{-a^3+a^2+a}{1-a^2}\)
\(\Rightarrow\left\{{}\begin{matrix}m=-1\\n=1\\\end{matrix}\right.\) \(\Rightarrow P=-3\)

A = 4/1.3 + 4/3.5 + 4/5.7 + ... + 4/11.13
A = 2.(2/1.3 + 2/3.5 + 2/5.7 + ... + 2/11.13)
A = 2.(1 - 1/3 + 1/3 - 1/5 + 1/5 - 1/7 + ... + 1/11 - 1/13)
A = 2.(1 - 1/13)
A = 2.12/13
A = 24/13

ta có:
\(-\frac{x}{2}=\frac{-y}{4}=\frac{6}{-8}\)
=>\(\frac{-x}{2}=\frac{6}{-8}\)
=>-8.(-x)=6.2
=>8x=12
=>x=3/2
lại có:
\(\frac{-y}{4}=\frac{6}{-8}\)
=>-8.(-y)=6.4
=>8y=24
=>y=3
Vậy x=3/2; y=3

(3x-4-x-1)(3x-4+x+1)=0
(2x-5)(4x-3)=0
2x-5 = 0 hoặc 4x-3=0
2x=5 hoặc 4x=3
x=5/2 hoặc x=3/4

\(R_{tđ}=\dfrac{R_1\cdot R_2}{R_1+R_2}=\dfrac{24\cdot12}{24+12}=8\Omega\)
\(I=\dfrac{U}{R}=\dfrac{12}{8}=1,5A\)
\(P=\dfrac{U^2}{R}=\dfrac{12^2}{8}=18W\)
\(Q_{tỏa1}=A_1=U_1\cdot I_1\cdot t=12\cdot\dfrac{12}{24}\cdot1\cdot3600=21600J\)
\(Q_{tỏa2}=A_2=U_2\cdot I_2\cdot t=12\cdot\dfrac{12}{12}\cdot1\cdot3600=43200J\)

 giúp mình với mình cần gấp và mình cần lời giải bài này
giúp mình với mình cần gấp và mình cần lời giải bài này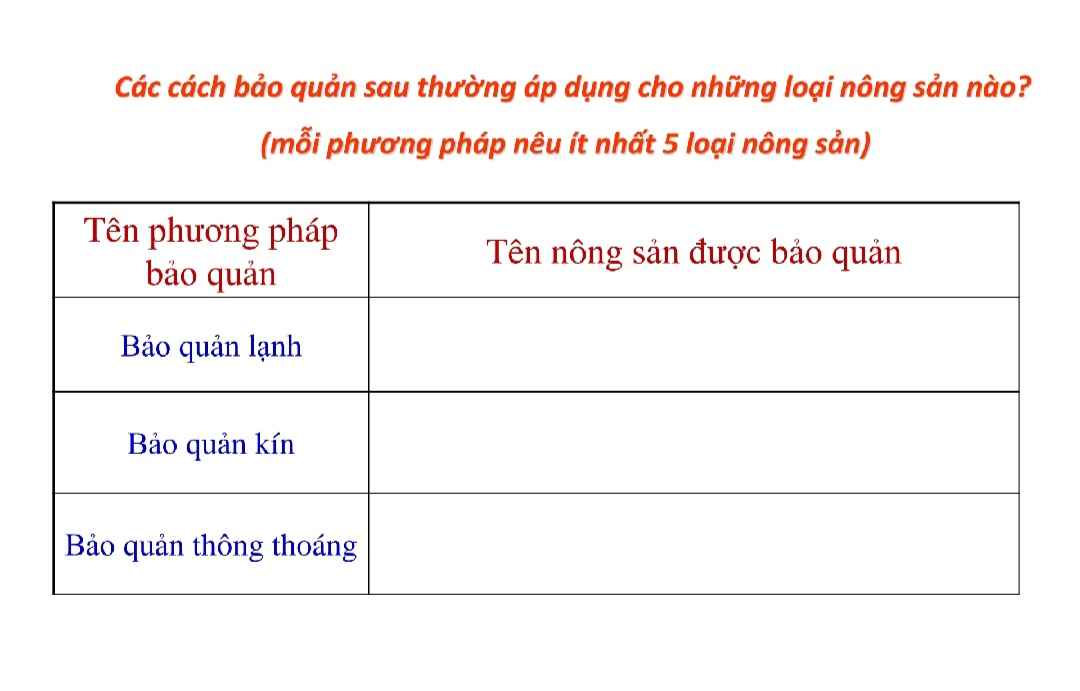


a. \(0< a< 90^0\Rightarrow cosa>0\)
\(cosa=\sqrt{1-sin^2a}=\dfrac{5}{13}\)
\(sin2a=2sina.cosa=\dfrac{120}{169}\)
\(cos2a=2cos^2a-1=2.\left(\dfrac{5}{13}\right)^2-1=-\dfrac{119}{169}\)
b.
\(\dfrac{3\pi}{2}< a< 2\pi\Rightarrow sina< 0\) \(\Rightarrow sina=-\sqrt{1-cos^2a}=-\dfrac{\sqrt{15}}{8}\)
\(sin2a=2sina.cosa=-\dfrac{7\sqrt{15}}{32}\)
\(cos2a=2cos^2a-1=2\left(\dfrac{7}{8}\right)^2-1=\dfrac{17}{32}\)
c.
\(\dfrac{\pi}{2}< a< \pi\Rightarrow sina>0\)
\(\Rightarrow sina=\sqrt{1-cos^2a}=\dfrac{\sqrt{2}}{2}\)
\(sin2a=2sina.cosa=-1\)
\(cos2a=2cos^2a-1=0\)
d.
\(\pi< a< \dfrac{3\pi}{2}\Rightarrow cosa< 0\)
\(\Rightarrow cosa=-\sqrt{1-sin^2a}=-\dfrac{1}{2}\)
\(sin2a=2sina.cosa=\dfrac{\sqrt{3}}{2}\)
\(cos2a=2cos^2a-1=-\dfrac{1}{2}\)