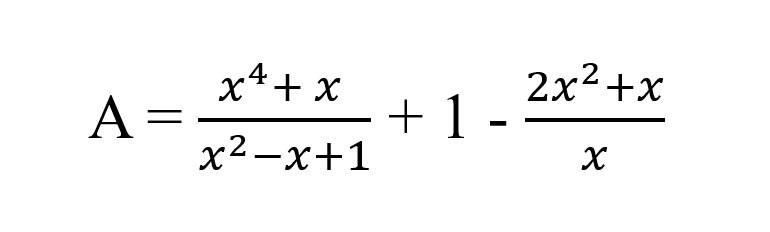
Cho biểu thức A ( như hình )
a) Rút gọn A
b) Tìm giá trị của x để A = 6
c) Tìm giá trị nhỏ nhất của A
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
VN
20 tháng 1 2017
Làm khâu rút gọn thôi
\(=\frac{15}{x+2}+\frac{42}{3x+6}\)
\(=\frac{15}{x+2}+\frac{42}{3\left(x+2\right)}\)
\(=\frac{3.15+42}{3\left(x+2\right)}\)
\(=\frac{87}{3\left(x+2\right)}\)
\(=\frac{29}{x+2}\)
VN
20 tháng 1 2017
Câu b có phải để tử chia hết cho mẫu không nhỉ? Không chắc thôi để ngkh làm
XO
21 tháng 8 2023
ĐKXĐ : \(x\ne0;x\ne\pm1\)
a) Bạn ghi lại rõ đề.
b) \(B=\dfrac{x-1}{x+1}+\dfrac{3x-x^2}{x^2-1}=\dfrac{x-1}{x+1}+\dfrac{3x-x^2}{\left(x-1\right).\left(x+1\right)}\)
\(=\dfrac{\left(x-1\right)^2+3x-x^2}{\left(x-1\right).\left(x+1\right)}=\dfrac{x+1}{\left(x-1\right).\left(x+1\right)}=\dfrac{1}{x-1}\)
c) \(P=A.B=\dfrac{x^2+x-2}{x.\left(x-1\right)}=\dfrac{\left(x-1\right).\left(x+2\right)}{x\left(x-1\right)}=\dfrac{x+2}{x}=1+\dfrac{2}{x}\)
Không tồn tại Min P \(\forall x\inℝ\)
nhận giá trị là số nguyên.
20 tháng 7 2021
a) Ta có: \(P=\dfrac{x^2-\sqrt{x}}{x+\sqrt{x}+1}-\dfrac{2x+\sqrt{x}}{\sqrt{x}}+\dfrac{2\left(x-1\right)}{\sqrt{x}-1}\)
\(=\sqrt{x}\left(\sqrt{x}-1\right)-2\sqrt{x}-1+2\left(\sqrt{x}+1\right)\)
\(=x-\sqrt{x}-2\sqrt{x}-1+2\sqrt{x}+2\)
\(=x-\sqrt{x}+1\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
3 tháng 7
a; A = \(\dfrac{1}{15}\) \(\times\) \(\dfrac{225}{x+2}\) + \(\dfrac{3}{14}\) \(\times\) \(\dfrac{196}{3x+6}\) (đk \(x\) ≠ - 2)
A = \(\dfrac{15}{x+2}\) + \(\dfrac{3\times14}{3\times\left(x+2\right)}\)
A = \(\dfrac{15}{x+2}\) + \(\dfrac{14}{x+2}\)
A = \(\dfrac{29}{x+2}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
3 tháng 7
b; A = \(\dfrac{29}{x+2}\) (-2 ≠ \(x\) \(\in\) Z)
A \(\in\) Z ⇔ 29 ⋮ \(x\) + 2
\(x\) + 2 \(\in\) Ư(29) = {-29; - 1; 1; 29}
Lập bảng ta có:
| \(x\) + 2 | - 29 | - 1 | 1 | 29 |
| \(x\) | -31 | -3 | -1 | 27 |
Theo bảng trên ta có: \(x\) \(\in\) {- 31; -3; -1; 27}
Vậy \(x\) \(\in\) {-31; -3; -1; 27}
a) \(A=\dfrac{x^4+x}{x^2-x+1}+1-\dfrac{2x^2+x}{x}\)
\(A=\dfrac{x.\left(x^3+1\right)}{x^2-x+1}+1-\dfrac{x.\left(2x+1\right)}{x}\)
\(A=\dfrac{x.\left(x+1\right).\left(x^2-x+1\right)}{x^2-x+1}+1-\left(2x+1\right)\)
\(A=x\left(x+1\right)+1-2x-1\)
\(A=x^2+x-2x=x^2-x\)
b) \(A=6\)
\(\Leftrightarrow x^2-x=6\)
\(\Leftrightarrow x^2-x-6=0\)\(\)
\(\Leftrightarrow\left(x-3\right)\left(x+2\right)=0\)
\(\Leftrightarrow x-3=0\) hay \(x+2=0\)
\(\Leftrightarrow x=3\) hay \(x=-2\)
c) \(A=x^2-x\)
\(A=x^2-x+\dfrac{1}{4}-\dfrac{1}{4}\)
\(A=\left(x-\dfrac{1}{2}\right)^2-\dfrac{1}{4}\)
mà \(\left(x-\dfrac{1}{2}\right)^2\ge0\)
\(\Rightarrow A=\left(x-\dfrac{1}{2}\right)^2-\dfrac{1}{4}\ge-\dfrac{1}{4}\)
\(\Rightarrow Min\left(A\right)=-\dfrac{1}{4}\)