Bài 1 : \(a)\) Chứng minh : \(cos15^o=\dfrac{\sqrt{6}+\sqrt{2}}{4}\)
\(b)\) Trục căn thức của biểu thức : \(A=\dfrac{1}{2+\sqrt[3]{2}+\sqrt[3]{32.cos^415^o-10-8\sqrt{3}}}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b) bạn trục mẫu đi nha dựa vào hằng đẳng thức a^2 -b^2=(a-b)(a+b)
rồi bạn tính nói chung mẫu bằng -1
tính cái trên tử kết quả là 4
c) bạn dựa vào câu b .\(\dfrac{1}{\sqrt{3}}=\dfrac{2}{2\sqrt{3}}>\dfrac{2}{\sqrt{3}+\sqrt{4}}\)
từ đó suy ra B > 2A vậy B>8

+ Ta có:
2√6−√5=2(√6+√5)(√6−√5)(√6+√5)26−5=2(6+5)(6−5)(6+5)
=2(√6+√5)(√6)2−(√5)2=2(√6+√5)6−5=2(6+5)(6)2−(5)2=2(6+5)6−5
=2(√6+√5)1=2(√6+√5)=2(6+5)1=2(6+5).
+ Ta có:
3√10+√7=3(√10−√7)(√10+√7)(√10−√7)310+7=3(10−7)(10+7)(10−7)
=3(√10−√7)(√10)2−(√7)2=3(10−7)(10)2−(7)2=3(√10−√7)10−7=3(10−7)10−7
=3(√10−√7)3=√10−√7=3(10−7)3=10−7.
+ Ta có:
1√x−√y=1.(√x+√y)(√x−√y)(√x+√y)1x−y=1.(x+y)(x−y)(x+y)
=√x+√y(√x)2−(√y)2=√x+√yx−y=x+y(x)2−(y)2=x+yx−y
+ Ta có:
2ab√a−√b=2ab(√a+√b)(√a−√b)(√a+√b)2aba−b=2ab(a+b)(a−b)(a+b)
=2ab(√a+√b)(√a)2−(√b)2=2ab(√a+√b)a−b=2ab(a+b)(a)2−(b)2=2ab(a+b)a−b.
\(\frac{2}{\sqrt{6}-\sqrt{5}}=\frac{2\left(\sqrt{6}+\sqrt{5}\right)}{\left(\sqrt{6}-\sqrt{5}\right)\left(\sqrt{6}+\sqrt{5}\right)}=\frac{2\left(\sqrt{6}+\sqrt{5}\right)}{6-5}=2\left(\sqrt{6}+\sqrt{5}\right)\)
\(\frac{3}{\sqrt{10}+\sqrt{7}}=\frac{3\left(\sqrt{10}-\sqrt{7}\right)}{\left(\sqrt{10}-\sqrt{7}\right)\left(\sqrt{10}+\sqrt{7}\right)}=\frac{3\left(\sqrt{10}-\sqrt{7}\right)}{10-7}=\sqrt{10}-\sqrt{7}\)
\(\frac{1}{\sqrt{x}-\sqrt{y}}=\frac{\sqrt{x}+\sqrt{y}}{x-y}\)
\(\frac{2ab}{\sqrt{a}-\sqrt{b}}=\frac{2ab\left(\sqrt{a}+\sqrt{b}\right)}{a-b}\)

\(\dfrac{2ab}{\sqrt{a}-\sqrt{b}}=\dfrac{2ab\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}=\dfrac{2ab\left(\sqrt{a}+\sqrt{b}\right)}{a-b}\)
\(\dfrac{1}{\sqrt{x}-\sqrt{y}}=\dfrac{\sqrt{x}+\sqrt{y}}{\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{x}+\sqrt{y}\right)}=\dfrac{\sqrt{x}+\sqrt{y}}{x-y}\)
\(\dfrac{3}{\sqrt{10}+\sqrt{7}}=\dfrac{3\left(\sqrt{10}-\sqrt{7}\right)}{\left(\sqrt{10}+\sqrt{7}\right)\left(\sqrt{10}-\sqrt{7}\right)}=\dfrac{3\left(\sqrt{10}-\sqrt{7}\right)}{10-7}=\dfrac{3\left(\sqrt{10}-\sqrt{7}\right)}{3}=\sqrt{10}-\sqrt{7}\)
\(\dfrac{2}{\sqrt{6}-\sqrt{5}}=\dfrac{2\left(\sqrt{6}+\sqrt{5}\right)}{\left(\sqrt{6}-\sqrt{5}\right)\left(\sqrt{6}+\sqrt{5}\right)}=\dfrac{2\left(\sqrt{6}+\sqrt{5}\right)}{6-5}=2\left(\sqrt{6}+\sqrt{5}\right)\)

Bài 1:
a.
\(\frac{1}{2\sqrt{2}-3\sqrt{3}}=\frac{2\sqrt{2}+3\sqrt{3}}{(2\sqrt{2}-3\sqrt{3})(2\sqrt{2}+3\sqrt{3})}=\frac{2\sqrt{2}+3\sqrt{3}}{(2\sqrt{2})^2-(3\sqrt{3})^2}=\frac{2\sqrt{2}+3\sqrt{3}}{-19}\)
b.
\(=\sqrt{\frac{(3-\sqrt{5})^2}{(3-\sqrt{5})(3+\sqrt{5})}}=\sqrt{\frac{(3-\sqrt{5})^2}{3^2-5}}=\sqrt{\frac{(3-\sqrt{5})^2}{4}}=\sqrt{(\frac{3-\sqrt{5}}{2})^2}=|\frac{3-\sqrt{5}}{2}|=\frac{3-\sqrt{5}}{2}\)
Bài 2.
a.
\(=\frac{\sqrt{8}(\sqrt{5}+\sqrt{3})}{(\sqrt{5}-\sqrt{3})(\sqrt{5}+\sqrt{3})}=\frac{2\sqrt{2}(\sqrt{5}+\sqrt{3})}{5-3}=\sqrt{2}(\sqrt{5}+\sqrt{3})=\sqrt{10}+\sqrt{6}\)
b.
\(=\sqrt{\frac{(2-\sqrt{3})^2}{(2-\sqrt{3})(2+\sqrt{3})}}=\sqrt{\frac{(2-\sqrt{3})^2}{2^2-3}}=\sqrt{(2-\sqrt{3})^2}=|2-\sqrt{3}|=2-\sqrt{3}\)

1:
\(A=\sqrt{x^2+\dfrac{2x^2}{3}}=\sqrt{\dfrac{5x^2}{3}}=\left|\sqrt{\dfrac{5}{3}}x\right|=-x\sqrt{\dfrac{5}{3}}\)
2: \(=\left(\dfrac{\sqrt{100}+\sqrt{40}}{\sqrt{5}+\sqrt{2}}+\sqrt{6}\right)\cdot\dfrac{2\sqrt{5}-\sqrt{6}}{2}\)
\(=\dfrac{\left(2\sqrt{5}+\sqrt{6}\right)\left(2\sqrt{5}-\sqrt{6}\right)}{2}\)
\(=\dfrac{20-6}{2}=7\)

Bài 1 :
a, ĐKXĐ : \(\dfrac{1}{2-x}\ge0\)
Mà 1 > 0
\(\Rightarrow2-x>0\)
\(\Rightarrow x< 2\)
Vậy ...
b, Ta có : \(\sqrt[3]{125}.\sqrt[3]{216}-\sqrt[3]{512}.\sqrt[3]{\dfrac{1}{8}}\)
\(=5.6-\dfrac{8.1}{2}=26\)
1a) Để căn thức bậc 2 có nghĩa thì \(\dfrac{1}{2-x}\ge0\Rightarrow2-x>0\Rightarrow x< 2\)
b) \(\sqrt[3]{125}.\sqrt[3]{-216}-\sqrt[3]{512}.\sqrt[3]{\dfrac{1}{8}}=\sqrt[3]{5^3}.\sqrt[3]{\left(-6\right)^3}-\sqrt[3]{8^3}.\sqrt[3]{\left(\dfrac{1}{2}\right)^3}\)
\(=5.\left(-6\right)-8.\dfrac{1}{2}=-34\)
\(\dfrac{\sqrt{ab}-b}{b}-\sqrt{\dfrac{a}{b}}=\dfrac{\sqrt{b}\left(\sqrt{a}-\sqrt{b}\right)}{\left(\sqrt{b}\right)^2}-\dfrac{\sqrt{a}}{\sqrt{b}}=\dfrac{\sqrt{a}-\sqrt{b}}{\sqrt{b}}-\dfrac{\sqrt{a}}{\sqrt{b}}\)
\(=-\dfrac{\sqrt{b}}{\sqrt{b}}=-1< 0\)
Bài 1 :
a) \(Cos30^o=Cos\left(2.15^o\right)=2cos^215^o-1\)
\(\Rightarrow cos^215^o=\dfrac{cos30^o+1}{2}\)
\(\Rightarrow cos^215^o=\dfrac{\dfrac{\sqrt[]{3}}{2}+1}{2}\)
\(\Rightarrow cos^215^o=\dfrac{\sqrt[]{3}+2}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt[]{\sqrt[]{3}+2}}{2}\)
\(\Rightarrow cos15^o=\dfrac{2\sqrt[]{\sqrt[]{3}+2}}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt[]{4\sqrt[]{3}+8}}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt[]{6+2.2\sqrt[]{2}\sqrt[]{6}+2}}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt[]{\left(\sqrt[]{6}+\sqrt[]{2}\right)^2}}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt[]{6}+\sqrt[]{2}^{ }}{4}\left(dpcm\right)\)
a)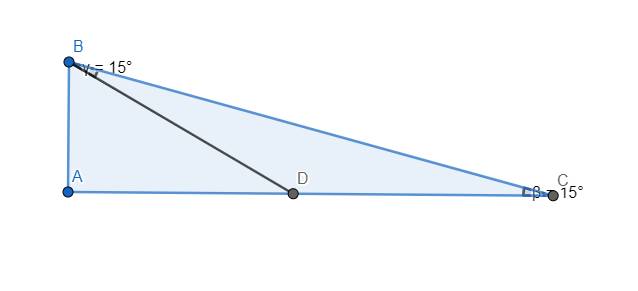
Dựng tam giác ABC vuông tại A với \(\widehat{C}=15^o\). Trên đoạn thẳng AC lấy điểm D sao cho \(\widehat{CBD}=15^o\). Không mất tính tổng quát, ta chuẩn hóa \(AB=1\). \(\Rightarrow\left\{{}\begin{matrix}BD=\dfrac{AB}{cos60^o}=2\\AD=AB.tan60^o=\sqrt{3}\end{matrix}\right.\)
Dễ thấy tam giác DBC cân tại D \(\Rightarrow BD=CD=2\) \(\Rightarrow AC=AD+DC=2+\sqrt{3}\)
\(\Rightarrow tanC=\dfrac{AB}{AC}=\dfrac{1}{2+\sqrt{3}}=2-\sqrt{3}\)
\(\Rightarrow\dfrac{sinC}{cosC}=2-\sqrt{3}\)
\(\Rightarrow sinC=\left(2-\sqrt{3}\right)cosC\)
Mà \(sin^2C+cos^2C=1\)
\(\Rightarrow\left(7-4\sqrt{3}\right)cos^2C+cos^2C=1\)
\(\Leftrightarrow\left(8-4\sqrt{3}\right)cos^2C=1\)
\(\Leftrightarrow cos^2C=\dfrac{1}{8-4\sqrt{3}}=\dfrac{2+\sqrt{3}}{4}\)
\(\Leftrightarrow cosC=\sqrt{\dfrac{2+\sqrt{3}}{4}}\) \(=\dfrac{\sqrt{2+\sqrt{3}}}{2}=\dfrac{\sqrt{8+4\sqrt{3}}}{4}\) \(=\dfrac{\sqrt{6}+\sqrt{2}}{4}\)
\(\Rightarrow cos15^o=\dfrac{\sqrt{6}+\sqrt{2}}{4}\)