
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\Rightarrow24-x=17-125=-108\\ \Rightarrow x=24-\left(-108\right)=24+108=132\)
\(125+\left(24-x\right)=17\)
\(\Rightarrow24-x=17-125\)
\(\Rightarrow24-x=-108\)
\(\Rightarrow-x=-108-24\)
\(\Rightarrow-x=-132\)
\(\Rightarrow x=132\)

\(26⋮\left(x-1\right);38⋮\left(x-1\right)\Rightarrow x-1\inƯC\left(26,38\right)=\left\{\pm2;\pm1\right\}\Rightarrow x\in\left\{-1;0;2;3\right\}\)
Mà x là số tự nhiên \(\Rightarrow x\in\left\{0;2;3\right\}\)
d) 26 = 2 . 13
38 = 2 . 19
ƯCLN(26,38) = 2
ƯC(26,38) = Ư(2) = 1,2
Mà theo đề bài : 26 ⋮ (x-1) ; 38 ⋮ (x-1)
Nên : x = 2 hoặc 3

a: \(2\left(x-51\right)=2\cdot2^3+20\)
=>\(2\left(x-51\right)=2^4+20=36\)
=>x-51=36/2=18
=>x=18+51=69
b: \(2x-49=5\cdot3^2\)
=>\(2x-49=5\cdot9=45\)
=>2x=45+49=94
=>x=94/2=47
c: \(\left[\left(8x-12\right):4\right]\cdot3^3=3^6\)
=>\(\left[4\cdot\dfrac{\left(2x-3\right)}{4}\right]=3^3\)
=>\(2x-3=3^3=27\)
=>2x=3+27=30
=>x=30/2=15
d: \(2^{x+1}-2^2=32\)
=>\(2^{x+1}=32+2^2=32+4=36\)
=>\(x+1=log_236\)
=>\(x=log_236-1\)
e: \(\left(x^3-77\right):4=5\)
=>\(x^3-77=20\)
=>\(x^3=77+20=97\)
=>\(x=\sqrt[3]{97}\)

a, 36:(x–5) = 2 2
(x–5) = 9
x = 14
b, [3.(70–x)+5]:2 = 46
[3.(70–x)+5] = 92
70–x = 29
x = 41
c, 450:[41–(2x–5)] = 3 2 .5
41–(2x–5) = 10
2x–5 = 31
2x = 36
x = 18
d, 230+[ 2 4 +(x–5)] = 315. 2018 0
16+(x–5) = 315–230
x–5 = 85–16
x = 69+5
x = 74
e, 2 x + 2 x + 1 = 48
2 x .(2+1) = 48
2 x = 16 = 2 4
x = 4
f, 3 x + 2 + 3 x = 2430
3 x . 3 2 + 1 = 2430
3 x = 2430:10 = 243 = 3 5
x = 5

a) \(xy+x+2y=5\Leftrightarrow xy+x+2y+2=7\Leftrightarrow\left(y+1\right)\left(x+2\right)=7\)
Vì x,y là số tự nhiên nên \(x,y\in N\)\(x,y\ge0\)\(\Rightarrow y+1\ge1;x+2\ge2\)
Từ đó ta có :
\(\hept{\begin{cases}x+2=7\\y+1=1\end{cases}\Leftrightarrow\hept{\begin{cases}x=5\\y=0\end{cases}}}\)
b) \(xy+2x+2y=-16\Leftrightarrow xy+2y+2x+4=-12\Leftrightarrow\left(y+2\right)\left(x+2\right)=-12\)
Lần lượt xét từng trường hợp , ta được :
(x;y) = (-14; -1) ; (-8 ; 0) ; (-6 ; 1) ; (-5 ;2) ; (-4 ;4)
a) \(\left(x+2\right)\left(y+1\right)=7=1.7=7.1\)
Hoặc \(\hept{\begin{cases}x+2=7\\y+1=1\end{cases}\Leftrightarrow\hept{\begin{cases}x=5\\y=0\end{cases}}}\in N\)
Hoặc\(\hept{\begin{cases}x+2=1\\y+1=7\end{cases}}\Leftrightarrow\hept{\begin{cases}x=-1\notin N\\y=6\end{cases}}\)
Vậy \(\left(x;y\right)=\left(5;0\right)\)
b)\(\left(x+2\right)\left(y+2\right)=-1.12=-12.1=-2.6=-6.2=-3.4=-4.3\)
tương tự giải 6 TH là được

a) \(\Leftrightarrow2x+5=3^6\\ \Leftrightarrow2x+5=729\\ \Leftrightarrow x=362\)
b) \(\Leftrightarrow x+55=60\\ \Leftrightarrow x=5\)
c) \(x=\left\{12;24;36;48\right\}\)

Bài 10:
a: 2x-3 là bội của x+1
=>\(2x-3⋮x+1\)
=>\(2x+2-5⋮x+1\)
=>\(-5⋮x+1\)
=>\(x+1\in\left\{1;-1;5;-5\right\}\)
=>\(x\in\left\{0;-2;4;-6\right\}\)
b: x-2 là ước của 3x-2
=>\(3x-2⋮x-2\)
=>\(3x-6+4⋮x-2\)
=>\(4⋮x-2\)
=>\(x-2\inƯ\left(4\right)\)
=>\(x-2\in\left\{1;-1;2;-2;4;-4\right\}\)
=>\(x\in\left\{3;1;4;0;6;-2\right\}\)
Bài 14:
a: \(4n-5⋮2n-1\)
=>\(4n-2-3⋮2n-1\)
=>\(-3⋮2n-1\)
=>\(2n-1\inƯ\left(-3\right)\)
=>\(2n-1\in\left\{1;-1;3;-3\right\}\)
=>\(2n\in\left\{2;0;4;-2\right\}\)
=>\(n\in\left\{1;0;2;-1\right\}\)
mà n>=0
nên \(n\in\left\{1;0;2\right\}\)
b: \(n^2+3n+1⋮n+1\)
=>\(n^2+n+2n+2-1⋮n+1\)
=>\(n\left(n+1\right)+2\left(n+1\right)-1⋮n+1\)
=>\(-1⋮n+1\)
=>\(n+1\in\left\{1;-1\right\}\)
=>\(n\in\left\{0;-2\right\}\)
mà n là số tự nhiên
nên n=0
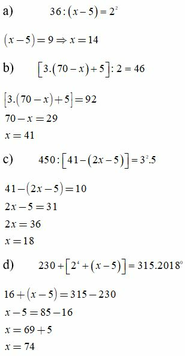
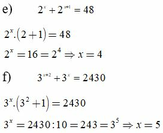
d)22.2x=16
⇔4.2x=16
⇔2x=4
⇔2x=22
⇔x=2
2x=24:22
2x=22
=> x=2