
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(1,\Leftrightarrow\left\{{}\begin{matrix}x=2y+4\\-4y-8+5y=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\cdot5+4=14\\y=5\end{matrix}\right.\\ 2,\Leftrightarrow\left\{{}\begin{matrix}5x-30+6x=3\\y=10-2x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=4\end{matrix}\right.\\ 3,\Leftrightarrow\left\{{}\begin{matrix}x=4-2y\\6y-12+y=7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{10}{7}\\y=\dfrac{19}{7}\end{matrix}\right.\)

\(a,\Leftrightarrow\left\{{}\begin{matrix}5x+15y=-10\\5x-4y=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}19y=-21\\5x-4y=11\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=-\dfrac{21}{19}\\5x-4\left(-\dfrac{21}{19}\right)=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{25}{19}\\y=-\dfrac{21}{19}\end{matrix}\right.\)
\(c,\Leftrightarrow\left\{{}\begin{matrix}3x+5y=1\\10x-5y=-40\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+5y=1\\13x=-39\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-3\\y=2\end{matrix}\right.\\ d,\Leftrightarrow\left\{{}\begin{matrix}5x-10y=-30\\5x-3y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5x-3y=5\\-7y=-35\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=5\end{matrix}\right.\\ e,\Leftrightarrow\left\{{}\begin{matrix}2\left(x+y\right)+3\left(x-y\right)=4\\2\left(x+y\right)+4\left(x-y\right)=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-y=6\\2\left(x+y\right)+3\cdot6=4\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x-y=6\\x+y=-7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{1}{2}\\y=-\dfrac{13}{2}\end{matrix}\right.\)

a: \(\left\{{}\begin{matrix}x+2y=3\\4x+5y=6\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4x+8y=12\\4x+5y=6\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3y=6\\x+2y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=2\\x=3-2y=3-2\cdot2=-1\end{matrix}\right.\)
b: \(\left\{{}\begin{matrix}x+y=5\\2x-y=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+y+2x-y=5+4\\x+y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3x=9\\x+y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=5-3=2\end{matrix}\right.\)
c: \(\left\{{}\begin{matrix}x+2y=5\\x-5y=-9\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+2y-x+5y=5+9=14\\x+2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7y=14\\x+2y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=2\\x=5-2y=1\end{matrix}\right.\)

\(HPT\Leftrightarrow\left\{{}\begin{matrix}2x-3y=2-m\\2x+4y=6m+2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+2y=3m+1\\7y=7m\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x+2m=3m+1\\y=m\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=m+1\\y=m\end{matrix}\right.\\ x^2+y^2=10\Leftrightarrow m^2+2m+1+m^2=10\\ \Leftrightarrow2m^2+2m-9=0\\ \Delta=4+72=76\\ \Leftrightarrow\left[{}\begin{matrix}m=\dfrac{-2-2\sqrt{19}}{4}=\dfrac{-1-\sqrt{19}}{2}\\m=\dfrac{-2+2\sqrt{19}}{4}=\dfrac{-1+\sqrt{19}}{2}\end{matrix}\right.\)

Bài 2:
a) Ta có: \(\Delta=\left(m-1\right)^2-4\cdot1\cdot\left(-m^2-2\right)\)
\(=m^2-2m+1+4m^2+8\)
\(=5m^2-2m+9>0\forall m\)
Do đó, phương trình luôn có hai nghiệm phân biệt với mọi m
Bài 1:
ĐKXĐ \(2x\ne y\)
Đặt \(\dfrac{1}{2x-y}=a;x+3y=b\)
HPT trở thành
\(\left\{{}\begin{matrix}a+b=\dfrac{3}{2}\\4a-5b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{3}{2}-b\\4\left(\dfrac{3}{2}-b\right)-5b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{3}{2}-b\\6-9b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}b=\dfrac{8}{9}\\a=\dfrac{11}{18}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+3y=\dfrac{8}{9}\\2x-y=\dfrac{18}{11}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=2x-\dfrac{18}{11}\\x+3\left(2x-\dfrac{18}{11}\right)=\dfrac{8}{9}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{82}{99}\\y=\dfrac{2}{99}\end{matrix}\right.\)

h) \(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=2\\\dfrac{3}{x}-\dfrac{4}{y}=-1\end{matrix}\right.\)\(\left(1\right)\)\(\left(đk:x,y\ne0\right)\)
Đặt \(a=\dfrac{1}{x},b=\dfrac{1}{y}\)
\(\left(1\right)\Leftrightarrow\) \(\left\{{}\begin{matrix}a+b=2\\3a-4b=-1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}3a+3b=6\\3a-4b=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=2\\7b=7\end{matrix}\right.\)\(\Leftrightarrow a=b=1\)
Thay a,b:
\(\Leftrightarrow\dfrac{1}{x}=\dfrac{1}{y}=1\Leftrightarrow x=y=1\left(tm\right)\)
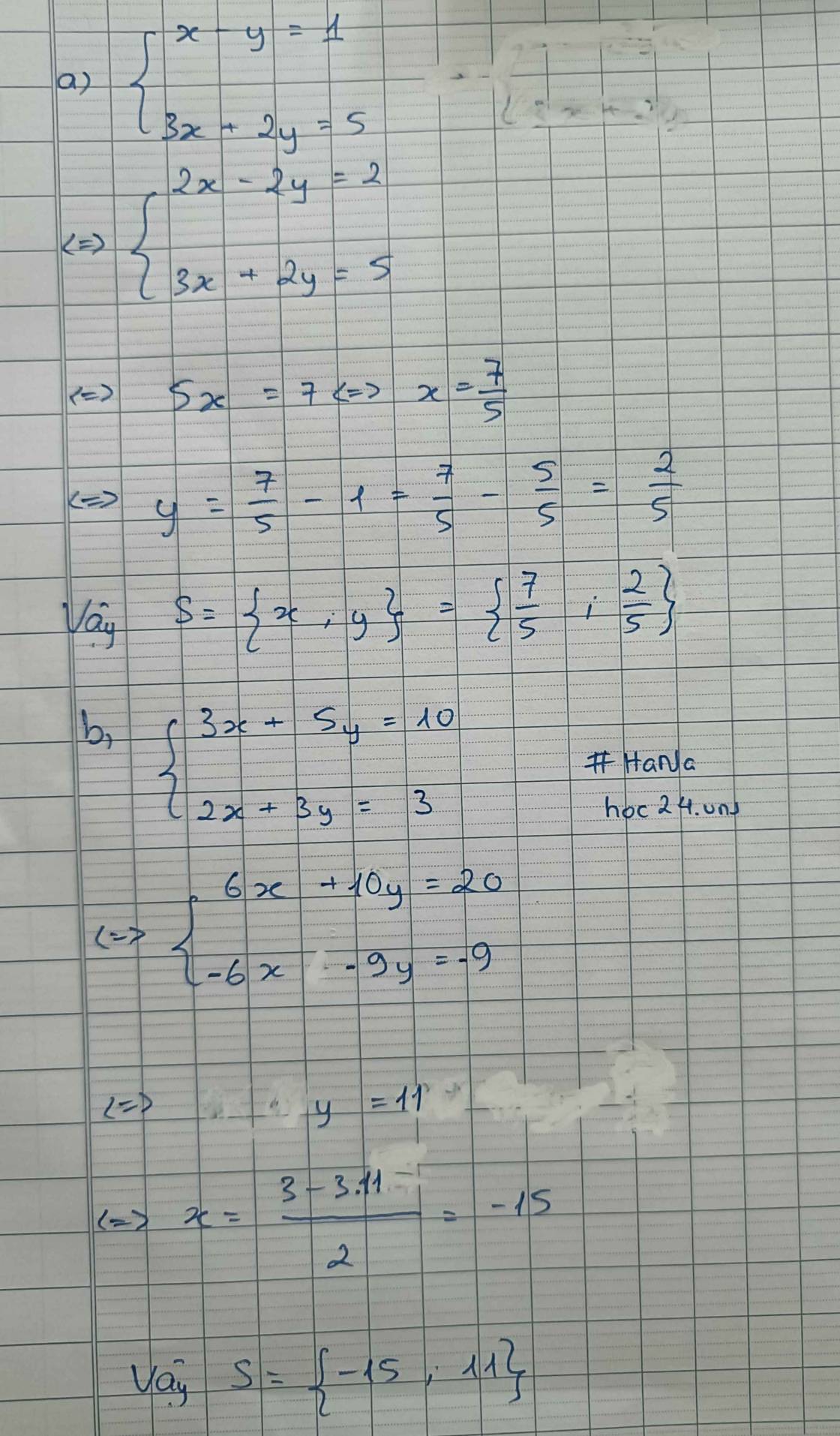
\(\left\{{}\begin{matrix}2x+5y=-\left(x+y\right)\left(1\right)\\6x+3y=y-10\left(2\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x+5y=-x-y\\6x+2y=-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x+6y=0\\6x+2y=-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x+6y=0\\3x+y=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}5y=5\\3x+y=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=-2y\\y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=-2\\y=1\end{matrix}\right.\)