b: B>0

=>\(\dfrac{1}{-x+\sqrt{x}}>0\)

=>\(-x+\sqrt{x}>0\)

=>\(x-\sqrt{x}< 0\)

=>\(\sqrt{x}\left(\sqrt{x}-1\right)< 0\)

=>\(\sqrt{x}-1< 0\)

=>\(\sqrt{x}< 1\)

=>0<=x<1

Kết hợp ĐKXĐ, ta được: 0<x<1

trong \(\Delta ABC\) vuông tại A

AB=AC.tanC=10.tan30=5,77

CB=\(\sqrt{AC^2+AB^2}=\sqrt{10^2+5,77^2}=11,55\)

\(AH.BC=AB.AC\Rightarrow AH=\dfrac{AB.AC}{BC}=\dfrac{5,77.10}{11,55}=5\)

\(\widehat{B}=90-\widehat{C}=90-30=60\)

1.

Ta có: $4x^2+4x+3=(4x^2+4x+1)+2=(2x+1)^2+2\geq 0+2=2$

$\Rightarrow A=\frac{6}{4x^2+4x+3}\leq \frac{6}{2}=3$

Vậy $A_{\max}=3$. Giá trị này đạt tại $2x+1=0\Leftrightarrow x=\frac{-1}{2}$

2.

$6+4x+x^2=(x^2+4x+4)+2=(x+2)^2+2\geq 0+2=2$

$\Rightarrow \frac{4}{6+4x+x^2}\leq \frac{4}{2}=2$

$\Rightarrow \frac{-4}{6+4x+x^2}\geq -2$

$\Rightarrow B\geq -2$

Vậy $B_{\min}=-2$. Giá trị này đạt tại $x+2=0\Leftrightarrow x=-2$

\(\sqrt{9x+9}-2\sqrt{\dfrac{x+1}{4}}=4\left(đk:x\ge-1\right)\)

\(\Leftrightarrow3\sqrt{x+1}-\sqrt{x+1}=4\)

\(\Leftrightarrow2\sqrt{x+1}=4\)

\(\Leftrightarrow\sqrt{x+1}=2\Leftrightarrow x+1=4\Leftrightarrow x=3\left(tm\right)\)

Bài 2:

e) \(\sqrt{4x-8}-12\sqrt{\dfrac{x-2}{9}}=\sqrt{x-2}-12\left(đk:x\ge2\right)\)

\(\Leftrightarrow\sqrt{4}.\sqrt{x-2}-12.\sqrt{\dfrac{1}{9}}.\sqrt{x-2}=\sqrt{x-2}-12\)

\(\Leftrightarrow2\sqrt{x-2}-4\sqrt{x-2}=\sqrt{x-2}-12\)

\(\Leftrightarrow3\sqrt{x-2}=12\)

\(\Leftrightarrow\sqrt{x-2}=4\)

\(\Leftrightarrow x-2=16\Leftrightarrow x=18\left(tm\right)\)

Cảm ơn bạn

Câu 1:

\(P=\dfrac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}+\dfrac{\left(2-\sqrt{a}\right)\left(2+\sqrt{a}\right)}{2-\sqrt{a}}=\sqrt{a}+2+\sqrt{a}+2=2\sqrt{a}+4\\ A=\dfrac{x+3\sqrt{x}+2+2x-4\sqrt{x}-2-5\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\\ A=\dfrac{3\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{3\sqrt{x}}{\sqrt{x}+2}\\ C=\dfrac{\sqrt{x}-1+\sqrt{x}+1-4\sqrt{x}+2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{-2\sqrt{x}-2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\\ C=\dfrac{-2\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2}{1-\sqrt{x}}\)

\(D=\dfrac{\sqrt{x}-3+\sqrt{x}+3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\cdot\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}{2}=\dfrac{2\sqrt{x}}{2}=\sqrt{x}\\ P=\dfrac{8\sqrt{x}-4x+8x}{\left(2+\sqrt{x}\right)\left(2-\sqrt{x}\right)}:\dfrac{\sqrt{x}-2-2\sqrt{x}+4}{\sqrt{x}\left(\sqrt{x}-2\right)}\\ P=\dfrac{4\sqrt{x}\left(2+\sqrt{x}\right)}{\left(2+\sqrt{x}\right)\left(2-\sqrt{x}\right)}\cdot\dfrac{\sqrt{x}\left(2-\sqrt{x}\right)}{\sqrt{x}-2}=\dfrac{4x}{\sqrt{x}-2}\\ Q=\dfrac{\left(\sqrt{a}+4\right)^2}{\sqrt{a}+4}+\dfrac{\left(3-\sqrt{a}\right)\left(3+\sqrt{a}\right)}{3-\sqrt{a}}-\dfrac{\sqrt{a}\left(\sqrt{a}-2\right)}{\sqrt{a}}\\ Q=\sqrt{a}+4+3+\sqrt{a}-\sqrt{a}+2\\ Q=\sqrt{a}+9\)

a/

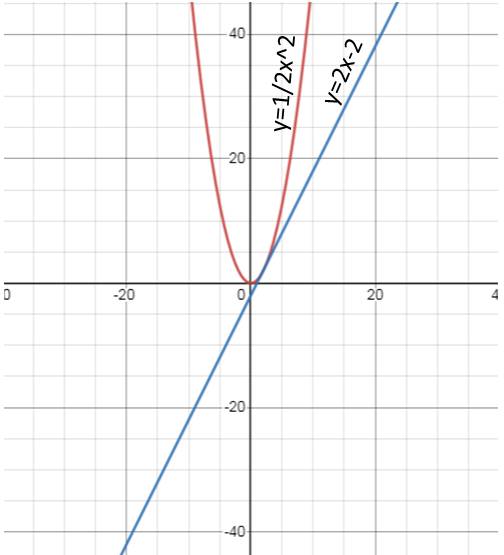
b/ 

Tọa độ giao điểm của 2 đồ thị là:

\(\dfrac{1}{2}x^2=2x-2\\ \Leftrightarrow\dfrac{1}{2}x^2-2x+2=0\\ \Leftrightarrow x=2\)

b) Phương trình hoành độ giao điểm của (P) và (d):

1/2 x² = 2x - 2

⇔x² = 4x - 4

⇔x² - 4x + 4 = 0

⇔(x - 2)² = 0

⇔x - 2 = 0

⇔x = 2

⇔y = 2.2 - 2 = 2

Vậy tọa độ giao điểm của (P) và (d) là (2;2)

08:43 :vvvv

BTVN :))