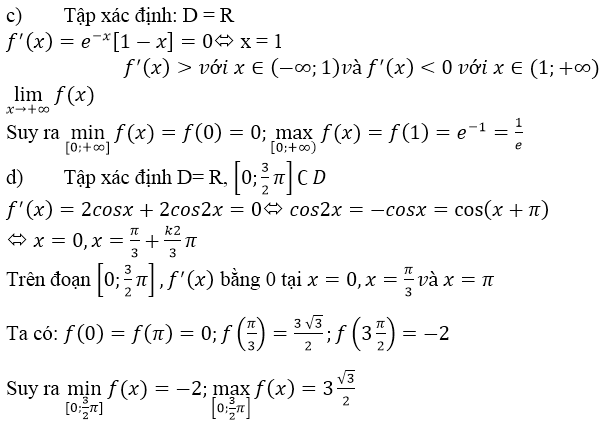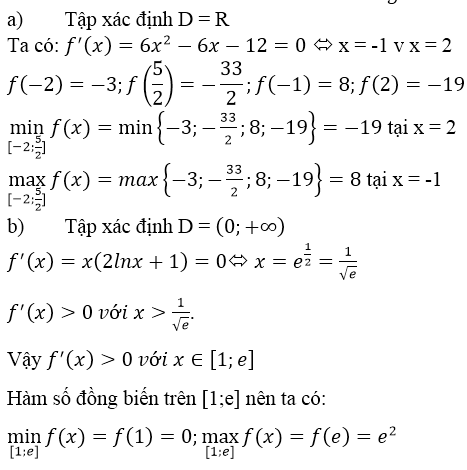Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) f(x) = 2x3 – 3x2 – 12x + 1 ⇒ f’(x) = 6x2 – 6x – 12
f’(x) = 0 ⇔ x ∈ {-1, 2}
So sánh các giá trị:
f(x) = -3; f(-1) = 8;
f(2) = -19, f(52)=−332f(52)=−332
Suy ra:
maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19
b) f(x) = x2 lnx ⇒ f’(x)= 2xlnx + x > 0, ∀ x ∈ [1, e] nên f(x) đồng biến.
Do đó:
maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0
c) f(x) = f(x) = xe-x ⇒ f’(x)= e-x – xe-x = (1 – x)e-x nên:
f’(x) = 0 ⇔ x = 1, f’(x) > 0, ∀x ∈ (0, 1) và f’(x) < 0, ∀x ∈ (1, +∞)
nên:
maxx∈[0,+∞)f(x)=f(1)=1emaxx∈[0,+∞)f(x)=f(1)=1e
Ngoài ra f(x) = xe-x > 0, ∀ x ∈ (0, +∞) và f(0) = 0 suy ra
maxx∈[0,+∞)f(x)=f(0)=0maxx∈[0,+∞)f(x)=f(0)=0
d) f(x) = 2sinx + sin2x ⇒ f’(x)= 2cosx + 2cos2x
f’(x) = 0 ⇔ cos 2x = -cosx ⇔ 2x = ± (π – x) + k2π
⇔ x∈{−π+k2π;π3+k2π3}x∈{−π+k2π;π3+k2π3}
Trong khoảng [0,3π2][0,3π2] , phương trình f’(x) = 0 chỉ có hai nghiệm là x1=π3;x2=πx1=π3;x2=π
So sánh bốn giá trị : f(0) = 0; f(π3)=3√32;f(π)=0;f(3π2)=−2f(π3)=332;f(π)=0;f(3π2)=−2
Suy ra:
maxx∈[0,3π2]f(x)=f(π3)=3√32minx∈[0,3π2]f(x)=f(3π2)=−2

Cho hàm số y=f(x)y=f(x) có đạo hàm và liên tục trên [0;π2][0;π2]thoả mãn f(x)=f′(x)−2cosxf(x)=f′(x)−2cosx. Biết f(π2)=1f(π2)=1, tính giá trị f(π3)f(π3)
A. √3+1/2 B. √3−1/2 C. 1−√3/2 D. 0

1. \(f\left(x\right)=e^{x^3-3x+3}\) trên đoạn \(\left[0;2\right]\)
Ta có : \(f'\left(x\right)=\left(3x^2-3\right)e^{x^3-3x+3}=0\Leftrightarrow3x^2-3=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=-1\notin\left[0;2\right]\\x=1\in\left[0;2\right]\end{array}\right.\)
mà : \(\begin{cases}f\left(0\right)=e^3\\f\left(1\right)=e\\f\left(2\right)=e^5\end{cases}\) \(\Rightarrow\begin{cases}Max_{x\in\left[0;2\right]}f\left(x\right)=e^5;x=1\\Min_{x\in\left[0;2\right]}f\left(x\right)=e;x=2\end{cases}\)
2. \(f\left(x\right)=\ln\left(x^2-x+1\right)\) trên đoạn \(\left[1;3\right]\)
Mà \(\begin{cases}f\left(1\right)=0\\f\left(3\right)=\ln7\end{cases}\) \(\Leftrightarrow\begin{cases}Max_{x\in\left[1;3\right]}f\left(x\right)=\ln7;x=3\\Min_{x\in\left[1;3\right]}f\left(x\right)=0;x=1\end{cases}\)

f'(x)>0 với mọi x khác -8, suy ra hàm số đã cho đồng biến trên [0;3].
Giá trị nhỏ nhất của f(x) trên [0;3] là (-m^2)/8. Ta có: (-m^2)/8=2.
Suy ra, không có giá trị nào của số thực m thỏa yêu cầu đề bài.

1. \(f\left(x\right)=e^{2-3x}\) trên đoạn \(\left[0;2\right]\)
Ta có :
\(f'\left(x\right)=-3e^{2-3x}< 0\) với \(x\in R\Rightarrow\) hàm số nghịch biến trên đoạn \(\left[0;2\right]\)
Với \(0\le x\le2\Leftrightarrow f\left(0\right)\ge f\left(x\right)\ge f\left(2\right)\Leftrightarrow e^2\ge f\left(x\right)\ge\frac{1}{e^4}\)
\(\Leftrightarrow\begin{cases}Max_{x\in\left[0;2\right]}f\left(x\right)=e^2;x=0\\Min_{x\in\left[0;2\right]}f\left(x\right)=\frac{1}{e^4};x=2\end{cases}\)
2. \(f\left(x\right)=e^{\sqrt{1-x^2}}\) trên đoạn \(\left[-1;1\right]\)
Ta có :
\(f'\left(x\right)=\frac{-x}{\sqrt{1-x^2}}e^{\sqrt{1-x^2}}=0\Leftrightarrow x=0\in\left[-1;1\right]\)
Mà : \(\begin{cases}f\left(-1\right)=1\\f\left(0\right)=e\\f\left(1\right)=1\end{cases}\) \(\Leftrightarrow\begin{cases}Max_{x\in\left[-1;1\right]}f\left(x\right)=e;x=0\\Min_{x\in\left[-1;1\right]}f\left(x\right)=1;x=\pm1\end{cases}\)

\(2x.f'\left(x\right)-f\left(x\right)=x^2\sqrt{x}.cosx\)
\(\Leftrightarrow\dfrac{1}{\sqrt{x}}.f'\left(x\right)-\dfrac{1}{2x\sqrt{x}}f\left(x\right)=x.cosx\)
\(\Leftrightarrow\left[\dfrac{f\left(x\right)}{\sqrt{x}}\right]'=x.cosx\)
Lấy nguyên hàm 2 vế:
\(\int\left[\dfrac{f\left(x\right)}{\sqrt{x}}\right]'dx=\int x.cosxdx\)
\(\Rightarrow\dfrac{f\left(x\right)}{\sqrt{x}}=x.sinx+cosx+C\)
\(\Rightarrow f\left(x\right)=x\sqrt{x}.sinx+\sqrt{x}.cosx+C.\sqrt{x}\)
Thay \(x=4\pi\)
\(\Rightarrow0=4\pi.\sqrt{4\pi}.sin\left(4\pi\right)+\sqrt{4\pi}.cos\left(4\pi\right)+C.\sqrt{4\pi}\)
\(\Rightarrow C=-1\)
\(\Rightarrow f\left(x\right)=x\sqrt{x}.sinx+\sqrt{x}.cosx-\sqrt{x}\)