
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


A = \(\dfrac{x^2-2x+2020}{2021x^2}\)
= \(\dfrac{2020x^2-2.2020.x+2020^2}{2021.2020x^2}\)
\(=\dfrac{2019x^2}{2021.2020x^2}+\dfrac{x^2-2.2020.x+2020^2}{2021.2020x^2}\)
= \(\dfrac{2019}{2021.2020}+\dfrac{\left(x-2020\right)^2}{2021.2020x^2}\ge\dfrac{2019}{2021.2020}\)
Dấu "=" xảy ra <=> x - 2020 = 0
<=> x = 2020
Vậy minA = \(\dfrac{2019}{2021.2020}\)đạt được tại x = 2020

\(Q=\dfrac{x^2+xy+y^2+300}{x+y}=\dfrac{\dfrac{1}{2}\left(x+y\right)^2+\dfrac{1}{2}\left(x^2+y^2\right)+300}{x+y}\)
\(Q\ge\dfrac{\dfrac{1}{2}\left(x+y\right)^2+\dfrac{1}{4}\left(x+y\right)^2+300}{x+y}=\dfrac{\dfrac{3}{4}\left(x+y\right)^2+300}{x+y}\)
\(Q\ge\dfrac{2\sqrt{\dfrac{3}{4}\left(x+y\right)^2.300}}{x+y}=30\)
\(Q_{min}=30\) khi \(x=y=10\)
cho em hỏi là
chỗ này \(\dfrac{1}{2}\left(x+y^{ }\right)^{2
}+\dfrac{1}{2}\left(x^2+y^2\right)+300\)
tại sao lại ra như vậy ạ

\(M=6x^2+4y^2+6xy+\left(xy+\dfrac{4x}{y}\right)+\left(3xy+\dfrac{3y}{x}\right)+2022\)
\(M\ge3x^2+y^2+3\left(x+y\right)^2+2\sqrt{\dfrac{4x^2y}{y}}+2\sqrt{\dfrac{9xy^2}{x}}+2022\)
\(M\ge3\left(x^2+1\right)+\left(y^2+4\right)+3\left(x+y\right)^2+4x+6y+2015\)
\(M\ge6x+4y+3\left(x+y\right)^2+4x+6y+2015\)
\(M\ge3\left(x+y\right)^2+10\left(x+y\right)+2015\ge3.3^2+10.3+2015=2072\)
Dấu "=" xảy ra khi \(\left(x;y\right)=\left(1;2\right)\)

a) đK: \(x\ne0;2\)
B = \(\dfrac{3x-4}{x\left(x-2\right)}.\dfrac{x\left(x-2\right)}{x^2-4-x^2}=\dfrac{3x-4}{-4}=\dfrac{4-3x}{4}\) \(\dfrac{x-4+2x}{x\left(x-2\right)}:\dfrac{\left(x-2\right)\left(x+2\right)-x^2}{x\left(x-2\right)}\)
= \(\dfrac{3x-4}{x\left(x-2\right)}.\dfrac{x\left(x-2\right)}{x^2-4-x^2}=\dfrac{4-3x}{4}\)
b) Thay x = -2 (TMDK) vào B, ta có:
\(B=\dfrac{4-3.\left(-2\right)}{4}=\dfrac{4+6}{4}=\dfrac{5}{2}\)
c) Để \(\left|B\right|-2x=5\)
<=> \(\left|\dfrac{4-3x}{4}\right|-2x=5\)
TH1: \(x\le\dfrac{4}{3}\)
<=> \(\left|\dfrac{4-3x}{4}\right|=\dfrac{4-3x}{4}\)
PT <=> \(\dfrac{4-3x}{4}-2x=5\)
<=> \(\dfrac{4-3x-8x}{4}=5\)
<=> \(4-11x=20\)
<=> x = \(\dfrac{-16}{11}\) (Tm)
TH2: \(x>\dfrac{4}{3}\)
<=> \(\left|\dfrac{4-3x}{4}\right|=\dfrac{3x-4}{4}\)
PT <=> \(\dfrac{3x-4}{4}-2x=5\)
<=> \(\dfrac{3x-4-8x}{4}=5\)
<=> \(-5x-4=20\)
<=> \(x=\dfrac{-24}{5}\left(l\right)\)
d) Xét (2-x)B = \(\dfrac{\left(2-x\right)\left(4-3x\right)}{4}\) = \(\dfrac{3x^2-10x+8}{4}\)
= \(\dfrac{3\left(x-\dfrac{5}{3}\right)^2-\dfrac{1}{3}}{4}\)
Mà \(3\left(x-\dfrac{5}{3}\right)^2\ge\) 0
=> (2-x)B \(\ge\dfrac{\dfrac{-1}{3}}{4}=\dfrac{-1}{12}\)
Dấu "=" <=> x = \(\dfrac{5}{3}\left(tm\right)\)
e) Số nguyên âm lớn nhất là -1
Để B = -1
<=> \(\dfrac{4-3x}{4}=-1\)
<=> 4 - 3x = -4
<=> \(x=\dfrac{8}{3}\left(tm\right)\)
g)
TH1: \(x\le\dfrac{4}{3}\)
<=> \(\left|\dfrac{4-3x}{4}\right|=\dfrac{4-3x}{4}\)
BDT <=> \(\dfrac{4-3x}{4}< 2x-4\)
<=> \(4-3x< 8x-16\)
<=> \(x>\dfrac{20}{11}\left(l\right)\)
TH2: \(x>\dfrac{4}{3}\)
<=> \(\left|\dfrac{4-3x}{4}\right|=\dfrac{3x-4}{4}\)
BDT <=> \(\dfrac{3x-4}{4}< 2x-4\)
<=> \(3x-4< 8x-16\)
<=> x > \(\dfrac{12}{5}\)
KHDK: \(x>\dfrac{12}{5}\)

1) Ta có: \(P=\left(\dfrac{1}{\sqrt{x}}+\dfrac{\sqrt{x}}{\sqrt{x}+1}\right):\dfrac{\sqrt{x}}{x+\sqrt{x}}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{\sqrt{x}}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}}\)
Để \(P=\dfrac{7}{2}\) thì \(2x+2\sqrt{x}+2-7\sqrt{x}=0\)
\(\Leftrightarrow2x-4\sqrt{x}-\sqrt{x}+2=0\)
\(\Leftrightarrow2\sqrt{x}\left(\sqrt{x}-2\right)-\left(\sqrt{x}-2\right)=0\)
\(\Leftrightarrow\left(\sqrt{x}-2\right)\left(2\sqrt{x}-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=\dfrac{1}{4}\end{matrix}\right.\)

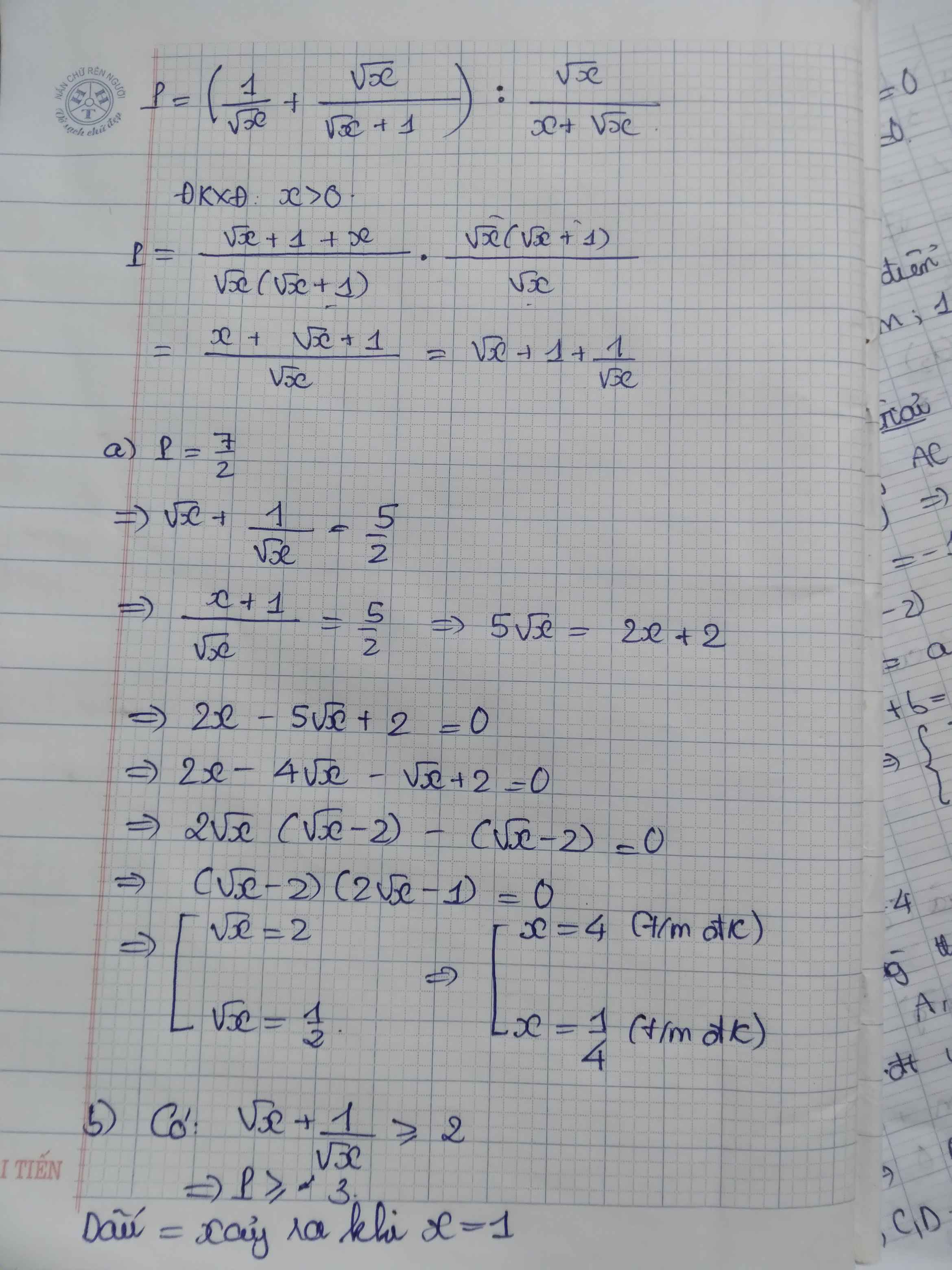
Đặt \(A=\dfrac{x}{x+2}=1-\dfrac{2}{x+2}\)
do \(x\ge0\Leftrightarrow x+2\ge2\Leftrightarrow\dfrac{1}{x+2}\le\dfrac{1}{2}\)
\(\Leftrightarrow\dfrac{-1}{x+2}\ge-\dfrac{1}{2}\)
\(\Leftrightarrow\dfrac{-2}{x+2}\ge-1\Leftrightarrow A=1-\dfrac{2}{x+2}\ge0\)
Dấu "=" xảy ra khi x = 0
\(\Rightarrow A_{min}=0\) khi x = 0