
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(x^4-1-2\left(m+1\right)x^2+2\left(m+1\right)=0\)
\(\Leftrightarrow\left(x^2-1\right)\left(x^2+1\right)-2\left(m+1\right)\left(x^2-1\right)=0\)
\(\Leftrightarrow\left(x^2-1\right)\left(x^2-2m-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2=1\\x^2=2m+1\end{matrix}\right.\)
Pt có 4 nghiệm pb khi: \(\left\{{}\begin{matrix}2m+1>0\\2m+1\ne1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m>-\dfrac{1}{2}\\m\ne0\end{matrix}\right.\)
Do \(x=\pm1< 3\) nên để \(x_1< x_2< x_3< x_4< 3\) thì:
\(\sqrt{2m+1}< 3\Leftrightarrow m< 4\) \(\Rightarrow\left\{{}\begin{matrix}-\dfrac{1}{2}< m< 4\\m\ne0\end{matrix}\right.\)
b. \(\left\{{}\begin{matrix}x_1-x_3=x_3-x_2\\x_1-x_3=x_2-x_1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=-x_2\\x_1-x_3=-x_1-x_1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=-x_1\\x_3=3x_1\end{matrix}\right.\)
Do vai trò \(x_1;x_2\) như nhau, giả sử \(x_1< 0\) \(\Rightarrow x_1;x_3\) là 2 nghiệm âm
TH1: \(\left\{{}\begin{matrix}x_1=-1\\x_2=1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_3=-\sqrt{2m+1}\\x_3=3x_1\end{matrix}\right.\) \(\Rightarrow-\sqrt{2m+1}=-3\Rightarrow m=4\)
TH2: \(x_1=-\sqrt{2m+1}\Rightarrow\left\{{}\begin{matrix}x_3=-1\\x_3=3x_1\end{matrix}\right.\) \(\Rightarrow-1=-3\sqrt{2m+1}\) \(\Rightarrow m=-\dfrac{4}{9}\)
thầy cho em hỏi nếu bài này đặt \(x^2=t^{ }\left(t\ge0\right)\)
thì giải pt ẩn t có 2 nghiệm phân biệt dương
\(=>\left\{{}\begin{matrix}\Delta>0\\S>0\\P>0\end{matrix}\right.\) em giải ra thì m>0 =)))

ĐKXĐ: \(x\ge0\)
\(\left(x^2-x-m\right)\sqrt{x}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2-x-m=0\left(1\right)\end{matrix}\right.\)
Giả sử (1) có nghiệm thì theo Viet ta có \(x_1+x_2=1>0\Rightarrow\left(1\right)\) luôn có ít nhất 1 nghiệm dương nếu có nghiệm
Do đó:
a. Để pt có 1 nghiệm \(\Leftrightarrow\left(1\right)\) vô nghiệm
\(\Leftrightarrow\Delta=1+4m< 0\Leftrightarrow m< -\dfrac{1}{4}\)
b. Để pt có 2 nghiệm pb
TH1: (1) có 1 nghiệm dương và 1 nghiệm bằng 0
\(\Leftrightarrow m=0\)
TH2: (1) có 2 nghiệm trái dấu
\(\Leftrightarrow x_1x_2=-m< 0\Leftrightarrow m>0\)
\(\Rightarrow m\ge0\)
c. Để pt có 3 nghiệm pb \(\Leftrightarrow\) (1) có 2 nghiệm dương pb
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta=1+4m>0\\x_1x_2=-m>0\\\end{matrix}\right.\) \(\Leftrightarrow-\dfrac{1}{4}< m< 0\)

\(1,\\ a,ĐK:m\ne1\\ \Delta=49+48\left(m-1\right)=48m+1\\ \text{PT vô nghiệm }\Leftrightarrow48m+1< 0\Leftrightarrow m< -\dfrac{1}{48}\\ \text{PT có nghiệm kép }\Leftrightarrow48m+1=0\Leftrightarrow m=-\dfrac{1}{48}\\ \text{PT có 2 nghiệm phân biệt }\Leftrightarrow48m+1>0\Leftrightarrow m>-\dfrac{1}{48};m\ne1\)
\(b,\Delta=4\left(m-1\right)^2+4\left(2m+1\right)=4m^2+8>0,\forall m\\ \text{Vậy PT có 2 nghiệm phân biệt với mọi m}\\ 2,\\ \text{PT có 2 nghiệm phân biệt }\)
\(\Leftrightarrow\Delta=4\left(m+1\right)^2-4\left(m^2-1\right)>0\\ \Leftrightarrow4m^2+8m+4-4m^2+4>0\\ \Leftrightarrow8m+8>0\\ \Leftrightarrow m>-1\)

Lời giải:
Nếu $m=-3$ thì PT trở thành: $7x^2-3=0$ có nghiệm $x=\pm \sqrt{\frac{3}{7}}$
-------------------------------------------------------------
Nếu $m\neq -3$Đặt $x^2=t$ thì pt trở thành:
$(m+3)t^2-(2m-1)t-3=0(*)$
1. Để pt ban đầu có 1 nghiệm thì PT $(*)$ có nghiệm $t=0$ và nếu có nghiệm còn lại thì nghiệm đó âm.
Để PT $(*)$ có nghiệm $t=0$ thì: $(m+3).0-(2m-1).0-3=0\Leftrightarrow -3=0$ (vô lý)
Do đó không tồn tại $m$ để pt có 1 nghiệm.
2. Để pt ban đầu có 2 nghiệm phân biệt thì PT $(*)$ có 1 nghiệm dương kép hoặc có 1 nghiệm dương và 1 nghiệm âm.
PT có 1 nghiệm dương, 1 nghiệm âm khi \(\left\{\begin{matrix} \Delta (*)=(2m-1)^2+12(m+3)> 0\\ P=\frac{-3}{m+3}<0\end{matrix}\right.\)
\(\Leftrightarrow m>-3\)
PT có nghiệm kép dương $\Leftrightarrow \Delta (*)=(2m-1)^2+12(m+3)=0\Leftrightarrow 4m^2+8m+37=0$ (vô lý)
Vậy $m>-3$
3.
PT ban đầu có 4 nghiệm phân biệt khi PT $(*)$ có 2 nghiệm dương phân biệt
Điều này xảy ra khi \(\left\{\begin{matrix} \Delta (*)=(2m-1)^2+12(m+3)>0\\ S=\frac{2m-1}{m+3}>0\\ P=\frac{-3}{m+3}>0\end{matrix}\right.\Leftrightarrow m< -3\)

\(x^3-x^2+2mx-2m=0\)
\(\Leftrightarrow x^2\left(x-1\right)+2m\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2+2m\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x^2=-2m\end{matrix}\right.\)
Để pt có 3 nghiệm \(\Rightarrow-2m>0\Rightarrow m< 0\)
a. Do vai trò 3 nghiệm như nhau, ko mất tính tổng quát giả sử \(x_1=1\) và \(x_2;x_3\) là nghiệm của \(x^2+2m=0\)
Để pt có 3 nghiệm pb \(\Rightarrow\left\{{}\begin{matrix}-2m>0\\-2m\ne1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}m< 0\\m\ne-\dfrac{1}{2}\end{matrix}\right.\)
Khi đó: \(x_2+x_3=0\Rightarrow x_1+x_2+x_3=1\ne10\) với mọi m
\(\Rightarrow\) Không tồn tại m thỏa mãn yêu cầu
b.
Giả sử pt có 3 nghiệm, khi đó \(\left[{}\begin{matrix}x_2=-\sqrt{-2m}< 0< 1\\x_3=\sqrt{-2m}\end{matrix}\right.\)
\(\Rightarrow\) Luôn có 1 nghiệm của pt âm \(\Rightarrow\) không tồn tại m thỏa mãn
Em coi lại đề bài

\(PT\Leftrightarrow x^2+x\left(m^2-4m+4\right)+4=0\\ \Leftrightarrow x^2+x\left(m-2\right)^2+4=0\)
PT có 2 nghiệm pb \(\Leftrightarrow\left(m-2\right)^4-16>0\Leftrightarrow\left(m-2\right)^4>16\Leftrightarrow\left[{}\begin{matrix}x< 0\\x>4\end{matrix}\right.\)


Đặt x2 + 2x + 4 = t . Điều kiện : t ≥ 3
Phương trình đã cho trở thành t2 - 2mt - 1 = 0 (1)
(1) là phương trình hoành độ giao điểm của đồ thị hàm số y = t2 - 2mt - 1 với trục Ox (tức đường thẳng y = 0). Yêu cầu bài toán thỏa mãn khi (1) có 2 nghiệm phân biệt t thỏa mãn t ≥ 3
Ta có bảng biến thiên của hàm số y = t2 - 2mt - 1
Nếu m > 3 thì yêu cầu bài toán thỏa mãn khi
8 - 6m ≥ 0 ⇔ m ≤ \(\dfrac{4}{3}\) (không thỏa mãn m > 3)
Nếu m < 3, yêu cầu bài toán thỏa mãn khi
8 - 6t ≤ 0 ⇔ m ≥ \(\dfrac{4}{3}\) Vậy m ∈ \(\)[\(\dfrac{4}{3};3\))
Nếu m = 3 thì phương trình trở thành
t2 - 6t - 1 = 0 có 2 nghiệm thỏa mãn \(\left\{{}\begin{matrix}t_1+t_2=6\\t_1.t_2=-1\end{matrix}\right.\)
tức phương trình có 2 nghiệm trái dấu (không thỏa mãn điều kiện 2 nghiệm t ≥ 3) nên m = 3 không thỏa mãn yêu cầu bài toán
Vậy tập hợp các giá trị m thỏa mãn yêu cầu bài toán là M = \(\left\{m\in R;\dfrac{4}{3}\le m< 3\right\}\)


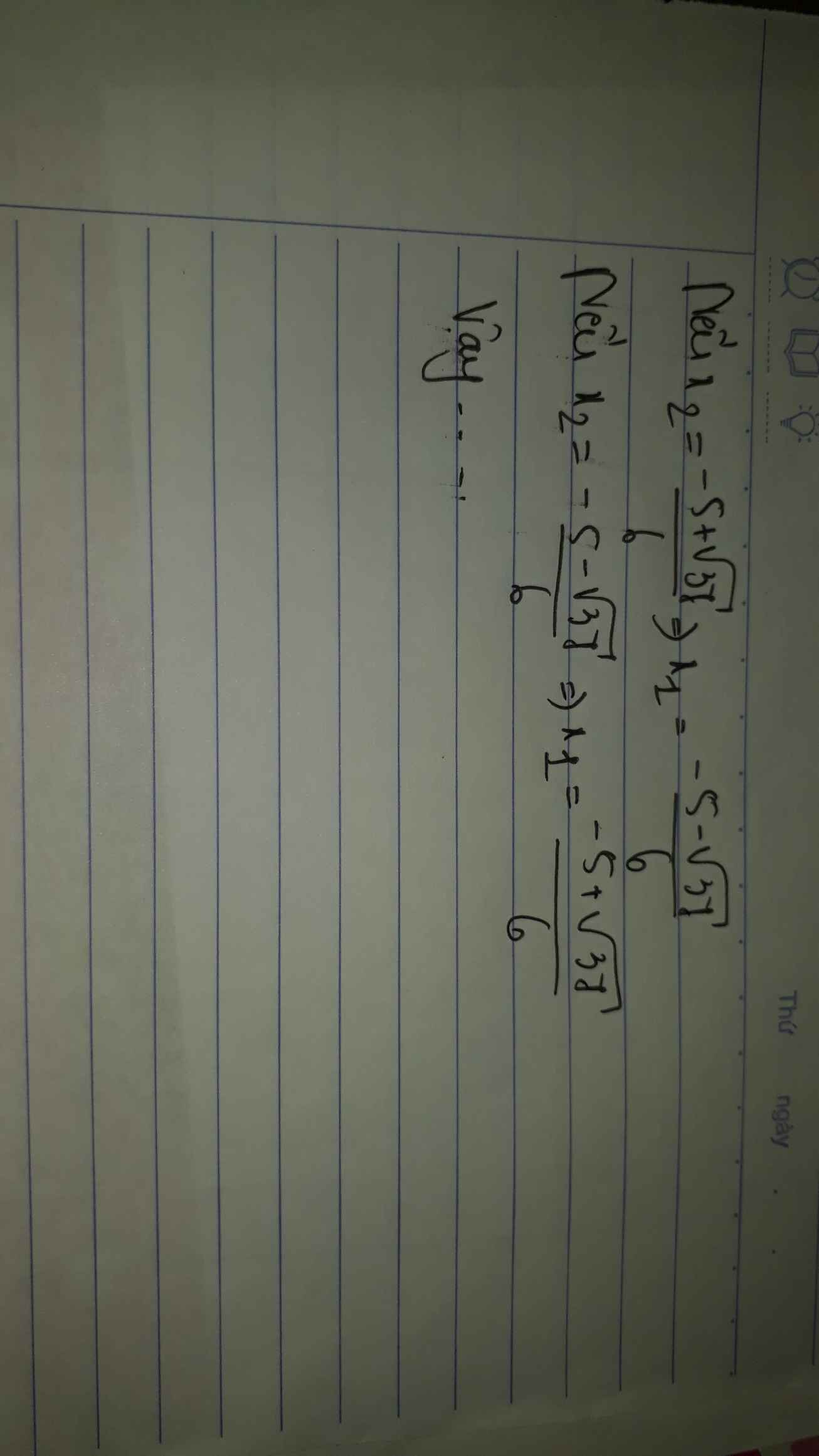
Đặt $x^2=a$. Khi đó pt có dạng :
$a^2-(2m+2)a+4=0$ (1)
Xét $\Denlta' = m^2+2m+1-4$
$ = m^2+2m-3=(m-1).(m+3)$
Để pt ban đầu có 4 nghiệm nghiệm thì pt (1) phải có 2 nghiệm phân biệt
Nên $(m-1).(m+3) > 0 $
$.....$
giải chi tiết được không