Tính : 1+2+22+23+...+250 = ?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a, A = 1 + 3 + 32 + 33 + ... + 32000
3.A = 3 + 32 + 33+ 33+... + 32001
3A - A = 3 + 32 + 33 + ... + 32001 - (1 + 3 + 32 + 33 + ... + 32000)
2A = 3 + 32 + 33 + ... + 32001 - 1 - 3 - 32 - 33 - ... - 32000
2A = 32001 - 1
A = \(\dfrac{3^{2001}-1}{2}\)

Ta có A = 2A – A = 2( 1 + 2 + 2 2 + 2 3 + . . . + 2 50 ) – ( 1 + 2 + 2 2 + 2 3 + . . . + 2 50 )
= 2 + 4 + 2 3 + 2 4 + . . . + 2 51 – ( 1 + 2 + 2 2 + 2 3 + . . . + 2 50 )
= 6 + 2 3 + 2 4 + . . . + 2 51 – ( 7 + 2 3 + . . . + 2 50 ) = 2 51 - 1
Suy ra : A + 1 = 2 51
Vậy A+1 là một lũy thừa của 2

a) \(A=1+2+2^2+...+2^{50}\)
\(\Rightarrow2A=2+2^2+...+2^{51}\)
\(\Rightarrow A=2A-A=2+2^2+...+2^{51}-1-2-2^2-...-2^{50}=2^{51}-1\)
b) \(B=1+3+3^2+...+3^{100}\)
\(\Rightarrow3B=3+3^2+...+3^{101}\)
\(\Rightarrow2B=3B-B=3+3^2+...+3^{101}-1-3-3^2-...-3^{100}=3^{101}-1\)
\(\Rightarrow B=\dfrac{3^{101}-1}{2}\)
c) \(C=5+5^2+...+5^{30}\)
\(\Rightarrow5C=5^2+5^3+...+5^{31}\)
\(\Rightarrow4C=5C-C=5^2+5^3+...+5^{31}-5-5^2-...-5^{30}=5^{31}-5\)
\(\Rightarrow C=\dfrac{5^{31}-5}{4}\)
d) \(D=2^{100}-2^{99}+2^{98}-...+2^2-2\)
\(\Rightarrow2D=2^{101}-2^{100}+2^{99}-...+2^3-2^2\)
\(\Rightarrow3D=2D+D=2^{101}-2^{100}+2^{99}-...+2^3-2^2+2^{100}-2^{99}+...+2^2-2=2^{101}-2\)
\(\Rightarrow D=\dfrac{2^{101}-2}{3}\)

a)\(...A=\dfrac{2^{50+1}-1}{2-1}=2^{51}-1\)
b) \(...\Rightarrow B=\dfrac{3^{80+1}-1}{3-1}=\dfrac{3^{81}-1}{2}\)
c) \(...\Rightarrow C+1=1+4+4^2+4^3+...+4^{49}\)
\(\Rightarrow C+1=\dfrac{4^{49+1}-1}{4-1}=\dfrac{4^{50}-1}{3}\)
\(\Rightarrow C=\dfrac{4^{50}-1}{3}-1=\dfrac{4^{50}-4}{3}=\dfrac{4\left(4^{49}-1\right)}{3}\)
Tương tự câu d,e,f bạn tự làm nhé

a) ( 72 - 8 x 9 ) : ( 20 + 21 + 22 + 23 + 24 + 25 )
= ( 72 - 72 ) : ( 20 + 21 + 22 + 23 + 24 + 25 )
= 0 : ( 20 + 21 + 22 + 23 + 24 + 25 )
= 0
b) ( 500 x 9 - 250 x 18 ) x ( 1 + 2 + 3 + ... + 9 )
= ( 500 x 9 - 250 x 2 x 9 ) x ( 1 + 2 + 3 + ... + 9 )
= ( 500 x 9 - 500 x 9 ) x ( 1 + 2 + 3 + ... + 9 )
= 0 x ( 1 + 2 + 3 + ... + 9 )
= 0
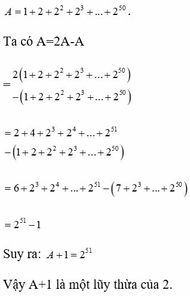
`#3107.101107`
Đặt $A = 1 + 2 + 2^2 + 2^3 + ... + 2^{50}$
$2A = 2 + 2^2 + 2^3 + ... + 2^{51}$
$2A - A = (2 + 2^2 + 2^3 + ... + 2^{51}) - (1 + 2 + 2^2 + ... + 2^{50})$
$A = 2 + 2^2 + 2^3 + ... + 2^{51] - 1 - 2 - 2^2 - ... - 2^{50}$
$A = 2^{51} - 1$
Vậy, `A =` $2^{51} - 1.$