
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




Tính [G(x) - f(x) ] = ( \(1-x^2+.....+x^{2020}\)) - (\(x^{2020}-x^{2019}+....-x+1\))
= (\(x^{2020}-x^{2019}+....-x+1\)) - (\(x^{2020}-x^{2019}+....-x+1\))
= 0
=> h(x) = [G(x) - f(x) ] * [G(x) + f(x) ]
= 0 * [G(x) + f(x) ]
= 0


\(x=2019\)\(\Rightarrow x+1=2020\)
\(\Rightarrow B=x^{2019}-\left(x+1\right).x^{2018}+........-\left(x+1\right).x^2+\left(x+1\right).x+1\)
\(=x^{2019}-x^{2019}+x^{2018}+.......-x^3-x^2+x^2+x+1\)
\(=x+1=2020\)
Vậy tại \(x=2019\)thì \(B=2020\)
Ta có x=2019
=> x + 1=2020
thay x+1 vào B, ta có:
\(A=x^{2019}-\left(x+1\right)x^{2018}+\left(x+1\right)x^{2017}-...+\left(x+1\right)x-1\)
=> \(A=x^{2019}-x^{2019}-x^{2018}+x^{2018}+x^{2017}-...+x^2+x-1\)
=> \(A=x-1=2020-1=2019\)

\(x=\frac{2019^{2020}+1}{2019^{2019}+1}>\frac{2019^{2020}+1+2018}{2019^{2019}+1+2018}=\frac{2019^{2020}+2019}{2019^{2019}+2019}=\frac{2019\left(2019^{2019}+1\right)}{2019\left(2019^{2018}+1\right)}=\frac{2019^{2019}+1}{2019^{2018}+1}\)(1)
\(y=\frac{2019^{2019}+2020}{2019^{2018}+2020}< \frac{2019^{2019}+2020-2019}{2019^{2018}+2020-2019}=\frac{2019^{2019}+1}{2019^{2018}+1}\left(2\right)\)
Từ (1) và (2) \(\Rightarrow x>y\)

(x-2020)x - 1 - (x - 2020)x + 2019 = 0
=> (x - 2020)x - 1 .[(x - 2020)2020 - 1] = 0
=> \(\orbr{\begin{cases}\left(x-2020\right)^{x-1}=0\\\left(x-2020\right)^{2020}-1=0\end{cases}\Rightarrow\orbr{\begin{cases}x-2020=0\\\left(x-2020\right)^{2020}=1^{2020}\end{cases}\Rightarrow}\orbr{\begin{cases}x-2020=0\\x-2020=\pm1\end{cases}}}\)
=> \(x-2020\in\left\{0;1;-1\right\}\Rightarrow x\in\left\{2020;2021;2019\right\}\)
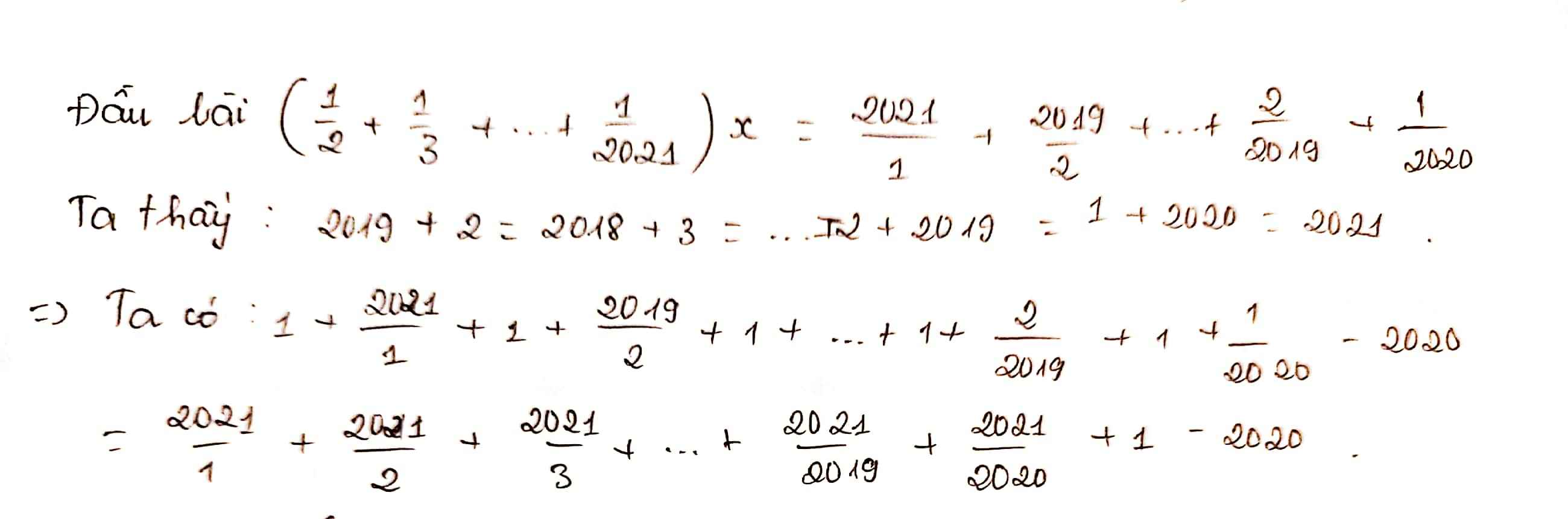
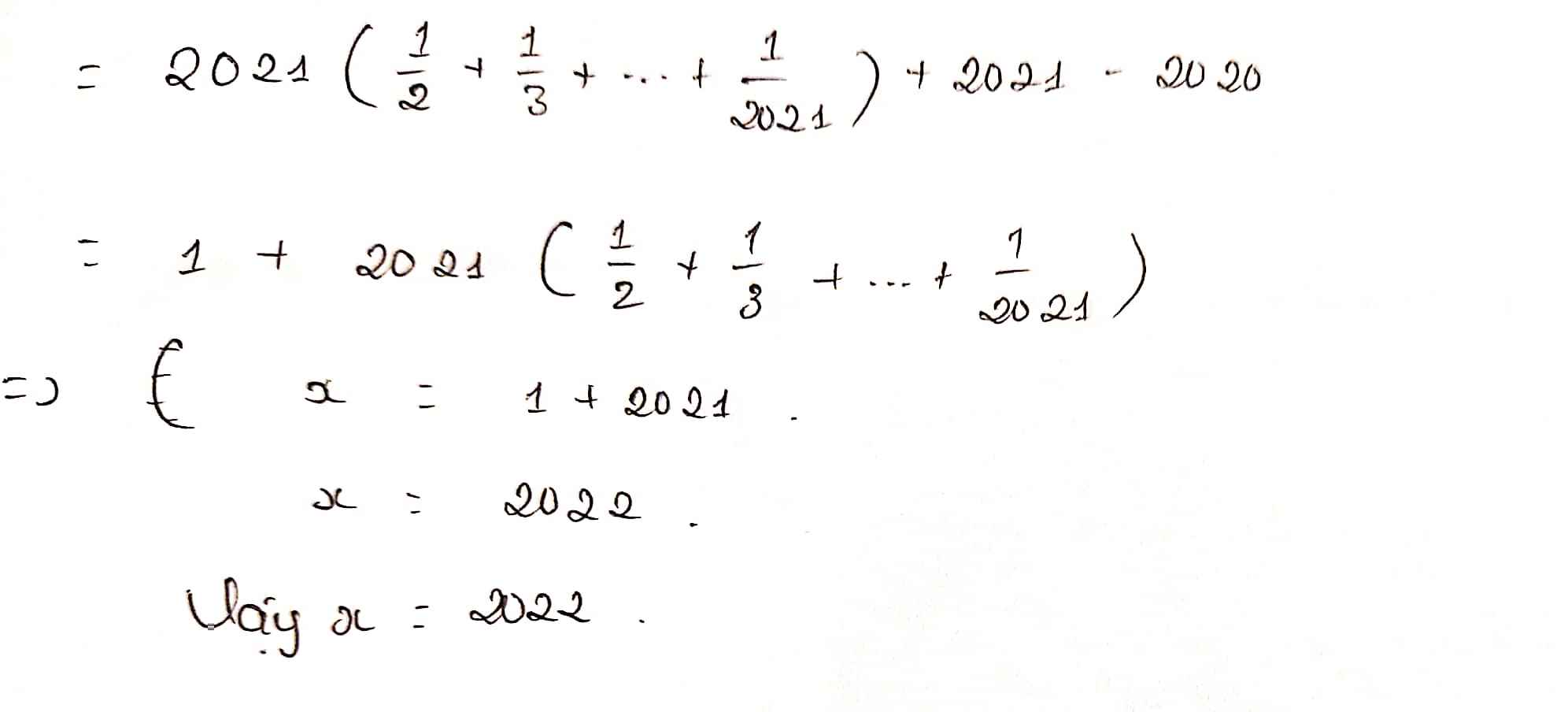
Vi x<1 nen x=0
Vay (2020-x )+(2019-x)+...+(1-x)
=2020+2019+...+1
Ta xet:1;2;...2019;2020
Day tren co so cac so la:
(2020-1):1+1=2020(so)
Tong cua day tren la:
(1+2020)*2020:2=2041210
Dap so:2041210
Neu dung thi k de ung ho minh nha ban!Thanks ban nhieu!!!